EQUIVALENT RATIOS WORD PROBLEMS

Problem 1 :

David makes 5 cups of punch by mixing 3 cups of cranberry juice with 2 cups of apple juice. How much cranberry juice and how much apple juice does David  need to make four times the original recipe ?

Solution :

Method 1 : Using table

Step 1 :

Make a table comparing the numbers of cups of cranberry juice and apple juice needed to make two times, three times, four times, and five times the original recipe.

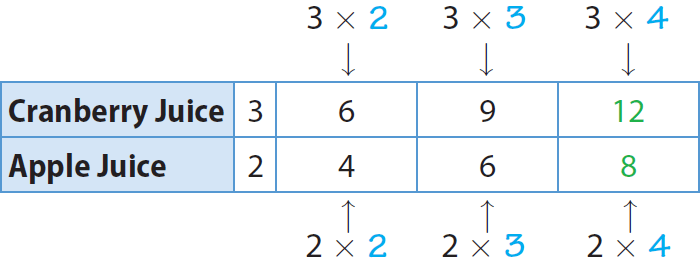
In the above table, both terms of the original ratio are multiplied by the same number to find an equivalent ratio.

Step 2 :

The last column of the table shows the numbers of cups of the two juices David needs for four times the original recipe.

David needs 12 cups of cranberry juice and 8 cups of apple juice.

Method 2 : Multiplying both terms of the ratio by the same number.

Step 1 :

Write the original ratio in fraction form.

That is,

3/2

Step 2 :

Multiply the numerator and denominator by the same number. To make four times the original recipe, multiply by 4.

That is,

To make four times the original recipe, David will need 12 cups of cranberry juice and 8 cups of apple juice.

Problem 2 :

Alex makes an alloy by mixing two types of metals, say A and B. To make one unit of alloy, he needs 12 grams of metal A and 17 grams of metal B. If he makes, 5 units  of alloy, how many grams of metal A and metal B does he need ? 

Solution :

Step 1 :

Alex needs 12 grams of metal A and 17 grams of metal B to make 1 unit of alloy.

From the above information, the ratio between metal A and metal B to make 1 unit of alloy is 

12/17

Step 2 :

Since Alex makes 5 units of alloy, we have to multiply the numerator and denominator of the above ratio by 5.

Then we have, 

(12/17) x (5/5)  =  60/85

To make 5 units of alloy, Alex needs 60 grams of metal A and 85 grams of metal B.

Problem 3 :

Are these ratios equivalent ?

$5 per 3 people

$10 per 6 people

Solution :

From the information we have, we get the following two ratios

5 : 3 and 10 : 6

To check these two ratios are equivalent, we have to apply cross product rule.

That is,

Product of extremes  =  Product of means ------ (1)

Here,

Extremes  =  5 and 6

Means  =  3 and 10

Then,

(1) -----> 5 x 6  =  3 x 10 ----->  30  =  30

We get, product of extremes is equal to product of means.

Therefore, the given two ratios are equivalent.

Problem 4 :

You are throwing a party and you need 5 liters of Yoda soda for every 12 guests. If you have 36 guests, how many liters of Yoda soda do you need ?

Solution :

The ratio between number of liters of soda and number of guests. 

5/12

If there are 36 guests, we have to make the denominator  of the above fraction as 36 using multiplication.

To make 12 as 36, we have to multiply both terms of the ratio by 3. 

Then we have, 

(5/12) x (3/3)  =  15/36

If you have 36 guests, you will need 15 liters of  Yoda soda. 

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.