EXAMPLE PROBLEMS OF FACTOR THEOREM IN DETERMINANTS

If each element of a matrix A is a polynomial in x and if |A| vanishes for x = a, then (x - a) is a factor of | A |.

(i) This theorem is very much useful when we have to obtain the value of the determinant in ‘factors’ form.

(ii) If we substitute b for a in the determinant | A |, any two of its rows or columns become identical, then | A | = 0, and hence by factor theorem (a - b) is a factor of | A |.

(iii) If r rows (columns) are identical in a determinant of order n (n ≥ r), when we put x = a, then (x - a)^{r - 1} is a factor of | A |.

(iv) A square matrix (or its determinant) is said to be in cyclic symmetric form if each row is obtained from the first row by changing the variables cyclically.

(v) If the determinant is in cyclic symmetric form and if m is the difference between the degree of the product of the factors (obtained by substitution) and the degree of the product of the leading diagonal elements and if

(1) m is zero, then the required factor is a constant k

(2) m is 1, then the required factor is k(a + b + c) and

(3) m is 2, then the required factor is k(a² + b² + c²) + l (ab + bc + ca).

Example Problems

Solve the following problems by using Factor Theorem :

(1) Show that

Solution

(2)  Show that

Solution

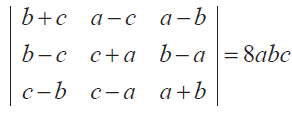
(3)  Solve

Solution

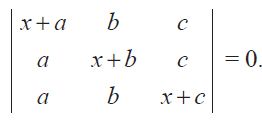
(4)  Show that

Solution

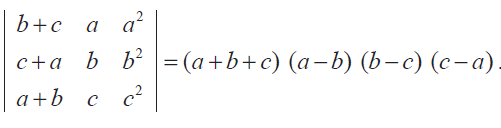
(5)  Solve

Solution

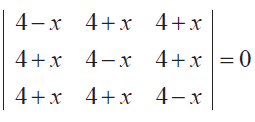
(6)  Show that

Solution

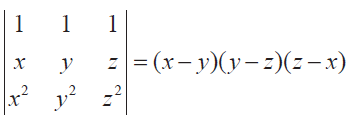
Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.