EXAMPLE PROBLEMS USING FACTOR THEOREM

Question 1 :

Solve the following problems by using Factor Theorem :

(1) Show that

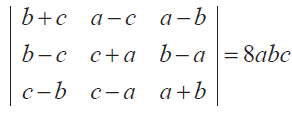
Solution :

Let us apply a = 0

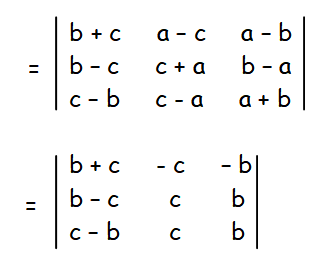
Let us factor c and b from column 2 and 3. After factor out b and c, C₂ and C₃ will be identical. So, the determinant will become 0.

Hence a is a factor. By applying the b = 0 and and c = 0, we get the same result.

So the factors are a, b and c.

Leading diagonals are (b + c), (c + a) and (a + b). The sum of the exponents of leading diagonal is 3.

m = 3 - 3  =  0

So the required factor will be constant (k).

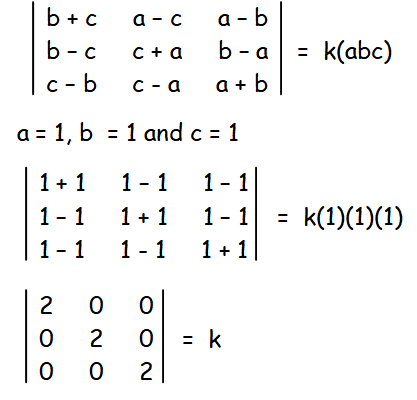
 2 [4 - 0]  =  k

 k  =  8

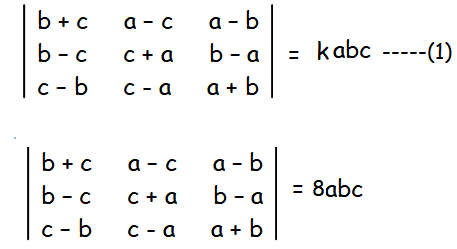
Hence proved.

Question 2 :

Solve

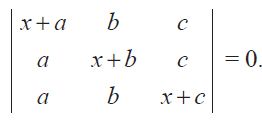
Solution :

Let the given determinant as delta. By applying the value x = 0, we get a in the first column, b in the second column and c in the third column. After factoring a, b and c from the first, second and third column respectively, we get identical rows and columns.

So (x - 0)² is a factor. Since the given matrix is in cyclic symmetric form, we may apply (x + a + b + c).

So, x + a = -b - c 

x + b  =  -a - c

and x + c  =  -a -b

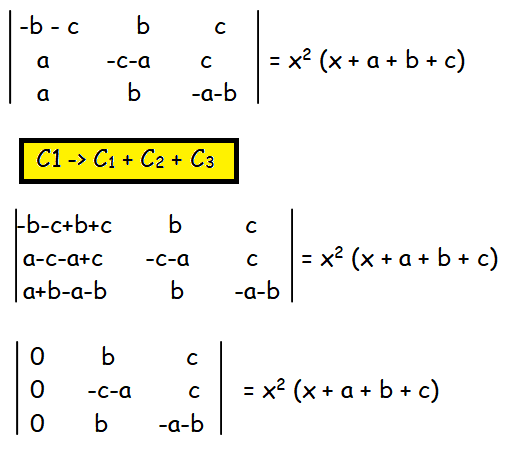
If one column in the determinant is 0, its determinant value will become 0.

x²  (x + a + b + c)  =  0

x  =  0, 0, -(a + b + c)

Question 3 :

Show that

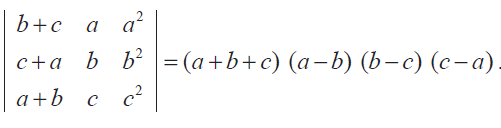
Solution :

By applying a = b, we get

First and second rows are equal. Determinant will become 0. So (a - b) is a factor.

By applying b = c, we get 2 identical rows. By applying c = a, we will get identical rows. So, (b - c) and (c - a) are factors.

So far we get 3 factors.

Sum of leading diagonals  =  4

m = 4 - 3  =  1

So, the required factor will be k(a + b + c).

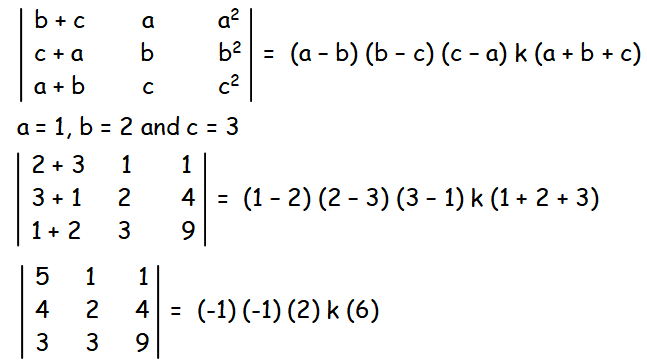
5(18 - 12) - 1(36 - 12) + 1(12 - 6)  =  12k

5(6) - 1(24) + 1(6)  =  12k

30 - 24 + 6  =  12k

12k  =  12

k = 1

By applying the value of k, we get the proof.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.