EXAMPLES ON INTERNAL AND EXTERNAL ANGLE BISECTOR THEOREM
Angle Bisector Theorem :
The internal (external) bisector of an angle of a triangle divides the opposite side internally (externally) in the ratio of the corresponding sides containing the angle.
To know more about proof, please visit the page "Angle bisector theorem proof".
Example 1 :
In a triangle MNO, MP is the external bisector of
angle M meeting NO produced at P. IF MN = 10 cm, MO = 6 cm, NO - 12 cm,
then find OP.
Solution :
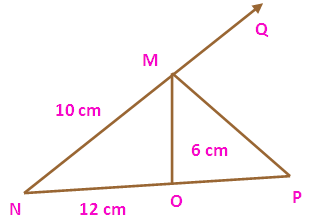
MP is the external bisector of angle M by using angle bisector theorem in the triangle MNO we get,
(NP/OP) = (MN/MO)
NP = NO + OP
= 12 + OP
(12 + OP)/OP = 10/6
6 (12 + OP) = 10 OP
72 + 6 OP = 10 OP
72 = 10 OP - 6 OP
4 OP = 72
OP = 72/4
= 18 cm
Example 2 :
In a quadrilateral ABCD, the bisectors of ∠B and ∠D intersect on AC at E. Prove that (AB/BC) = (AD/DC).
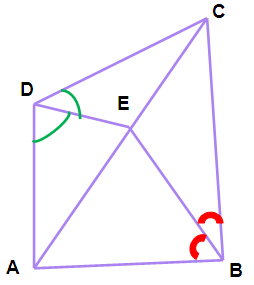
Solution :
Here DE is the internal angle bisector of angle D.
by using internal bisector theorem, we get
(AE/EC) = (AD/DC) ----- (1)
Here BE is the internal angle bisector of angle B.
(AE/EC) = (AB/BC) ----- (2)
from (1) and (2) we get,
(AB/BC) = (AD/DC)
Hence proved.
Example 3 :
The internal bisector of ∠A of triangle ABC meets BC at D and the external bisector of ∠A meets BC produced at E. Prove that (BD/BE) = (CD/CE).
Solution :
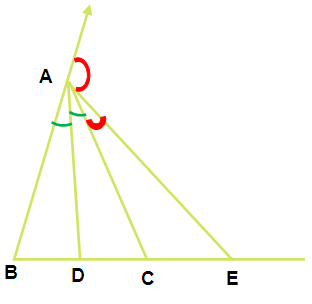
In triangle ABC, AD is the internal bisector of angle A.
by using angular bisector theorem in triangle ABC
(BD/DC) = (AB/AC) ----- (1)
In triangle ABC, AE is the internal bisector of angle A.
(BE/CE) = (AB/AC) ----- (2)
from (1) and (2) we get,
(BD/DC) = (BE/CE)
(BD/BE) = (DC/CE)
Hence proved.
Example 4 :
ABCD is a quadrilateral with AB = AD. If AE and AF are internal bisectors of ∠BAC and ∠DAC respectively,then prove that the sides EF and BD are parallel.
Solution:
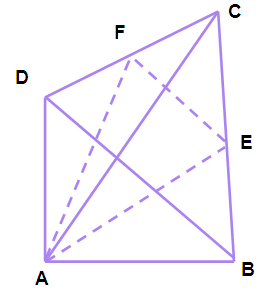
In triangle ABC, AE is the internal bisector of ∠BAC
by using bisector theorem, we get
(AB/AC) = (BE/EC) ----- (1)
In triangle ADC, AF is the internal bisector of ∠DAC
by using bisector theorem, we get
(AD/AC) = (DF/FC)
Since lengths of AD and AB are equal, we may replace AB instead of AD.
(AB/AC) = (DF/FC) ----- (2)
from (1) and (2) we get
(DF/FC) = (BE/EC)
So, EF and BD are parallel by using converse of "Thales theorem".
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 150)
Apr 25, 25 11:46 AM
Digital SAT Math Problems and Solutions (Part - 150) -
AP Calculus AB Problems with Solutions (Part - 19)
Apr 24, 25 11:10 PM
AP Calculus AB Problems with Solutions (Part - 19) -
AP Calculus AB Problems with Solutions (Part - 18)
Apr 24, 25 11:06 PM
AP Calculus AB Problems with Solutions (Part - 18)
