EXPONENTIAL FUNCTIONS AND THEIR GRAPHS

What is exponential functions ?

Let a and b be real number constants. An exponential function in x is a function that can be written in the form

f(x) = a ⋅ b^x

where a is nonzero, b is positive and b ≠ 1.

The constant a is the initial value of f (the value x = 0) and b is the base.

Let us consider the following functions,

The function f(x) = x² and g(x) = 2^x each involve a base raised to the power

Difference Between Power Function and Exponential Function

For f(x) = x², the base is the variable x, and the exponent is the constant 2. So, f(x) is a monomial and it is power function.

For g(x) = 2^x, the base is the constant , the exponent is the variable x. g is an exponential function.

Note :

Exponential functions are defined and continuous for all real numbers.

Graphs of Exponential Functions

Example 1 :

Graph each function in a viewing window [-2, 2] by [-1, 6].

(a) y₁ = 2^x(b) y₂ = 3^x

(i) Which point is common to all four graphs ?

(ii) Analyze the functions for domain, range, continuity, increasing or decreasing behavior, symmetry, extrema, asymptotes and end behaviour.

Solution :

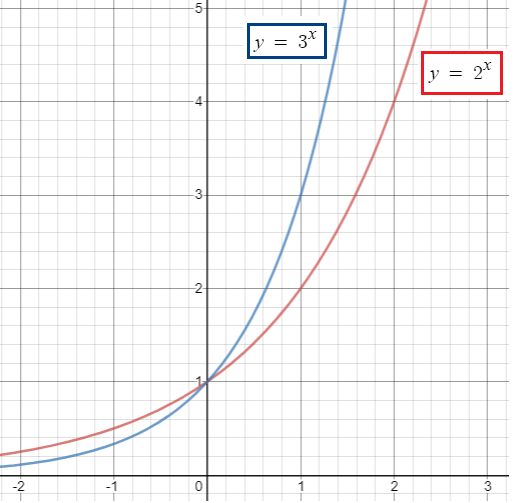
(i) Every graph is passing through the point (0, 1).

(ii) Analyzing the function :

Domain :

Domain is the defined value of x. For this function, the domain is all real numbers.

Range :

The range is y > 0.

Continuity :

Every exponential functions are defined and continuous for all real numbers.

Increasing / decreasing :

Since the base is integer, the graph is increasing.

Symmetry :

It is symmetric about none.

Asymptotes :

The graph is asymptotic to the x-axis as x approaches negative infinity

Extreama :

The graph increases without bound as x approaches positive infinity. So there is no extreama.

End behaviour :

When x approaches x to ∞, f(x) = ∞

When x approaches x to -∞, f(x) = 0

Example 2 :

Graph each function in viewing windows [-2, 2] by [-1, 6]

(a) y₁ = (1/2)^x(b) y₁ = (1/3)^x