EXPRESS THE FOLLOWING IN SIMPLEST EXPONENTIAL FORM

What is exponential form ?

The exponential form is a shortcut way of writing repeated multiplication involving base and exponents.

For example :

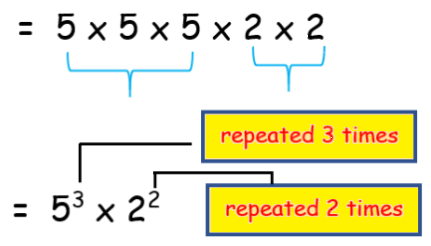
In this form, the power represents the number of times we are multiplying the base by itself.

Sometimes it may necessary to use the rules of exponents. 

Express in simplest form with a prime number base :

Problem 1 :

4

Solution :

Decompose 4 into prime factors.

4 = 2×2

2 is repeated 2 times.

= 2² 

Problem 2 :

16

Solution :

Decompose 16 into prime factors.

16 = 2×2×2×2

2 is repeated 4 times.

= 2⁴ 

Problem 3 :

27

Solution :

Decompose 27 into prime factors.

27 = 3×3×3

3 is repeated 3 times.

= 3³ 

Problem 4 :

4²

Solution :

4² = (2×2)²

2 is repeated 2 times.

= (2²)²

By using power rule (a^m)ⁿ = a^mn, we get

= 2⁴  

Problem 5 :

25²

Solution :

25² = (5×5)²

5 is repeated 2 times.

= (5²)²

By using power rule (a^m)ⁿ = a^mn, we get

= 5⁴ 

Problem 6 :

2^t × 8

Solution :

Decompose 8 into prime factors.

8 = 2×2×2

= 2^t × 8

= 2^t×2×2×2

2 is repeated 3 times.

= 2^t × 2³

By using product rule a^m × aⁿ = a^m+n, we get

= 2^{t + 3}

Problem 7 :

3^a ÷ 3

Solution :

= 3^a ÷ 3

= 3^a ÷ 3¹

By using quotient rule a^m ÷ aⁿ = a^m-n, we get

= 3^{a – 1} 

Problem 8 :

2ⁿ × 4ⁿ

Solution :

= 2ⁿ × 4ⁿ

= 2ⁿ×(2×2)ⁿ

= 2ⁿ × (2²)ⁿ

= (2)ⁿ × (2)²ⁿ

By using product rule a^m × aⁿ = a^m+n, we get

= 2^{n+ 2n}

= 2³ⁿ 

Problem 9 :

9/(3^x)

Solution :

= 9/(3^x)

= (3²)/(3^x)

By using quotient rule a^m/aⁿ = a^m-n, we get

= 3^{2 – x}

Problem 10 :

5ⁿ⁺²/5^n–2

Solution :

= 5ⁿ⁺²/5^n–2

By using quotient rule a^m/aⁿ = a^m-n, we get

= 5^{(n+2)–(n–2)}

= 5^n+2-n+2

= 5²⁺²

= 5⁴

Problem 11 :

(3⁴)^a+1

Solution :

= (3⁴)^a+1

By using power rule (a^m)ⁿ = a^mn, we get

= 3^{(4a + 4)} 

Problem 12 :

3^x × 3^4-x

Solution :

= 3^x × 3^4–x

By using product rule a^m × aⁿ = a^m+n, we get

= (3)^x+4–x

= 3⁴ 

Problem 13 :

(4^a)/2^b

Solution :

= (4^a)/2^b

= (2 × 2)^a/2^b

= (2²)^a/2^b

= 2^2a/2^b

By using quotient rule a^m/aⁿ = a^m-n, we get

= 2^{(2a – b)} 

Problem 14 :

8^x/16^y

Solution :

8^x/16^y = (2 × 2 × 2)^x/(2 × 2 × 2 × 2)^y

= (2³)^x/(2⁴)^y

 = 2^3x/2^4y

By using quotient rule a^m/aⁿ = a^m-n, we get

= 2^{3x – 4y} 

Problem 15 :

5^1+x /5^x-1

Solution :

= 5^1+x/5^x–1 

By using quotient rule a^m/aⁿ = a^m-n, we get

= 5^1+x-x+1

= 5²

Problem 16 :

(3^a × 9^a)/27^a+2

Solution :

= (3^a × (3²)^a)/(3³) ^{a + 2}

= (3^a × 3^2a)/3^3a+6

= 3^a+2a/3^3a+6

= 3^a+2a-3a-6

= 3^a-a-6

= 3^-6

= 1/3⁶

Apart from the stuff given above,  if you need any other stuff in math, please use our google custom search here.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.