FACTOR THEOREM EXAMPLES AND SOLUTIONS

Question 1 :

Solve the following problems by using Factor Theorem :

(1) Solve

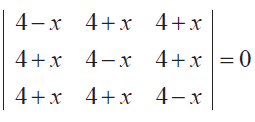
Solution :

By applying x = 0, we get identical rows and columns.

Hence the determinant will become 0.

So, x² is a factor.

By adding row 1, row 2 and row 3, we get

4 - x + 4 + x + 4 + x  =  0

12 + x = 0

x  =  -12

Hence -12 is the value which make the determinant zero. So the answers are 0, 0 and -12.

Question 2 :

Show that

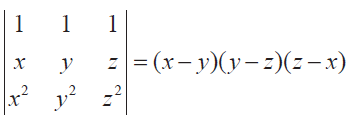
Solution :

let us apply, x = y

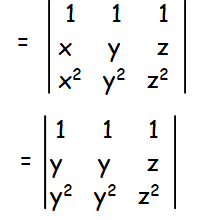
Column 1 and 2 are identical. So the determinant will become zero.

Hence (x - y) is a factor. In the same way, we may show that (y - z) and (z - x) are factors.

Sum of exponents of leading diagonal  =  3

A number of factors that we get so far  =  3

Hence the required factor is a constant (k).

1(18 - 12) - 1(9 - 3) + 1(4 - 2)  =  k(-1)(-1)(2)

6 - 6 + 2  =  2k

k  =  1

By applying the value of k, we get the given proof.

Question 3 :

In a triangle ABC, if

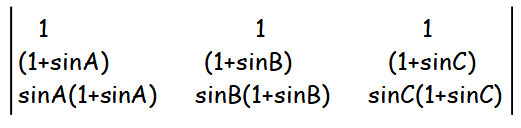
prove that triangle ABC is an isosceles triangle.

Solution :

By putting sin A = sin B, we get

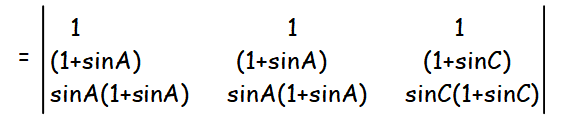
That is, by putting sin A = sin B we see that, the given equation is satisfied.

Similarly by putting sin B = sin C and sin C = sin A, the given equation is satisfied.

Thus, we have A = B or B = C or C = A.

In all cases atleast two angles are equal. Thus the triangle is isosceles.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.