FACTORING QUADRATIC POLYNOMIALS WORKSHEET

Factor each quadratic polynomial. 

Problem 1 :

x² + 6x + 5

Problem 2 : 

x² + 2x - 35

Problem 3 : 

x² - 6x - 7

Problem 4 : 

x² - 18x + 65

Problem 5 :

3x² – 5x – 12

Problem 6 :

2x² + x - 6

Problem 7 :

The height of the rock thrown off a cliff can be modeled by the equation h = -16t² - 8t + 120, where h is the height in feet and t is the time in seconds. How long does it take the rock to reach the ground ?

Problem 8 :

The product of two consecutive negative integers is 1122. What are the numbers?

Detailed Answer Key

1. Answer :

x² + 6x + 5

In the quadratic polynomial above, the coefficient of x² is 1.

Decompose the constant term +5 into two factors such that the product of the two factors is equal to +5 and the addition of two factors is equal to the coefficient of x, that is +6. 

Then, the two factors of +5 are 

+1 and +5

Factor the given quadratic polynomial using +1 and +5. 

x² + 6x + 5  =  (x + 1)(x + 5)

The factors of the given quadratic polynomial are

(x + 1) and (x + 5)

2. Answer :

x² + 2x - 35

In the quadratic polynomial above, the coefficient of x² is 1.

Decompose the constant term -35 into two factors such that the product of the two factors is equal to -35 and the addition of two factors is equal to the coefficient of x, that is +2. 

Then, the two factors of -35 are 

-5 and +7

Factor the given quadratic polynomial using -5 and +7. 

x² + 2x - 35  =  (x - 5)(x + 7)

The factors of the given quadratic polynomial are

(x - 5) and (x + 7)

3. Answer :

x² - 6x - 7

In the quadratic polynomial above, the coefficient of x² is 1.

Decompose the constant term -7 into two factors such that the product of the two factors is equal to -7 and the addition of two factors is equal to the coefficient of x, that is -6. 

Then, the two factors of -7 are 

-7 and +1

Factor the given quadratic polynomial using -7 and +1. 

x² - 6x - 7  =  (x - 7)(x + 1)

The factors of the given quadratic polynomial are

(x - 7) and (x + 1)

4. Answer :

x² - 18x + 65

In the quadratic expression polynomial, the coefficient of x² is 1.

Decompose the constant term +65 into two factors such that the product of the two factors is equal to +65 and the addition of two factors is equal to the coefficient of x, that is -18. 

Then, the two factors of +65 are 

-5 and -13

Factor the given quadratic polynomial using -5 and -13. 

x² - 18x + 65  =  (x - 5)(x - 13)

Therefore, the factors of the given quadratic polynomial are

(x - 5) and (x - 13)

5. Answer :

3x² – 5x – 12

In the given quadratic polynomial, the coefficient of x² is not 1.

So, multiply the coefficient of x² and the constant term "-12". 

3 ⋅ (-12)  =  -36

Decompose -36 into two factors such that the product of two factors is equal to -36 and the addition of two factors is equal to the coefficient of x, that is -5.

Then, the two factors of -36 are 

+4 and -9

Now we have to divide the two factors 4 and -3 by the coefficient of x², that is 3.

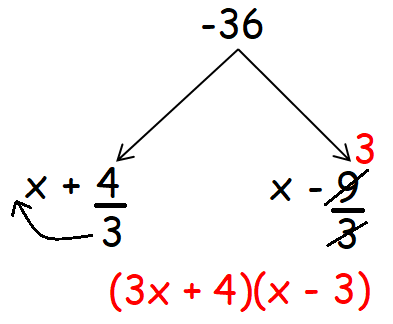
3x² – 5x – 12  =  (3x + 4)(x - 3)

The factors of the given quadratic expression are

(3x + 4) and (x - 3)

6. Answer : 

2x² + x - 6

In the given quadratic polynomial, the coefficient of x² is not 1.

Multiply the coefficient of x² and the constant term "-6". 

That is, 

2 ⋅ (-6)  =  -12

Decompose -12 into two factors such that the product of two factors is equal to -12 and the addition of two factors is equal to the coefficient of x, that is 1.

Then, the two factors of -12 are 

4 and -3

Now we have to divide the two factors 4 and -3 by the coefficient of x², that is 2.fdcatoringqno5p5.png

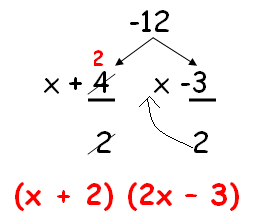
2x² + x - 6  =  (x + 2)(2x - 3)

The factors of the given quadratic expression are

(x + 2) and (2x - 3)

7. Answer :

h = -16t² - 8t + 120

-16t² - 8t + 120 = 0

Dividing the equation by 8, we get

-2t² - t + 15 = 0

-2t² + 6t - 5t + 15 = 0

-2t (t - 3) -5(t - 3) = 0

(-2t - 5)(t - 3) = 0

Equating each factor to 0, we get

So, the solutions are 3 and -5/2.

8. Solution :

n = 1^st negative integer

n + 1 = 2^nd consecutive integer

n(n + 1) = 1122

n² + n = 1122

n² + n – 1122 = 0

(n + 34)(n – 33) = 0

n = -34, 33 n + 1 = -33, 34

So, the two negative numbers are -33 and -34.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.