FIND ABSOLUTE EXTREMA OF FUNCTION ON CLOSED INTERVAL

The absolute maxima and absolute minima are referred to describing the largest and smallest values of a function on an interval.

Let x₀ be a number in the domain D of a function f(x) . Then f (x₀) is the absolute maximum value of f (x) on D,

if f(x₀) ≥ f(x) for all x ∈ D and f(x₀) is the absolute minimum value of f(x) on D if f(x₀) ≤ f(x) 

Find the absolute extrema of the following functions on the given closed interval.

Problem 1 :

f (x)  =  x³ − 12x + 10 ; [1, 2]

Solution :

f (x)  =  x³ − 12x + 10

f'(x)  =  3x² - 12(1)

f'(x)  =  3x² - 12

f'(x)  =  0

 3x² - 12  =  0

x²  =  4

x  =  ±2

x = 2 ∈ [1, 2]

f(1)  =  1³ − 12(1) + 10  ==>  -1

f(2)  =  2³ − 12(2) + 10

=  8 - 24 + 10

=  -6

Absolute maximum is -1 and absolute minimum is -6.

Problem 2 :

f(x)  =  3x⁴ - 4x³ ; [-1, 2]

Solution :

f(x)  =  3x⁴ - 4x³

f'(x)  =  12x³ - 12x²

f'(x)  =  0

12x³ - 12x²  =  0

12x²(x-1)  =  0

x  =  0 and x  =  1

f(x)  =  3x⁴-4x³

f(-1)  =  3(-1)⁴-4(-1)³  =  7

f(0)  =  3(0)⁴-4(0)³  =  0

f(1)  =  3(1)⁴-4(1)³  =  -1

f(2)  =  3(2)⁴-4(2)³  =  16

So, absolute maximum is 16 and absolute minimum is -1.

Problem 3 :

f(x)  =  6x^4/3 - 3x^1/3 ;  [-1, 1]

Solution :

f(x)  =  6x^4/3 - 3x^1/3

f'(x)  =  6 (4/3) x^1/3  - 3(1/3)x^-2/3

f'(x)  =  8x^1/3  - x^-2/3

f'(x)  =  0

8x^1/3  - x^-2/3  =  0

8x^1/3  =  x^-2/3 

x^1/3  x^2/3  =  1/8

x  =  1/8

f''(x)  =  (8/3)x^(1/3)-1  + (2/3) x^{(-2/3) - 1}

f''(x) =  (8/3)x^(-2/3)  + (2/3) x^(-5/3)

f''(x) =  (8/3)(1/8)^(-2/3)  + (2/3) (1/8)^(-5/3)

=  (8/3)(1/2)^-2  + (2/3) (1/2)^-5

=  (8/3)(4)  + (2/3) (32)

= 32/3 + 64/3

= 96/3

= 32 > 0 

So, relative minimum is at x  1/8.

Problem 4 :

f(x)  =  2 cos x + sin 2x ; [0, π/2]

Solution :

f(x)  =  2 cos x + sin 2x

f'(x)  =  -2 sinx + 2 cos 2x

f'(x)  =  0

-2 sinx + 2 cos 2x  =  0

-2 sinx + 2 (1-2sin²x)  =  0

-2 sinx + 2 - 4sin²x  =  0

4 sin²x+2sinx-2  =  0

Let t  =  sinx

4t²+2t-2  =  0

2t²+t-1  =  0

(2t-1) (t+1)  =  0

t  =  1/2 and t  =  -1

sin x  =  1/2 and sin x  =  -1

f(x)  =  2 cos x + sin 2x

f(π/2)  =  2 cos π/2 + sin 2(π/2)

f(π/2)  =  0

f(0)  =  2 cos 0 + sin 2(0)

f(0)  =  2

f(π/6)  =  2 cos π/6 + sin 2(π/6)

f(π/2)  =  1+√3/2

So, absolute maximum is 2 and absolute minimum is 0.

Problem 5 :

A function f is continuous on the closed interval [-1, 3] and its first and second derivatives have the following properties.

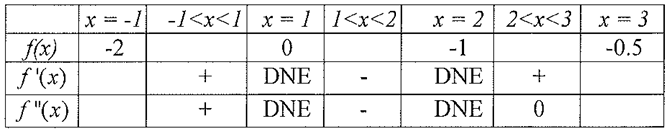
a) Find the x-coordinate of all local extrema of f(x) and indicate which are maxima and which are minima. Justify.

b) Find the x-coordinates of absolute extrema on f on [-1, 3].

c) Find all intervals on which the graph f' is concave up or concave down 

Solution :

a) By observing the table, in the interval -1 < x < 1 the curve is increasing since it has the positive slope and in the interval 1 < x < 2 the curve is decreasing. So, 

at x = 1, the function f(x) has relative maximum 

By observing the table, in the interval -1 < x < 2 the curve is decreasing since it has the negative slope and in the interval 2 < x < 3 the curve is increasing since it has positive slope. So, 

at x = 2, the function f(x) has relative minimum

b)  f(-1) = -2, f(1) = 0, f(2) = -1, f(3) = -0.5

• At x = 1, absolute maximum since f(1) > f(3)
• At x = -1, absolute minimum since f(-1) < f(2)

c)

• f is concave up on (-1, 1) since f''(x) > 0
• f is concave down on (1, 2) since f''(x) < 0

Problem 6 :

The function f(x) = x⁴ - 4x² has 

a) One relative minimum and two relative maxima

b)  One relative minimum and one relative maximum

c) Two relative maxima and no relative minimum

d)  Two relative minima and no relative maximum

e) Two relative minima and one relative maximum

Solution :

f(x) = x⁴ - 4x²

f'(x) = 4x³ - 4(2x)

= 4x³ - 8x

f'(x) = 0

4x(x² - 2) = 0

x = 0, x = -√2 and √2

f''(x) = 4(3x²) - 8(1)

= 12x² - 8

f''(0) = 12(0)² - 8

= -8 < 0 maxima

f''(-√2) = 12(-√2)² - 8

= 24 - 8

= 16 > 0 minima

f''(√2) = 12(√2)² - 8

= 24 - 8

= 16 > 0 minima

Two relative minima and one relative maxima. So, option e is correct.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.