FIND DERIVATIVES OF RADICAL FUNCTIONS

To find the derivative of a radical function, first write the radical sign as exponent and find derivative using chain rule. 

Example :

f(x) = √x

f(x) = x^1/2

Find derivative with respect to x.

f'(x) = (1/2)x^1/2-1(x')

f'(x) = (1/2)x^-1/2(1)

= (1/2)(1/x^1/2)

= (1/2)(1/√x)

= 1/(2√x)

Base on the above example, we can derive formula for derivative of a radical function.

Let f(x) = √z. Then derivative f(x) : 

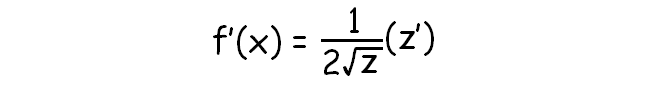
Find the derivative of the following radical functions with respect to x :

Example 1 :

y = √(x + 2)

Solution :

y = √(x + 2)

y' = {1/[2√(x + 2)]}(x + 2)'

y' = {1/[2√(x + 2)]}(1)

y' = 1/[2√(x + 2)]

Example 2 :

y = √(2x - 1)

Solution :

y = √(2x - 2)

y' = {1/[2√(2x - 1)]}(2x - 1)'

y' = {1/[2√(2x - 1)]}(2)

y' = 1/√(2x - 1)

Example 3 :

y = √(3x² + 5)

Solution :

y = √(3x² + 5)

y' = {1/[2√(3x² + 5)]}(3x² + 5)'

y' = {1/[2√(3x² + 5)]}(6x)

= 3x/√(3x² + 5)

Example 4 :

y = √(2x⁴ + 2x - 1)

Solution :

y = √(2x⁴ + 2x - 1)

y' = {1/[2√(2x⁴ + 2x - 1)]}(2x⁴ + 2x - 1)'

y' = {1/[2√(2x⁴ + 2x - 1)]}(8x³ + 2)

= (4x³ + 1)/(√2x⁴ + 2x - 1)

Example 5 :

y = (x³ + 2x)√x

Solution :

y = (x³ + 2x)√x

Since two x terms are multiplied, we have to use the product rule to find the derivative.

Product rule :

(uv)' = uv' + u'v

y' = (x³ + 2x)(1/2√x) + (3x² + 2)√x

= (x³/2√x + 2x/2√x) + 3x²√x + 2√x

= (1/2)x^(3-1/2) + x^{(1 - 1/2)} + 3x^{(2 + 1/2)} + 2√x

= (1/2)x^5/2 + x^1/2 + 3x^5/2 + 2√x

= [(1/2) + 3]x^5/2 + √x + 2√x

  = (7/2)x^5/2 + 3√x

Example 6 :

y = (√x + 2x)/x² - 1

Solution :

y = (√x + 2x)/x² - 1

In the above function, we have variable x in both numerator and denominator.

So, we have to use the quotient rule to find the derivative 

Quotient rule :

(u/v)' = (vu' - uv')/v²

= [(x² - 1)(1/2√x + 2) - (√x + 2x) (2x)]/(x² - 1)²

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.