COMPLEMENTARY SUPPLEMENTARY VERTICAL ADJACENT AND CONGRUENT ANGLES
Complementary Angles
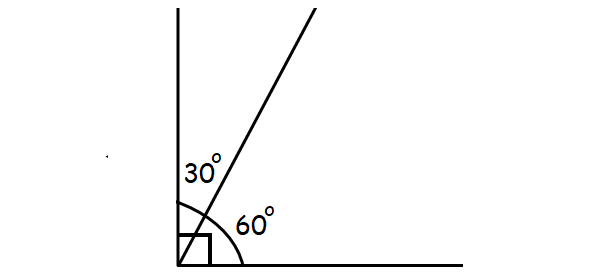
Two angles are said to be complementary to each other if sum of their measures is 90°.
For example, if ∠A = 52° and ∠B = 38°, then angles ∠A and ∠B are complementary to each other.
Supplementary Angles
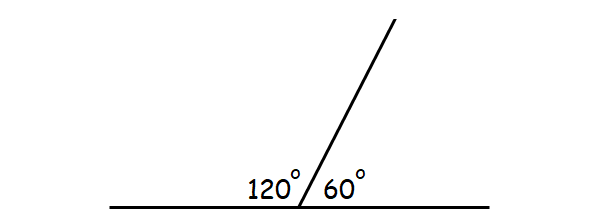
Two angles are said to be supplementary to each other if sum of their measures is 180°.
For example, the angles whose measures are 112° and 68° are supplementary to each other.
Vertical Angles
The angles opposite each other when two lines cross. They are always equal.
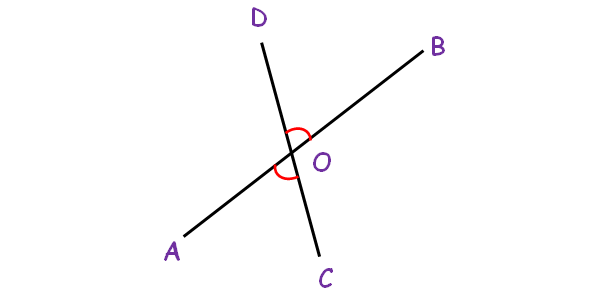
∠DOB = ∠AOC
∠DOA = ∠COB
Adjacent Angles
Two angles are adjacent when they have common side and common vertex and do not overlap.

Angle AOC is adjacent to angle BOC
Because,
- they have a common side (line OB)
- they have a common vertex (point O)
Congruent Angles
Congruent Angles have the same angle
- They don't have to point in the same direction.
- They don't have to be on similar sized lines.
- Just the same angle.

∠ABC = ∠DOE
Solved Problems
Problem 1 :
Find the value of x in the diagram shown below.
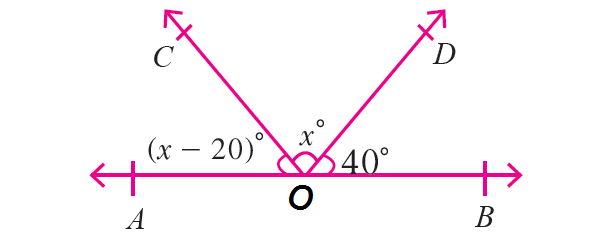
Solution :
Because all the three angle measures in the above diagram are on the same straight line AOB, they are supplementary.
(x - 20)° + x° + 40° = 180°
x - 20 + x + 40 = 180
2x + 20 = 180
Subtract 20 from each side.
2x = 160
Divide each side by 2.
x = 80
Problem 2 :
In the diagram shown below, if the lines AB and CD are parallel and EF is transversal, find the value of x.

In the diagram shown above, because the lines AB and CD are parallel and EF is transversal, ∠FOB and ∠OHD are corresponding angles and they are congruent.
∠FOB = ∠OHD
(2x + 20)° = (3x - 10)°
2x + 20 = 3x - 10
Subtract 2x from each side.
20 = x - 10
Add 10 to each side.
30 = x
Problem 3 :
In the diagram shown below, if the lines AB and CD are parallel and EF is transversal, find the value of x.
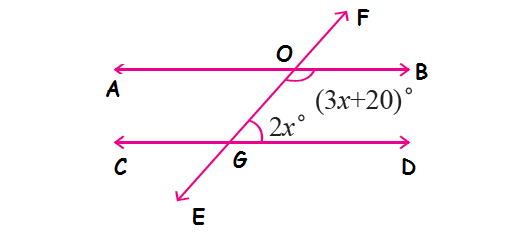
In the diagram shown above, because the lines AB and CD are parallel and EF is transversal, ∠BOG and ∠OGD are consecutive interior angles and they are supplementary.
∠BOG + ∠OGD = 180°
(3x + 20)° + 2x° = 180°
3x + 20 + 2x = 180
5x + 20 = 180
Subtract 20 from each side.
5x = 160
Divide each side by 5.
x = 32
Problem 4 :
Find the value of 'x' in the diagram shown below.
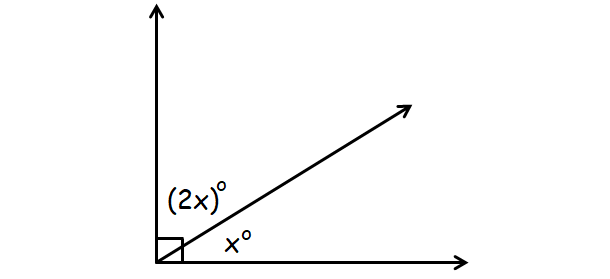
In the diagram shown below, it clear that the angle measures x° and (2x)° are complementary.
(2x)° + x° = 90°
2x + x = 90
3x = 90
Divide each side by 3.
x = 30
Problem 5 :
Find the value of x in the diagram shown below.
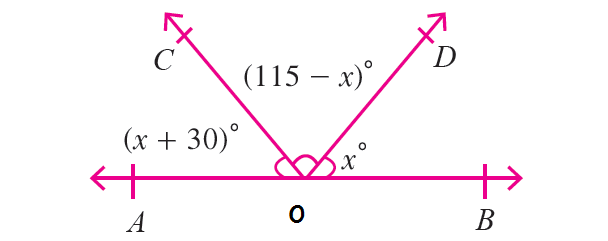
Solution :
Because all the three angle measures in the above diagram are on the same straight line AOB, they are supplementary.
(x + 30)° + (115 - x)° + x° = 180°
x + 30 + 115 - x + x = 180
x + 145 = 180
Subtract 145 from each side.
x = 35
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 152)
Apr 28, 25 11:54 AM
Digital SAT Math Problems and Solutions (Part - 152) -
Digital SAT Math Problems and Solutions (Part - 151)
Apr 26, 25 11:18 AM
Digital SAT Math Problems and Solutions (Part - 151) -
AP Calculus BC Problems with Solutions
Apr 26, 25 05:49 AM
AP Calculus BC Problems with Solutions
