FIND ORTHOCENTER OF A TRIANGLE WITH GIVEN VERTICES

What is Orthocenter ?

It can be shown that the altitudes of a triangle are concurrent and the point of concurrence is called the orthocentre of the triangle.The orthocentre is denoted by O.

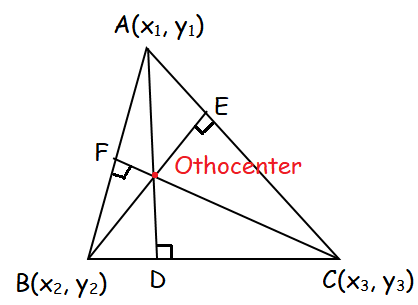
Let ABC be the triangle AD,BE and CF are three altitudes from A, B and C to BC, CA and AB respectively.

Steps Involved in Finding Orthocenter of a Triangle :

• Find the equations of two line segments forming sides of the triangle.
• Find the slopes of the altitudes for those two sides.
• Use the slopes and the opposite vertices to find the equations of the two altitudes.
• Solve the corresponding x and y values, giving you the coordinates of the orthocenter.

Example 1 :

Find the co ordinates of the orthocentre of a triangle whose vertices are (3, 4) (2, -1) and (4, -6).

Solution :

Let the given points be A (3, 4) B (2, -1) and C (4, -6)

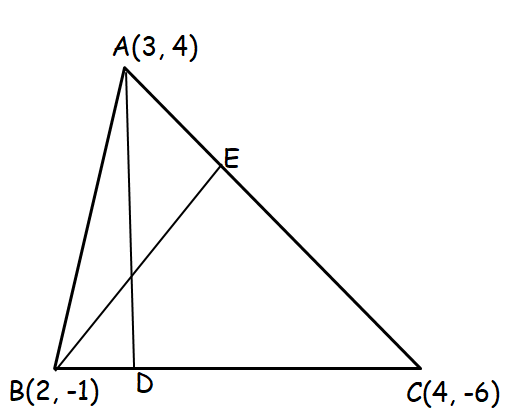
Slope of AC  =  [(y₂ - y₁)/(x₂ - x₁)]

A (3, 4) and C (4, -6)

here x₁  =  3, x₂  =  4, y₁  =  4 and y₂  =  -6

  =  [(-6-4)/(4-3)]

  =  (-10)/1

  = -10

Slope of the altitude BE  =  -1/ slope of AC

  =  -1/(-10)

  =  1/10

Equation of the altitude BE :

(y - y₁)  =  m (x -x₁)

Here B (2, -1) and m  =  1/10

 (y - (-1))  =  1/10 (x - 2)

10 (y + 1)  =  (x - 2)

10y + 10  =  x - 2

x - 10y - 2 - 10  =  0  

x - 10y - 12  =  0

x - 10y - 12  =  0

x  =  10y + 12  --------(1)

Slope of BC  =  (y₂ - y₁)(x₂ - x₁)]

B (2,-1) and C (4,-6)

here x₁ = 2,x₂ = 4,y₁ = -1 and y₂ = -6

  =  [(-6 - (-1))/(4 - 2)]

  =  (-6 + 1)/2

   =  -5/2

Slope of the altitude AD  =  -1/ slope of AC

=  -1/(-5/2)

=  2/5

Equation of the altitude AD :

(y - y₁)  =  m (x - x₁)

Here A(3, 4)  m  =  2/5

(y - 4)  =  2/5 (x - 3)

5(y - 4)  =  2(x - 3)

5y - 20  =  2x - 6

2x - 5y - 6 + 20  =  0

2x - 5y + 14  =  0 --------(2)

Substituting (1) into (2), we get

2(10y + 12) - 5y + 14  =  0

20y + 24 - 5y + 14  =  0

15y + 38  =  0

15y  =  -38

y  =  -38/15

By applying y  =  -38/15 in (1), we get

x  =  10(-38/15) + 12

x  =  -76/3  +  12

x  =  -40/3

Therefore the orthocenter is (-40/3, -38/15).

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.