FINDING AREA AND PERIMETER INVOLVING POLYNOMIALS

Example 1 :

Find, in simplest form, an expression for the perimeter P of:

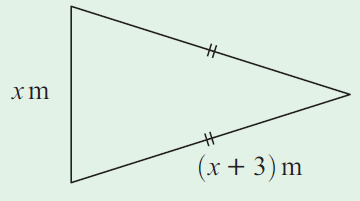
Solution :

The given triangle is a isosceles triangle. Side lengths of the given triangle are x + 3, x + 3 and x. 

Perimeter of the triangle given = x + 3 + x + 3 + x

= 3x + 6

So, perimeter of the triangle is 3x + 6.

Example 2 :

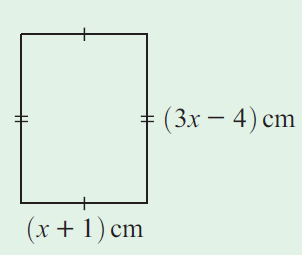
Solution :

The given figure is a rectangle.

Perimeter of rectangle = 2(length + width)

Length = 3x - 4 and width = x + 1

= 2(3x - 4 + x + 1)

= 2(4x - 3)

= 8x - 6

So, the required perimeter is 8x - 6.

Example 3 :

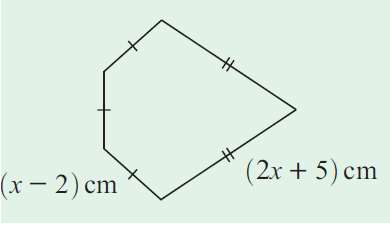
Solution :

Perimeter of the shape = 2(2x + 5) + 3(x - 2)

= 4x + 10 + 3x - 6

= 7x + 4

Example 4 :

Find, in simplest form, an expression for the area A of :

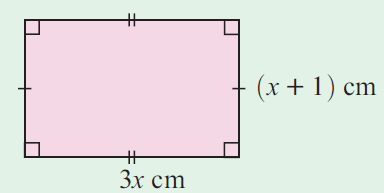
Solution :

The given shape is rectangle.

Area of rectangle = length x width

length = x + 1 and width = 3x

= (x + 1) ⋅ 3x

= 3x² + 3x

Example 5 :

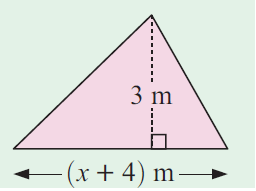
Solution :

Area of triangle = (1/2) ⋅ base ⋅ height

= 1/2 ⋅ (x + 4) ⋅ 3

= (3/2)(x + 4)

Example 6 :

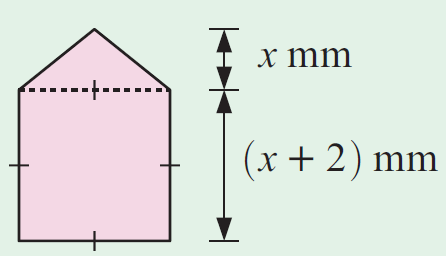
Solution :

Area of given shape = Area of square + Area of triangle

Side length of square = x + 2, base of triangle = x + 2 and height of the triangle = x.

Area of square = (x + 2)(x + 2)

= x² + 4x + 4

Area of triangle = (1/2) ⋅ base ⋅ height

= (1/2) ⋅ (x + 2) ⋅ x

= x(x + 2)/2

Area of the shape = (x² + 4x + 4) + x(x + 2)/2

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.