FINDING CENTER FOCI VERTICES AND DIRECTRIX OF ELLIPSE AND HYPERBOLA

Ellipse - Symmetric About x-Axis

where a² > b² and major axis is along x-axis.

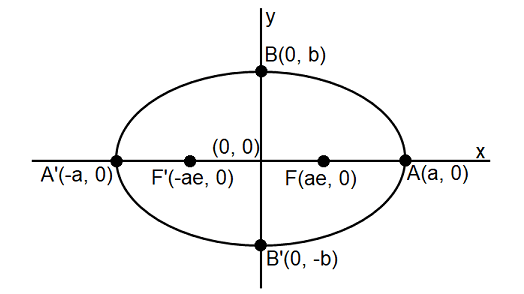
Center : (0, 0).

Foci : F(ae, 0) and F'(-ae, 0)

Vertices : A(a, 0) and A'(-a, 0).

Equations of directrices : x = a/e and x = -a/e

Ellipse - Symmetric About y-Axis

Center : (0, 0).

Foci : F(0, ae) and F'(0, -ae)

Vertices : A(0, a) and A'(0, -a).

Equations of directrices : y = a/e and y = -a/e

Hyperbola - Symmetric About x-Axis

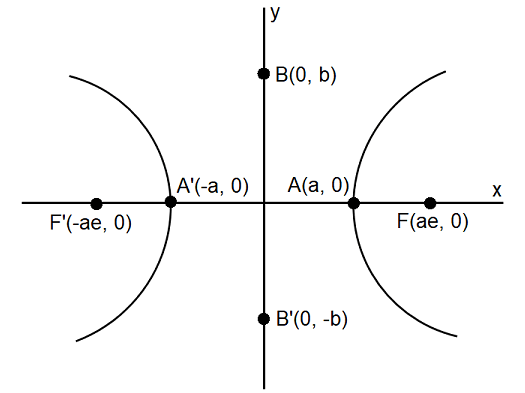
Center : (0, 0).

Foci : F(ae, 0) and F'(-ae, 0)

Vertices : A(a, 0) and A'(-a, 0).

Equations of directrices : x = a/e and x = -a/e

Hyperbola - Symmetric About y-Axis

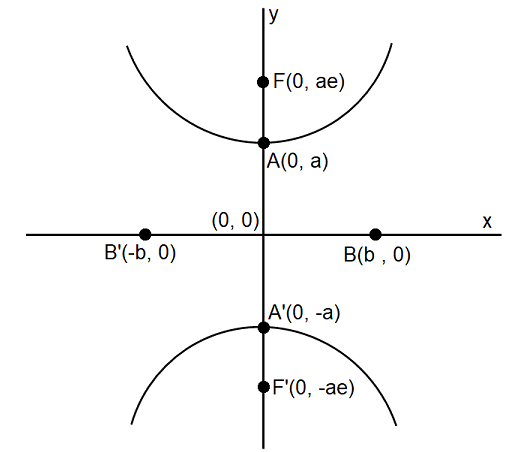
Center : (0, 0).

Foci : F(0, ae) and F'(0, -ae)

Vertices : A(0, a) and A'(0, -a).

Equations of directrices : y = a/e and y = -a/e

Examples 1-2 : Find center, foci, vertices, and equations of directrices of of the following ellipses :

Example 1 :

Solution :

The given ellipse is symmetric about x-axis.

Center : (0, 0)

Foci :

F(ae, 0)  and  F'(-ae, 0)

Foci are F(4, 0) and F'(-4, 0).

Vertices :

A(a, 0)  and  A'(-a, 0)

A(5, 0)  and  A'(-5, 0) 

Equations of directrices :

x = a/e  and  x = -a/e

Example 2 :

Solution :

The given ellipse is symmetric about y-axis.

Center : (0, 0)

Foci :

F₁ (ae, 0) F₂ (-ae, 0)

Foci are F(0, √7) and F'(0, √7).

Vertices :

A(0, a)  and  A'(0, -a)

A(0, √10)  and  A'(0, -√10) 

Equations of directrices :

y = a/e  and  y = -a/e

Example 3 :

Solution :

The given hyperbola is symmetric about x-axis.

Center : (0, 0)

Foci :

F(ae, 0)  and  F'(-ae, 0)

Foci are F(13, 0) and F'(-13, 0).

Vertices :

A (a, 0) A' (-a, 0) 

A (5, 0) A' (-5, 0) 

Equation of directrices :

x = a/e  and  x = -a/e

Example 4 :

Solution :

The given hyperbola is symmetric about y-axis.

Center : (0, 0)

Foci :

F(0, ae)  and  F'(0, -ae)

Foci are F(0, 5) and F'(0, -5).

Vertices :

A(0, a)  and  A'(0, -a) 

A(0, 4)  and  A'(0, -4) 

Equations of directrices :

y = a/e  and  y = -a/e

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.