FINDING MISSING MEASURES IN SIMILAR TRIANGLES
About "Finding missing measures in similar triangles"
Finding missing measures in similar triangles :
Because corresponding angles are congruent and corresponding sides are proportional in similar triangles, we can use similar triangles to solve real-world problems.
Finding missing measures in similar triangles - Practice problems
Problem 1 :
While playing tennis, David is 12 meters from the net, which is 0.9 meter high. He needs to hit the ball so that it just clears the net and lands 6 meters beyond the base of the net. At what height should Matt hit the tennis ball ?
Solution :
Step 1 :
Draw an appropriate diagram to the given information
In the above diagram,
BC = Height of the net
DE = Height of ball when hit
A = Point at where the ball lands
Step 2 :
Let us compare two corresponding angles of triangle ABC and ADE.
Triangle ABC
m∠A (Common angle)
m∠B (Right angle)
Triangle ADE
m∠A (Common angle)
m∠D (Right angle)
Because two angles in one triangle are congruent to two angles in the other triangle, the two triangles are similar.
Step 3 :
Since the triangle ABC and ADE are similar triangles, corresponding side lengths are proportional.
So, we have
AD / DB = DE / BC
(AB + BD) / DB = DE / BC
Substitute the lengths from the figure.
(6 + 12) / 6 = h / 0.9
18 / 6 = h / 0.9
3 = h / 0.9
Multiply both sides by 0.9
3 ⋅ 0.9 = (h/0.9) ⋅ 9
2.7 = h
Hence, David should hit the ball at a height of 2.7 meters.
Problem 2 :
Jose is building a wheelchair ramp that is 24 feet long and 2 feet high. She needs to install a vertical support piece 8 feet from the end of the ramp. What is the length of the support piece in inches ?
Solution :
Step 1 :
Draw an appropriate diagram to the given information
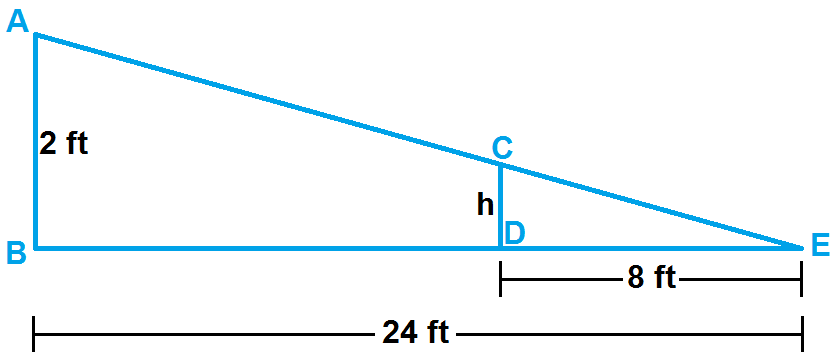
In the above diagram,
AB = Height of the chair
CD = Height of the support piece
E = End of the ramp
Step 2 :
Let us compare two corresponding angles of triangle ABE and CDE.
Triangle ABE
m∠E (Common angle)
m∠B (Right angle)
Triangle ADE
m∠E (Common angle)
m∠D (Right angle)
Because two angles in one triangle are congruent to two angles in the other triangle, the two triangles are similar.
Step 3 :
Since the triangle ABE and ADE are similar triangles, corresponding side lengths are proportional.
So, we have
DE / BE = CD / AB
Substitute the lengths from the figure.
8 / 24 = h / 2
1 / 3 = h / 2
Multiply both sides by 2.
(1/3) ⋅ 2 = (h/2) ⋅ 2
2/3 ft = h
or
h = 2/3 ft
Step 4 :
Convert feet into inches.
Since 1 ft = 12 inches, we have to multiply by 12 to convert ft into inches.
h = 2/3 ft ----> h = (2/3) ⋅ 12 inches
h = 8 inches
Hence, the length of the support piece is 8 inches.
After having gone through the stuff given above, we hope that the students would have understood "Finding missing measures in similar triangles".
Apart from the stuff given above, if you want to know more about "Finding missing measures in similar triangles", please click here
Apart from the stuff given in this section, if you need any other stuff in math, please use our google custom search here.
WORD PROBLEMS
HCF and LCM word problems
Word problems on simple equations
Word problems on linear equations
Word problems on quadratic equations
Area and perimeter word problems
Word problems on direct variation and inverse variation
Word problems on comparing rates
Converting customary units word problems
Converting metric units word problems
Word problems on simple interest
Word problems on compound interest
Word problems on types of angles
Complementary and supplementary angles word problems
Markup and markdown word problems
Word problems on mixed fractrions
One step equation word problems
Linear inequalities word problems
Ratio and proportion word problems
Word problems on sets and venn diagrams
Pythagorean theorem word problems
Percent of a number word problems
Word problems on constant speed
Word problems on average speed
Word problems on sum of the angles of a triangle is 180 degree
OTHER TOPICS
Time, speed and distance shortcuts
Ratio and proportion shortcuts
Domain and range of rational functions
Domain and range of rational functions with holes
Graphing rational functions with holes
Converting repeating decimals in to fractions
Decimal representation of rational numbers
Finding square root using long division
L.C.M method to solve time and work problems
Translating the word problems in to algebraic expressions
Remainder when 2 power 256 is divided by 17
Remainder when 17 power 23 is divided by 16
Sum of all three digit numbers divisible by 6
Sum of all three digit numbers divisible by 7
Sum of all three digit numbers divisible by 8
Sum of all three digit numbers formed using 1, 3, 4
Sum of all three four digit numbers formed with non zero digits
