FINDING NATURE OF ROOTS OF QUADRATIC EQUATION BY GRAPHING
To obtain the roots of the quadratic equation in the form ax2 + bx + c = 0 graphically, first we have to draw the graph of y = ax2 + bx + c .
The solutions of the quadratic equation are the x coordinates of the points of intersection of the curve with X axis.
Finding the Nature of Roots of Quadratic Equations Graphically
To determine the nature of solutions of a quadratic equation, we can use the following procedure.
(i) If the graph of the given quadratic equation intersects the x-axis in two distinct points, then the given equation has two real and unequal roots.
(ii) If the graph of the given quadratic equation touches the x-axis in only one point, then the given equation has only one root which is same as saying two real and equal roots.
(iii) If the graph of the given equation does not intersect the x-axis at any point, then the given equation has no real root.
Solved Questions
Question 1 :
Graph the following quadratic equations and state their nature of solutions.
x2 + x + 7 = 0
Solution :
Draw the graph for the function y = x2 + x + 7
Let us give some random values of x and find the values of y.
|
x -4 -3 -2 -1 0 1 2 3 4 |
x2 16 9 4 1 0 1 4 9 16 |
x -4 -3 -2 -1 0 1 2 3 4 |
+7 7 7 7 7 7 7 7 7 7 |
y 19 13 9 7 7 9 13 19 27 |
Points to be plotted :
(-4, 19) (-3, 13) (-2, 9) (-1, 7) (0, 7) (1, 9) (2, 13) (3, 19) (4, 27)
To find the x-coordinate of the vertex of the parabola, we may use the formula x = -b/2a
x = -1/2(1) = -1/2
By applying x = -1/2, we get the value of y.
y = (-1/2)2 + (-1/2) + 7
y = (1/4) - (1/2) + 7
y = (1 - 2 + 28)/4 = 27/4
Vertex (1/2, 27/4)
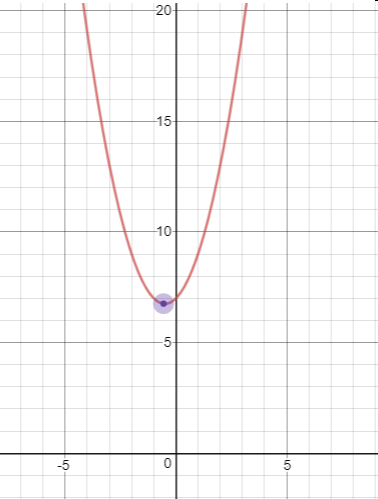
The graph of the given parabola does not intersect the x-axis at any point. Hence it has no real roots.
Question 2 :
Graph the following quadratic equations and state their nature of solutions.
x2 − 9 = 0
Solution :
Let us give some random values of x and find the values of y.
|
x -4 -3 -2 -1 0 1 2 3 4 |
x2 16 9 4 1 0 1 4 9 16 |
-9 -9 -9 -9 -9 -9 -9 -9 -9 -9 |
y 7 0 -5 -8 -9 -8 -5 0 7 |
Points to be plotted :
(-4, 7) (-3, 0) (-2, -5) (-1, -8) (0, -9) (1, -8) (2, -5) (3, 0) (4, 7)
To find the x-coordinate of the vertex of the parabola, we may use the formula x = -b/2a
x = 0/2(1) = 0
By applying x = 0, we get the value of y.
y = 02 - 9
y = -9
Vertex (0, -9)
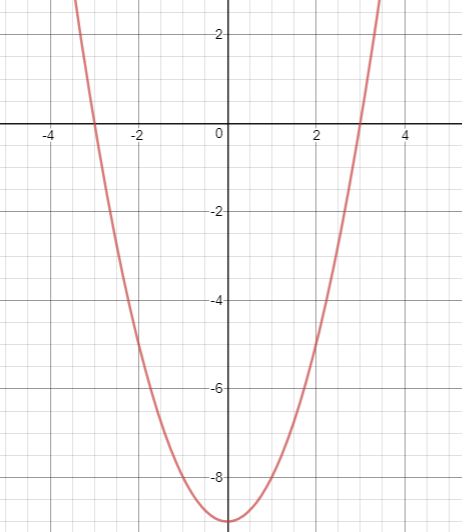
The graph of the given parabola intersects the x-axis at two points. Hence it has two real and unequal roots.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 106)
Feb 04, 25 08:16 AM
Digital SAT Math Problems and Solutions (Part - 106) -
SAT Math Resources (Videos, Concepts, Worksheets and More)
Feb 04, 25 08:15 AM
SAT Math Resources (Videos, Concepts, Worksheets and More) -
Digital SAT Math Problems and Solutions (Part - 107)
Feb 04, 25 08:11 AM
Digital SAT Math Problems and Solutions (Part - 107)

