FINDING THE MISSING SIDE LENGTH USING SIMILAR TRIANGLES
Question :
Find the values of x and y in the figure shown below.
Answer :
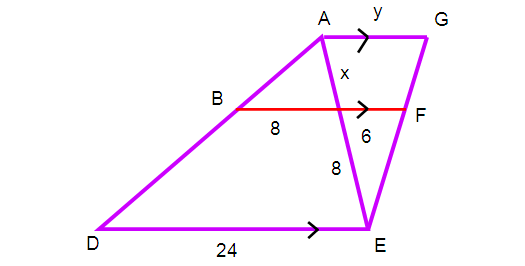
From triangles ABC and triangle ADE
∠ABC = ∠ADE (corresponding angles)
∠A = ∠A (common angle)
So ∆ ABC ~ ∆ ADE
(AC/AE) = (BC/DE)
[x/(x + 8)] = (8/24)
[x/(x + 8)] = (1/3)
3 x = 1 (x + 8)
3 x = x + 8
3 x – x = 8
2 x = 8
x = 8/2
x = 4 cm.
also ∆ EAG and ∆ ECF are congruent triangles
So, (EC/EA) = (CF/AG)
AG = (EA ⋅ CF)/EC
EA = EC + CA
= 8 + 4 ==> 12 cm
y = (6 ⋅ 12)/8
y = 72/8
= 9 cm
The values of x and y are 4 and 9 cm respectively.
Example 2 :
Find the values of x and y in the figure shown below.
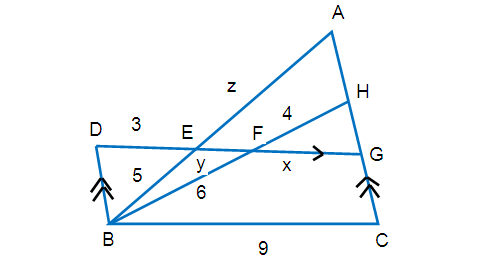
In the diagram shown below, the sides FG and BC are parallel. So, ∆ HFG ~ ∆ HBC.
Then,
(HF/HB) = (FG/BC)
(4/10) = (x/9)
x = (4 ⋅ 10)/4
x = 3.6 cm
In triangle ∆ FBD and ∆FHG the sides BD and GH are parallel,
∠FBD = ∠FHG (alternate angles)
∠BFD = ∠HFG (vertically opposite angels)
By using AA similarity criterion ∆ FBD ~ ∆ FHG
(FG/FD) = (FH/FB)
[x/(y + 3)] = (4/6)
3.6/(y + 3) = (2/3)
3.6(3) = 2 (y + 3)
10.8 = 2 y + 6
2 y = 10.8 – 6
2 y = 4.8
y = 4..8/2
y = 2.4 cm
In triangles ∆ AEG and ∆ ABC, the sides EG and BC are parallel,
∠A = ∠A (common angle)
∠AEG = ∠ABC (corresponding angles)
By using AA similarity criterion ∆ AEG ~ ∆ ABC
(AE/AB) = (EG/BC)
[z/(z + 5 )] = (x + y)/9
[z/(z + 5 )] = 6/9
9 z = 6 (z + 5)
9 z = 6 z + 30
9 z – 6 z = 30
3 z = 30
z = 30/3
z = 10 cm
So length of the side FG = 3.6 cm
Length of the side BF = 2.4 cm
Length of the side AE = 10 cm
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 144)
Apr 14, 25 07:27 PM
Digital SAT Math Problems and Solutions (Part - 144) -
Quadratic Equation Problems with Solutions (Part - 1)
Apr 14, 25 11:33 AM
Quadratic Equation Problems with Solutions (Part - 1) -
Quadratic Equation Problems with Solutions (Part - 2)
Apr 14, 25 11:22 AM
Quadratic Equation Problems with Solutions (Part - 2)
