FORMULA FOR a plus b WHOLE SQUARE

In this section,  you will learn the formula or expansion  for (a + b)².

That is, 

(a + b)²  =  (a + b)(a + b)

(a + b)²  =  a² + ab + ab + b²

(a + b)²  =  a² + 2ab + b²

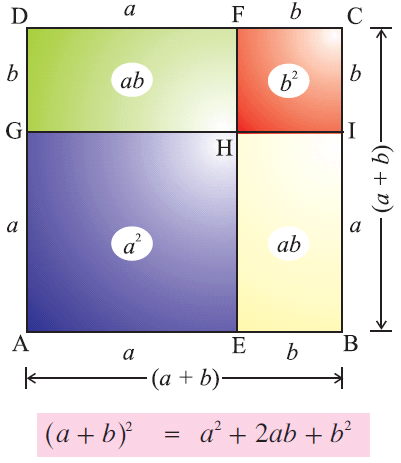
Proving the Expansion of a plus b Whole Square Geometrically

In this section, we are going to see, how to prove the expansion of (a + b)² geometrically. 

We can prove the the expansion of (a + b)² using the area of a square as shown below. 

Solved Problems

Problem 1 : 

Expand : 

(x + y)² 

Solution :

(x + y)² is in the form of (a + b)²

Comparing (a + b)² and (x + y)², we get

a  =  x

b  =  y

Write the formula / expansion for (a + b)².

(a + b)²  =  a² + 2ab + b²

Substitute x for a and y for b. 

(x + y)²  =  x² + 2(x)(y) + y²

(x + y)²  =  x² + 2xy + y²

So, the expansion of (x + y)² is

x² + 2xy + y²

Problem 2 :

Expand : 

(x + 2)² 

Solution :

(x + 2)² is in the form of (a + b)²

Comparing (a + b)² and (x + 2)², we get

a  =  x

b  =  2

Write the formula / expansion for (a + b)².

(a + b)²  =  a² + 2ab + b²

Substitute x for a and 2 for b. 

(x + 2)²  =  x² + 2(x)(2) + 3²

(x + 2)²  =  x² + 4x + 9

So, the expansion of (x + 2)² is

x² + 4x + 9

Problem 3 :

Expand : 

(5x + 3)² 

Solution :

(5x + 3)² is in the form of (a + b)²

Comparing (a + b)² and (5x + 3)², we get

a  =  5x

b  =  3

Write the expansion for (a + b)².

(a + b)²  =  a² + 2ab + b²

Substitute 5x for a and 3 for b. 

(5x + 3)²  =  (5x)² + 2(5x)(3) + 3²

(5x + 3)²  =  25x² + 30x + 9

So, the expansion of (5x + 3)² is

25x² + 30x + 9

Problem 4 : 

If a + b  =  7 and a² + b²  =  29, then find the value of ab. 

Solution :

To get the value of ab, we can use the formula or expansion of (a + b)².

Write the formula / expansion for (a + b)².

(a + b)²  =  a² + 2ab + b²

or

(a + b)²  =  a² + b² + 2ab

Substitute 7 for (a + b)  and 29 for (a² + b²).

7²  =  29 + 2ab

49  =  29 + 2ab

Subtract 29 from each side. 

20  =  2ab

Divide each side by 2. 

10  =  ab

So, the value of ab is 10. 

Problem 5 :

Find the value of :

(√2 + 1/√2)²

Solution :

 (√2 + 1/√2)² is in the form of (a + b)²

Comparing (a + b)² and (√2 + (1/√2)², we get

a  =  √2

b  =  1/√2

Write the expansion for (a + b)².

(a + b)²  =  a² + 2ab + b²

Substitute √2 for a and 1/√2 for b. 

(√2 + 1/√2)²  =  (√2)² + 2(√2)(1/√2) + (1/√2)²

(√2 + 1/√2)²  =  2 + 2 + 1/2

(√2 + 1/√2)²  =  9/2

So, the value of (√2 + 1/√2)² is

9 / 2

Problem 6 :

Find the value of :

(105)²  

Solution :

Instead of multiplying 105 by 105 to get the value of (105)², we can use the algebraic formula for (a + b)² and find the value of (105)² easily.

Write (105)² in the form of (a + b)².

(105)²  =  (100 + 5)²

Write the expansion for (a + b)².

(a + b)²  =  a² + 2ab + b²

Substitute 100 for a and 5 for b. 

(100 + 5)²  =  (100)² + 2(100)(5) + (5)²

(100 + 5)²  =  10000 + 1000 + 25

(105)²  =  11025

So, the value of (105)² is

11025

Algebraic Identities

Algebraic identities are equalities which remain true regardless of the values of any variables which appear within it.

To know more identities in Algebra, 

Please click here

In our website, we have provided two calculators for algebra identities.

One is to find the expansion for (a + b)ⁿ and other one is to find the expansion for (a - b)ⁿ.  

Please click the below links to get expansion calculator that you need.  

Expansion Calculator for (a + b)ⁿ

Expansion Calculator for (a - b)ⁿ

If you would like to have problems on algebraic identities, please click the link given below. 

Worksheet on Algebraic Identities

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.