FREE SHSAT MATH  PRACTICE TEST

Question 10 :

The perimeter of a triangle, all of whose sides have integer length, is 19.  A possible length for the largest side is

(A)  6  (B)  8  (C)  10  (D)  15  (E)  17

Solution :

The sum of three lengths is given as 19. If the longest side is 10, the sum of the other two sides would be 9, which is impossible.

Hence the longest side must be less than 10. If the longest side is only 6, then the perimeter cannot be more than 18.

So the answer is 8.

Question 11 :

A value of x that satisfies the inequality 2x – 10 > 3x is

(A)  -11  (B)  -10  (C)  -1  (D)  0  (E)  12

Solution :

2x – 10 > 3x

Subtract 2x on both sides, we get

2x - 2x - 10 > 3x - 2x

-10 > x

Hence the answer is -10

Question 12 :

The midpoints of the four sides of square ABCD are joined to form square WXYZ as shown.  The area of square WXYZ is 16.  The area of triangle YCX is

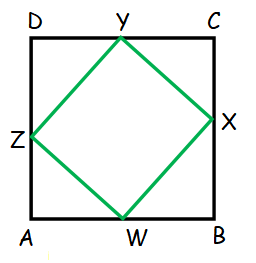
(A)  4  (B)  2  (C)  1.5  (D)  1  (E)  none of these

Solution :

Area of square WXXZ  =  16

a²  =  16

a  =  4

Base of YCX is = 2, height = 2

Area of triangle YCX  =  (1/2) ⋅ base ⋅ height

  =  (1/2) ⋅ 2 ⋅ 2

  =  2 square units

Hence the answer is 2 square units.

Question 13 :

The mean of 3 numbers is 7.  The mean of 4 other numbers is 8.  The mean of 5 other numbers is 11.  What is the mean of all 12 numbers?

(A)  4  (B)  4(2/13)  (C)  8(2/3)  (D)  9  (E)  36

Solution :

Mean of 3 numbers  =  7

Sum of 3 numbers/3  =  7

Sum of 3 numbers  =  21

Sum of 4 numbers/4  =  8

Sum of 4 numbers  =  32 

Sum of 5 numbers/5  =  11

Sum of 5 numbers  =  55

Mean of 12 numbers  =  Sum of 12 numbers / 12

=  (21 + 32 + 55) / 12

=  108/12

=  9

Question 14 :

There were 20 socks in a drawer.  Of these, 8 were blue, 10 were black, and 2 were white.  Someone then removed 4 blue socks.  If one sock is now drawn at random, what is the probability that it is not white?

(A)  1/10  (B)  9/10  (C)  1/8  (D)  7/8

(E)  None of these

Solution :

Number of socks  =  20

Number of blue socks  =  8 - 4  =  4

umber of black socks  =  10

Number of white socks  =  2

After removing 4 blue socks, we will have 16 socks.

Probability of getting other than white socks 

  =  (10 + 4)/16

  =  14/16

  =   7/8

Hence the answer is 7/8.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.