GRAPHING ENLARGEMENTS

When a dilation in the coordinate plane has the origin as the center of dilation, we can find points on the dilated image by multiplying the x and y coordinates of the original figure by the scale factor.

For example, if the scale factor is 'k', the algebraic representation of the dilation is

(x, y) → (kx, ky)

For enlargements, k > 1.

Example 1 :

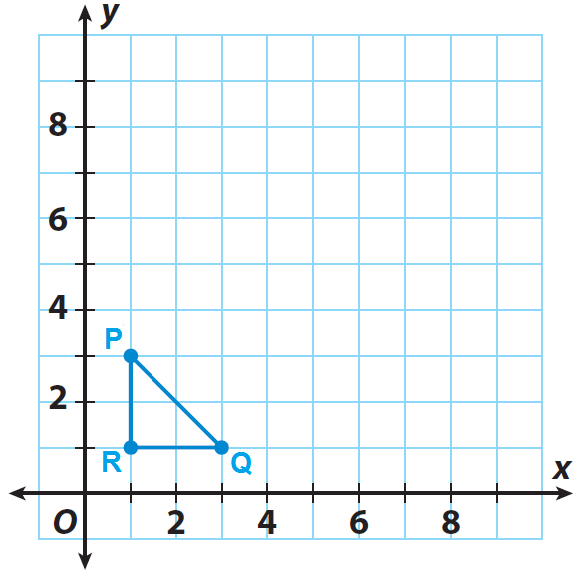
The triangle PQR shown on the grid is the pre-image. If the center of dilation is the origin and the scale factor is 3, graph the dilated image P'Q'R'.

Solution : 

Step 1 :

List the coordinates of the vertices of the pre image. 

P(1, 3), Q(3, 1) and R(1, 1)

Step 2 :

Since the scale factor is 3, the rule to get the coordinates of the vertices of the image is 

 (x, y) → (3x, 3y)

Step 3 :

List the coordinates of the vertices of the image. 

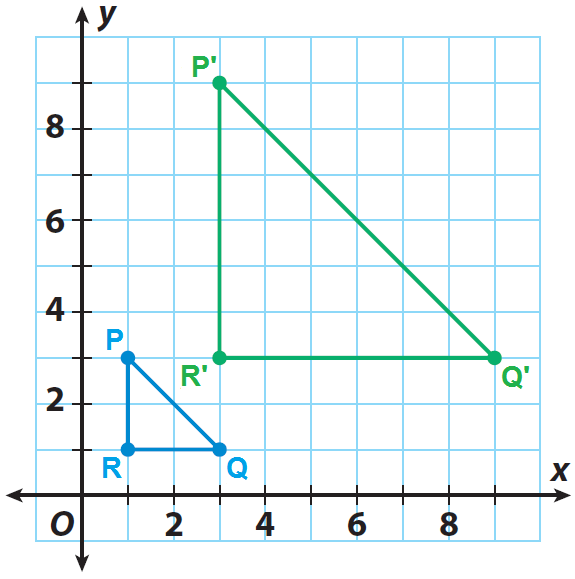
P(1, 3)  --->  P'(3, 9)

 Q(3, 1)  --->  Q'(9, 3)

R(1, 1)  --->  R'(3, 3)

Step 4 : 

Graph the image P'Q'R'.

Example 2 :

The rectangle JKLM shown on the grid is the pre-image. If the center of dilation is the origin and the scale factor is 2, graph the dilated image J'K'L'M'.

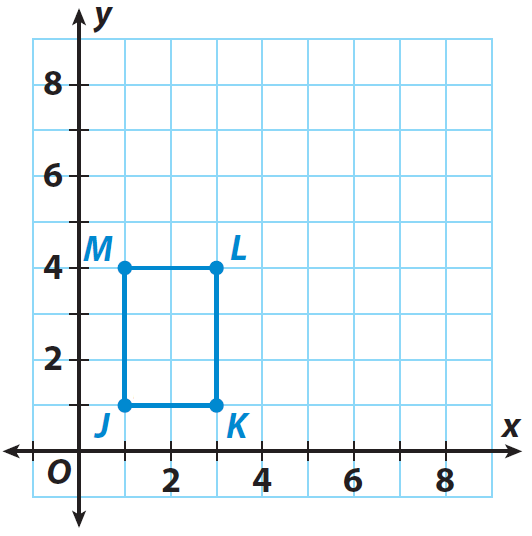
Solution : 

Step 1 :

List the coordinates of the vertices of the pre image. 

J(1, 1), K(3, 1), L(3, 4) and M(1, 4)

Step 2 :

Since the scale factor is 2, the rule to get the coordinates of the vertices of the image is 

 (x, y) → (2x, 2y)

Step 3 :

List the coordinates of the vertices of the image. 

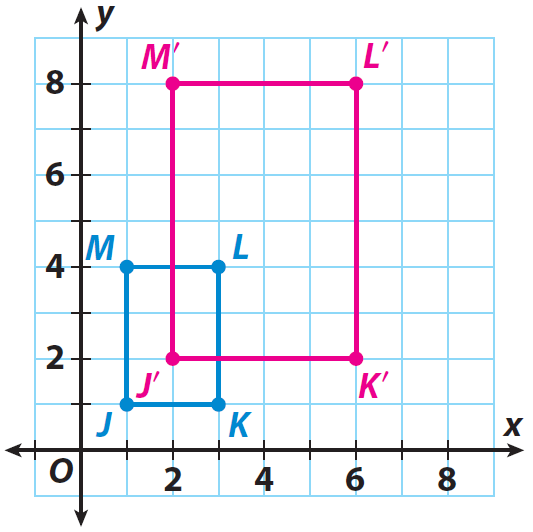
J(1, 1)  --->  J'(2, 2)

 K(3, 1)  --->  K'(6, 2)

L(3, 4)  --->  L'(6, 8)

M(1, 4)  --->  M'(2, 8)

Step 4 : 

Graph the image J'K'L'M'.

Example 3 :

The triangle ABC shown on the grid is the pre-image. If the center of dilation is the origin and the scale factor is 3, graph the dilated image A'B'C'.

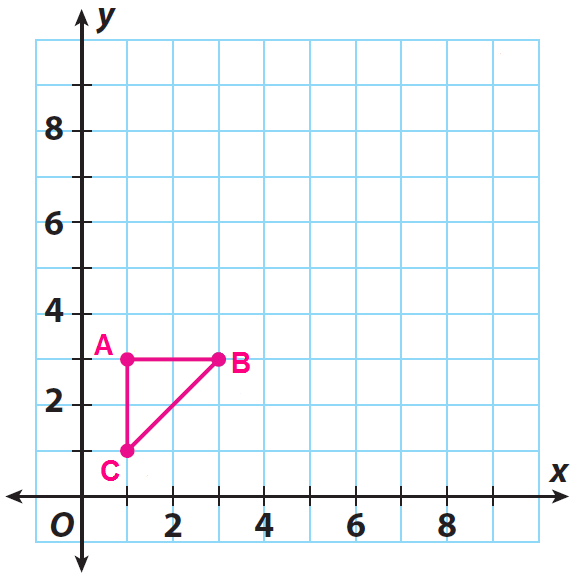
Solution : 

Step 1 :

List the coordinates of the vertices of the pre image. 

A(1, 3), B(3, 3) and C(1, 1)

Step 2 :

Since the scale factor is 3, the rule to get the coordinates of the vertices of the image is 

 (x, y) → (3x, 3y)

Step 3 :

List the coordinates of the vertices of the image. 

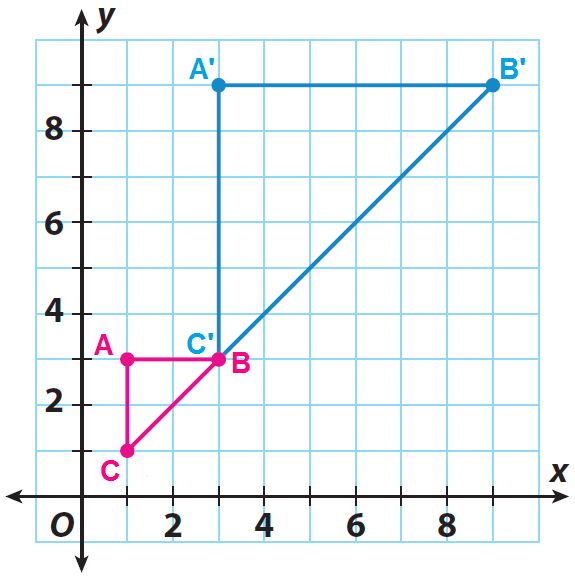
A(1, 3)  --->  A'(3, 9)

 B(3, 3)  --->  B'(9, 9)

C(1, 1)  --->  C'(3, 3)

Step 4 : 

Graph the image A'B'C'.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.