GRAPHING LINEAR EQUATIONS USING INTERCEPTS

Example 1 : 

2x + 3y  =  12

Solution : 

Find the intercepts.

Plot (6, 0) and (0, 4).

Connect with a straight line.

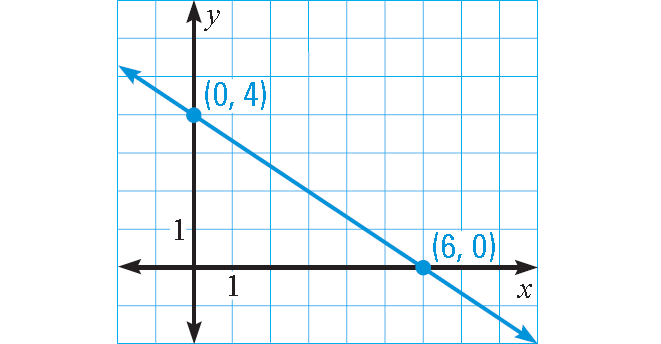
Example 2 : 

-2x + 8y  =  16

Solution : 

Find the intercepts.

Plot (-8, 0) and (0, 2).

Connect with a straight line.

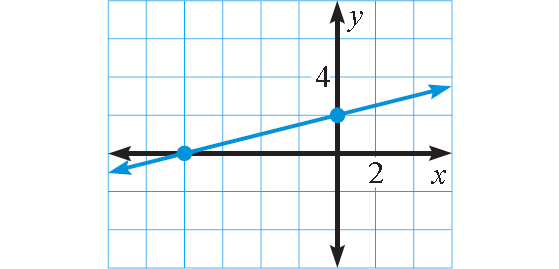
Example 3 : 

y  =  -0.5x - 1.5

Solution : 

Find the intercepts.

Plot (-3, 0) and (0, -1.5).

Connect with a straight line.

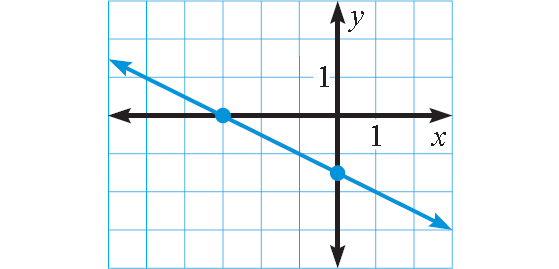
Example 4  : 

y  =  2x/3 + 4

Solution : 

Find the intercepts.

Plot (-6, 0) and (0, 4).

Connect with a straight line.

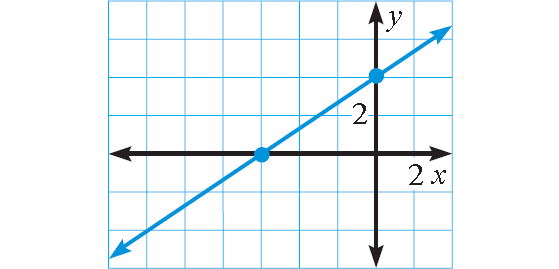
Example 5 :

The function below shows the cost of a hamburger with different numbers of toppings (t).

f(t) = 1.90 + 1.40t

a. What is the y-intercept, and what does it mean?

b. What is the slope, and what does it mean?

c. If Jodi paid $3.30 for a hamburger, how many toppings were on Jodi’s hamburger?

Solution :

f(t) = 1.90 + 1.40t

Comparing with the function y = mx + b

f(t) = cost of hamburger

t = different number of toppings

a) y-intercept = 1.90

cost of hamburger with no toping is $1.90

b) Slope = 1.40

Based on number of toppings, the cost of hamburger will increase with the rate of change 1.40.

c) When cost of hamburger = 3.30

3.30 = 1.90 + 1.40x

Subtracting 1.90, we get

3.30 - 1.90 = 1.40x

1.40x = 1.4

x = 1.4/1.40

x = 1

There is 1 toping is added.

Example 6 :

The function below shows the cost of an ice cream sundae with different numbers of toppings (t).

f(t) = 2.25 + 0.75t

a. What is the y-intercept, and what does it mean?

b. What is the slope, and what does it mean?

c. If Kaye paid $6.00 for a sundae, how many toppings were on Kaye’s sundae?

Solution :

f(t) = 2.25 + 0.75t

Comparing with the function y = mx + b

f(t) = cost of ice cream

t = different number of toppings

a) y-intercept = 2.25

cost of ice cream with no toping is $2.25

b) Slope = 0.75

Based on number of toppings, the cost of ice cream will increase with the rate of change 0.75

c) When cost of ice cream = 6.50

6.50 = 2.25 + 0.75t

6.50 - 2.25 = 0.75t

4.25 = 0.75t

t = 4.25 / 0.75

t = 5.6

There is 6 toping is added.

Example 7 :

Colby put $100 in a savings account. The graph below shows how the amount in the account would increase over the next ten years. What does the y-intercept represent?

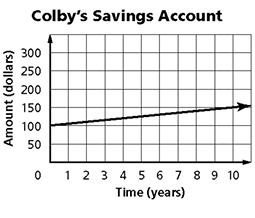
Solution :

Here the y-intercept is $100.

At the beginning, in his bank account he had $100.

Example 8 :

Write the equation of the line in slope-intercept form.

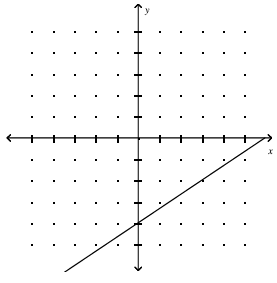
Solution :

From the graph given above, we know that y-intercept = -4

Creating the equation in slope intercept form,

y = mx + b

y = mx - 4

Tracing one more point which lies on the line, we get (3, -2). Applying this point in the equation above

-2 = m(3) - 4

-2 + 4 = 3m

3m = 2

m = 2/3

Applying the slope, we get

y = (2/3)x - 4

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.