HOW TO SKETCH THE GRAPH OF SINUSOIDAL FUNCTION
The general form of a sinusoidal function :
y = a sin [k(x - b)] + N
To sketch the graph of a sinuoidal function, first we have to sketch the graph of a basic sine function, which is
y = sin (x)
We have one pattern of y = sin (x) from x = 0 to x = 2π.
Then, we have to apply the following transformations one by one in the given order to get the graph of the given sinusoidal function.
vertical strech/compression (a)
horizontal stretch/compression (k)
reflection over x-axis (if a < 0)
horizontal shift (b)
vertical shift (N)
Example :
Sketch the graph of
Solution :
Sketch the graph of y = sin (x).
When x = 0,
y = sin (0)
y = 0
y = 1
When x = π,
y = sin (π)
y = 0
y = -1
When x = 2π,
y = sin (2π)
y = 0
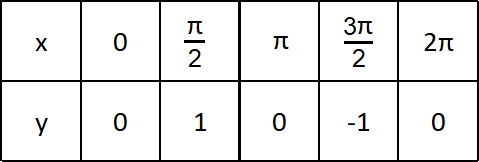
We get the following points from the above table.
Plot the above points on a xy-plane and sketch the graph of y = sin (x).
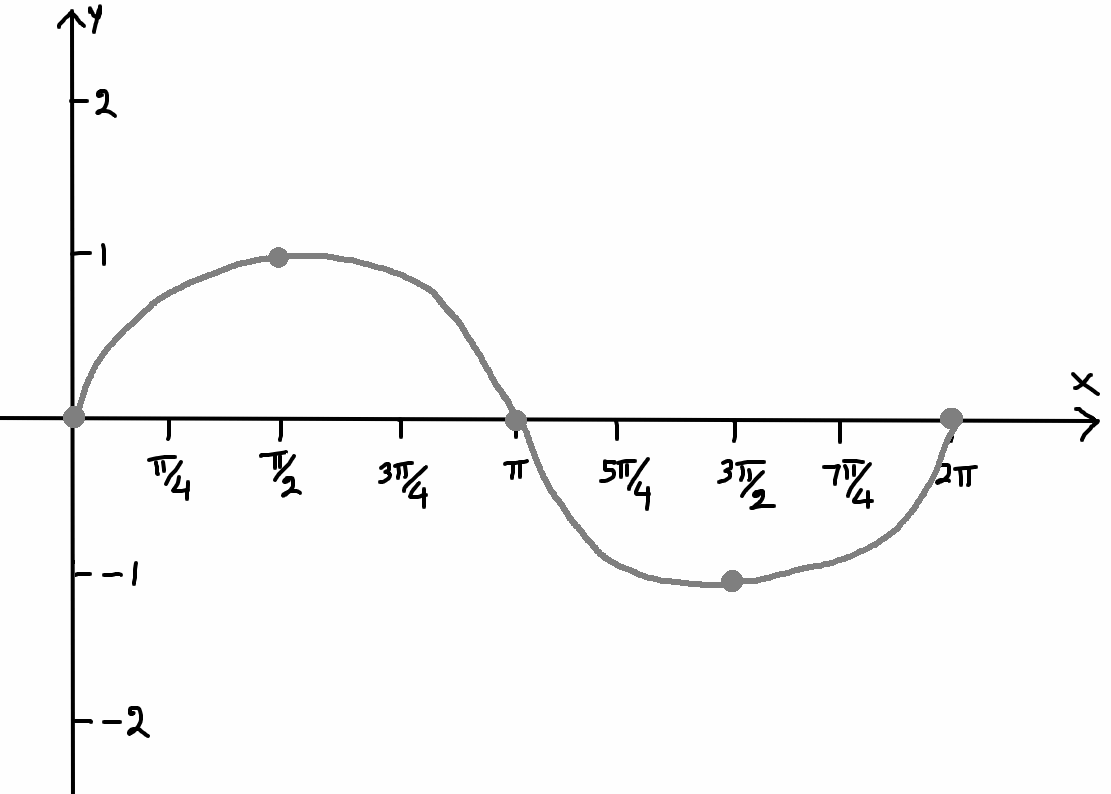
Comparing
y = a sin [k(x - b)] + N
and
we get
a = 2 and k = 2
Vertical Stretch/Compression :
a = 2 refers to vertical stretch by a factor of 2.
That is, at each point on the above graph, multiply the y-coordinate by 2.
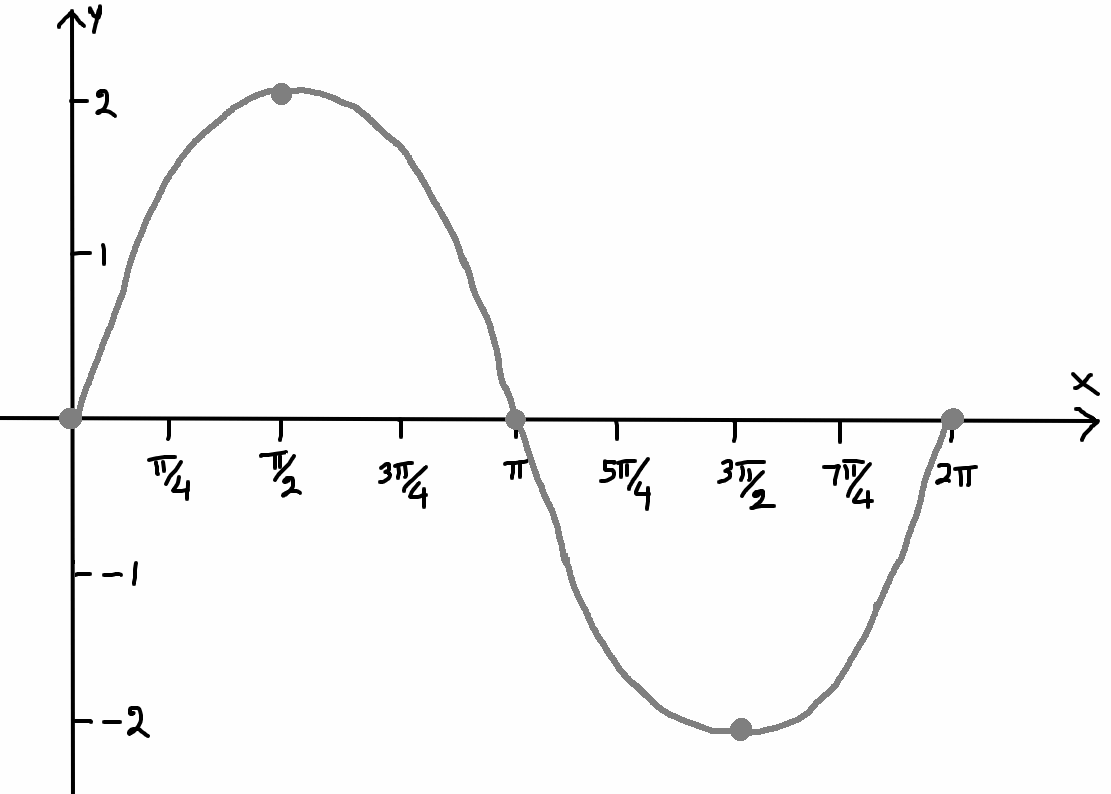
Horizontal Stretch/Compression :
k = 2 refers to horizontal compression by a factor of 2.
That is, at each point on the above graph, multiply the x-coordinate by ½.
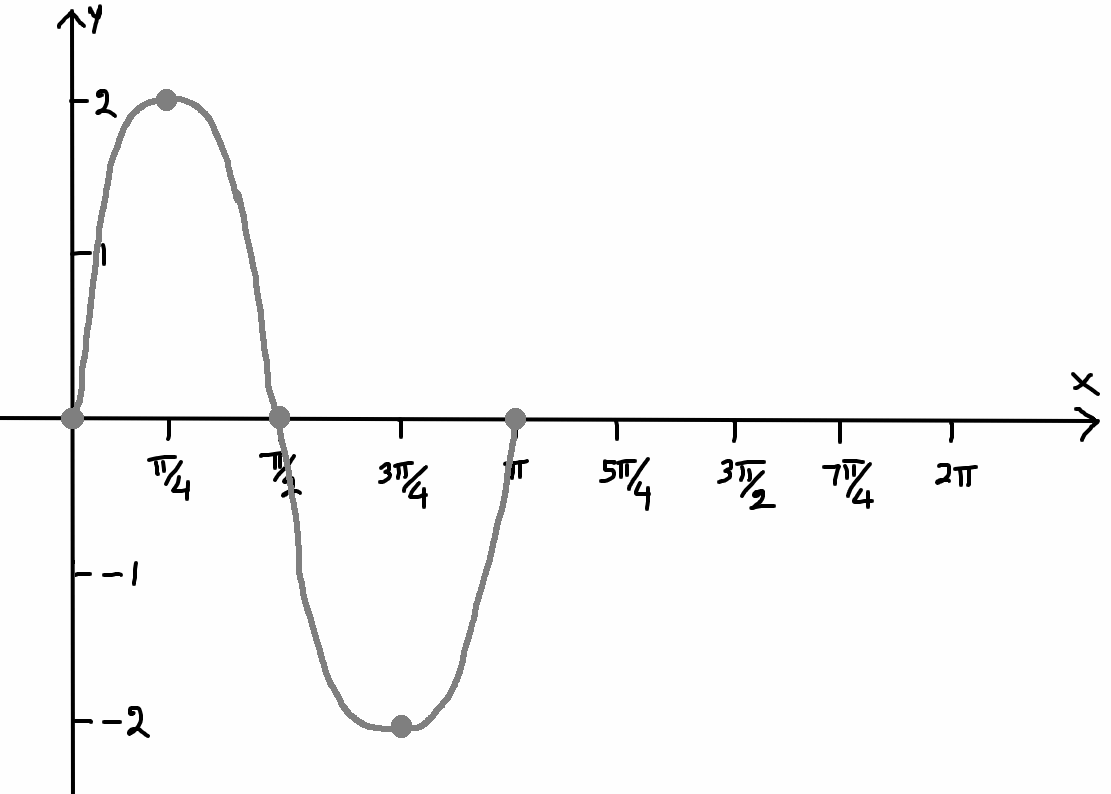
Horizontal Shift :
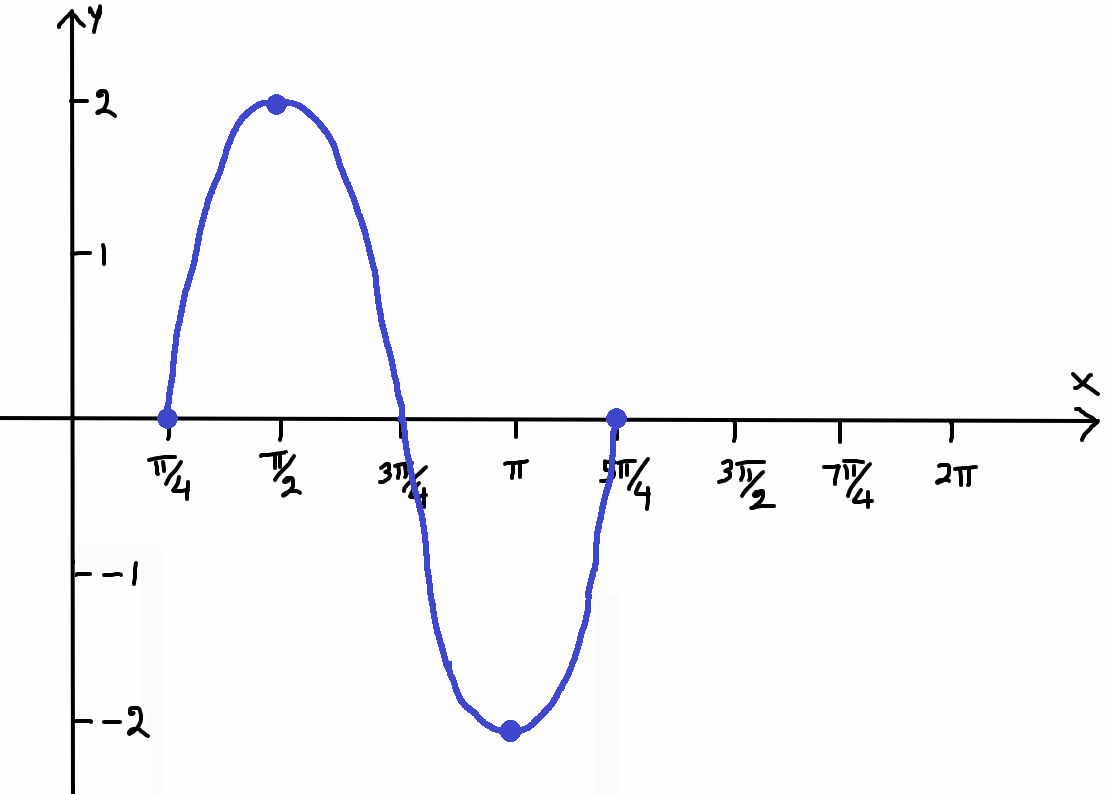
The above is the graph of the given sinusoidal function. In the given sinusoidal function, since there is no value at the place of N, there is no vertical shift.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 142)
Apr 11, 25 06:26 PM
Digital SAT Math Problems and Solutions (Part - 142) -
Digital SAT Math Problems and Solutions (Part - 141)
Apr 11, 25 10:38 AM
Digital SAT Math Problems and Solutions (Part - 141) -
Logarithm Problems and Solutions (Part - 3)
Apr 11, 25 08:19 AM
Logarithm Problems and Solutions (Part - 3)