GRAPHING USING INTERCEPTS WORKSHEET
Use intercepts to graph the line described by each equation.
Problem 1 :
-x + 4y = 4
Problem 2 :
y = 2x - 4
Problem 3 :
2x + 3y + 6 = 0
Problem 4 :
y = 4x + 4
Problem 5 :
x + 4y = -4
Problem 6 :
-x + 2y = 4
Problem 7 :
The distance time graph shows Carloβs motion in front of a sensor.
a) Identify the d-intercept and explain what it means
b) Identify the t-intercept and explain what it means
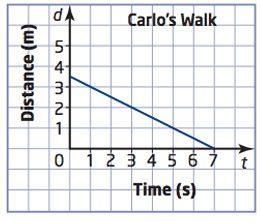
c) Describe the instructions you would give someone walking in front of a sensor to reproduce this graph.
Problem 8 :
Consider the line
π₯ + 4π¦ = β4
To graph this line, you could:
a) determine the x- and y-intercepts
b) Create a table of values
c) use the equation to find the coordinates of three points on the line Which method of graphing do you prefer in this case? Explain.
Detailed Answer Key
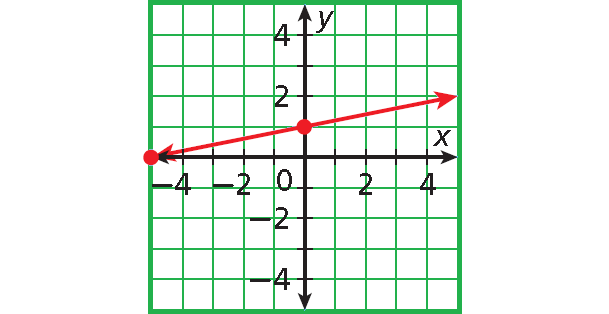
1. Answer :
Find the intercepts.
x-intercept :
-x + 4y = 4
-x + 4(0) = 4
-x + 0 = 4
x = -4
(-4, 0)
y-intercept :
-x + 4y = 4
-0 + 4y = 4
4y = 4
y = 1
(0, 1)
Plot (-4, 0) and (0, 1).
Connect with a straight line.
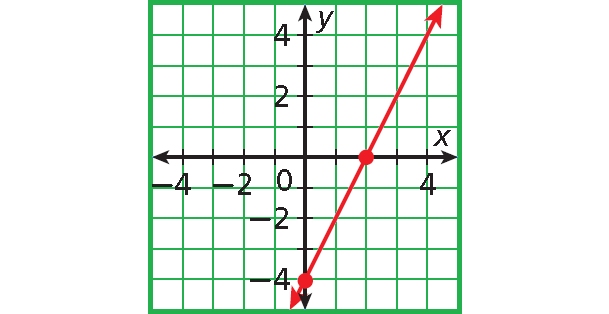
2. Answer :
Find the intercepts.
x-intercept :
y = 2x - 4
0 = 2x - 4
4 = 2x
2 = x
(2, 0)
y-intercept :
y = 2x - 4
y = 2(0) - 4
y = 0 - 4
y = -4
(0, -4)
Plot (2, 0) and (0, -4).
Connect with a straight line.
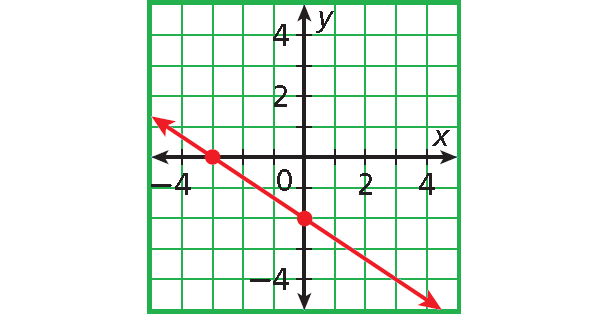
3. Answer :
Find the intercepts.
x-intercept :
2x + 3y + 6 = 0
2x + 3(0) + 6 = 0
2x + 0 + 6 = 0
2x + 6 = 0
2x = -6
2x/2 = -6/2
x = -3
(-3, 0)
y-intercept :
2x + 3y + 6 = 0
2(0) + 3y + 6 = 0
0 + 3y + 6 = 0
3y + 6 = 0
3y = -6
3y/3 = -6/3
y = -2
(0, -2)
Plot (-3, 0) and (0, -2).
Connect with a straight line.
4. Answer :
Find the intercepts.
x-intercept :
y = 4x + 4
0 = 4x + 4
-4 = 4x
-1 = x
(-1, 0)
y-intercept :
y = 4x + 4
y = 4(0) + 4
y = 0 + 4
y = 4
(0, 4)
Plot (-1, 0) and (0, 4).
Connect with a straight line.
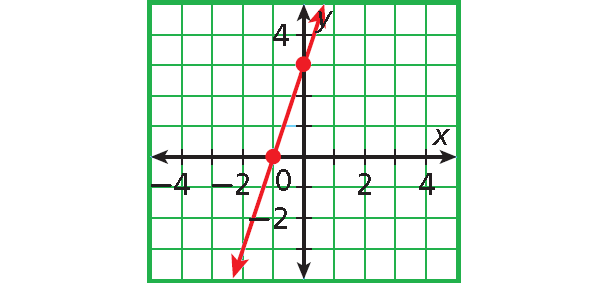
5. Answer :
Find the intercepts.
x-intercept :
x + 4y = -4
x + 4(0) = -4
x + 0 = -4
x = -4
(-4, 0)
y-intercept :
x + 4y = -4
0 + 4y = -4
4y = -4
y = -1
(0, -1)
Plot (-4, 0) and (0, -1).
Connect with a straight line.
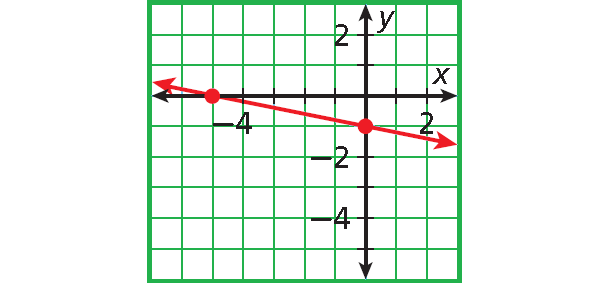
6. Answer :
Find the intercepts.
x-intercept :
-x + 2y = 4
-x + 2(0) = 4
-x + 0 = 4
-x = 4
x = -4
(-4, 0)
y-intercept :
-x + 2y = 4
-0 + 2y = 4
0 + 2y = 4
2y = 4
y = 2
(0, 2)
Plot (-4, 0) and (0, 2).
Connect with a straight line.
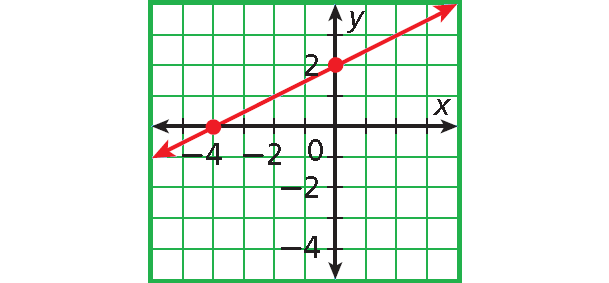
7. Answer :
a) Approximately the d-intercept is 3.5, which means he is at 3.5 m distance from the motion sensor.
b) Here t-intercept is 7. Carol stops after 7 seconds.
c) Start at a distance of 3.5m from the motion sensor and walk at a constant speed of 0.5 m/s for 7s, then stop
8. Answer :
π₯ + 4π¦ = β4
To graph this line, you could:
a) determining the x- and y-intercepts :
|
x-intercept : Put y = 0 x + 4(0) = -4 x = -4 |
y-intercept : Put x = 0 0 + 4y = -4 4y = -4 y = -4/4 y = -1 |
x-intercept is (-4, 0) and y-intercept is (0, -1).
b)
|
When x = -1, π₯ + 4π¦ = β4 -1 + 4y = -4 4y = -4 + 1 4y = -3 y = -3/4 |
When x = 0 0 + 4π¦ = β4 4y = -4 y = -4/4 y = -1 |
|
When x = 1 1 + 4π¦ = β4 4y = -4 - 1 4y = -5 y = -5/4 |
When x = 2 2 + 4π¦ = β4 4y = -4 - 2 4y = -6 y = -6/4 y = -3/2 |
|
x -1 0 1 2 |
y -3/4 -1 -5/4 -3/2 |
(-1, -3/4) (0, -1) (1, -5/4) and (2, -3/2)
c.
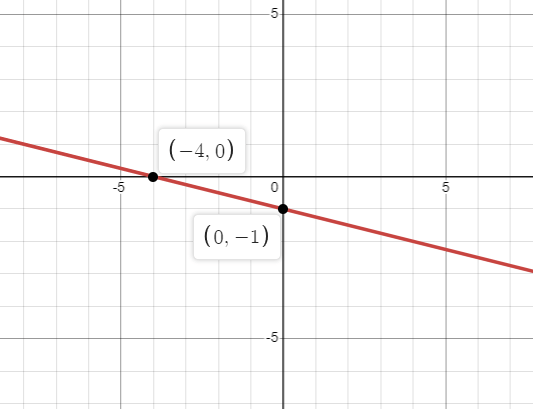
To draw the graph, we can use two different ways.
i) graphing using intercepts :
x-intercept is (-4, 0) and y-intercept is (0, -1)
Joining above points, we get the straight line.
ii) Using slope intercept :
From the y-intercept -1, to get the next point we have to go 1 unit up and 4 units to the left. In the same way, we have to trace the other points and draw the straight line.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
Β©All rights reserved. onlinemath4all.com
Recent Articles
-
SAT Math Resources (Videos, Concepts, Worksheets and More)
Dec 21, 24 02:20 AM
SAT Math Resources (Videos, Concepts, Worksheets and More) -
Digital SAT Math Problems and Solutions (Part - 90)
Dec 21, 24 02:19 AM
Digital SAT Math Problems and Solutions (Part - 90) -
Digital SAT Math Problems and Solutions (Part - 89)
Dec 20, 24 06:23 AM
Digital SAT Math Problems and Solutions (Part - 89)
