HORIZONTAL AND VERTICAL SHIFTS

Horizontal shift of a function is moving its graph to left or right and vertical shift of a function is moving its graph up or down.

Solved Problems

Problems 1-3 : Refer the graph of f(x) given below.

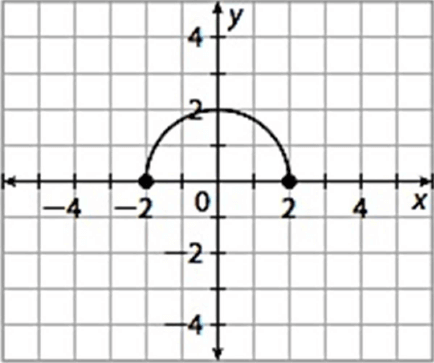
Problem 1 :

Sketch the graph of g(x) such that

g(x) = f(x) - 3

Solution :

In f(x) - 3, 3 is subtracted from f. So, there is a vertical shift of 3 units down.

To get the graph of g(x), the graph of f(x) has to be shifted 3 units down.

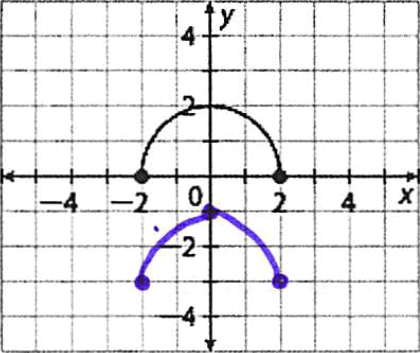
Problem 2 :

Sketch the graph of h(x) such that

h(x) = f(x - 2)

Solution :

In f(x - 2), 2 is subtracted from x. So, there is an horizontal shift of 2 units to the right.

To get the graph of h(x), the graph of f(x) has to be shifted 2 units to the right.

Problem 3 :

Sketch the graph of j(x) such that

j(x) = f(x + 1) + 1

Solution :

In f(x + 1), 1 is added to x. So, there is an horizontal shift of 1 unit to the left.

In f(x + 1) + 1, 1 is added to f. So, there is a vertical shift of 1 unit up.

To get the graph of j(x), the graph of f(x) has to be shifted 1 unit to the left and 1 unit up.

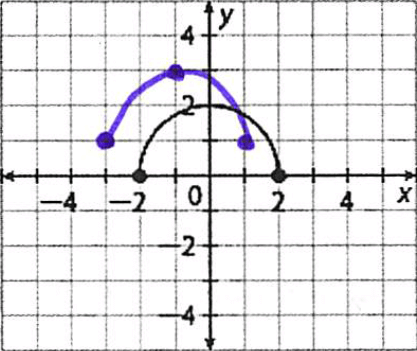
Problems 4-6 : State the transformations on f(x) that woul create the graph of the new function described.

Problem 4 :

g(x) = f(x - 9) + 14

Solution :

Horizontal Shift : 9 units to the right

Vertical Shift : 14 units up

Problem 5 :

Solution :

Vertical Shift : 0.8 units down

Problem 6 :

j(x) = 12 + f(x)

Solution :

j(x) = 12 + f(x)

j(x) = f(x) + 12

NO horizontal shift

Vertical Shift : 12 units up

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.