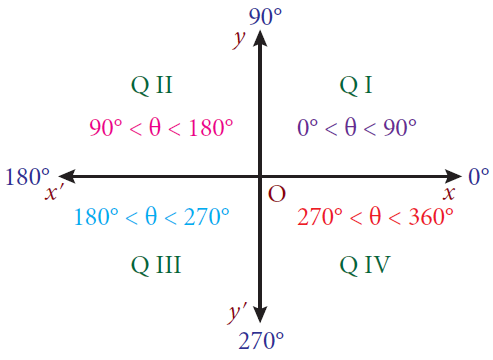
HOW TO DETERMINE IN WHICH QUADRANT AN ANGLE LIES

An angle is said to be in standard position if its vertex is at the origin and its initial side is along the positive x-axis.

An angle is said to be in the first quadrant, if in the standard position, its terminal side falls in the first quadrant.

Similarly, we can define for the other three quadrants. Angles in standard position having their terminal sides along the x-axis or y-axis are called quadrantal angles.

Thus, 0°, 90°, 180°, 270° and 360° are quadrantal angles.

Example :

Identify the quadrant in which each of the following  angles lies. 

(i) 25° (ii) 825° (iii) -55° (iv) 328° (v) -230°

Solution :

(i) 25°

Since 25° is between 0 and 90, it is in quadrant I.

(ii) 825°

825° is greater than 360°. 

When 825° is divided by 360°, the remainder is 105°. 

Since 105° is between 90° and 180°, it is in quadrant II. 

That is, 825° is in quadrant II. 

(iii) -55°

Since it is negative angle, we have to do counter clock rotation.

The difference between 360° and 55° is 305°.

Since 305° is between 270° and 360°, it is in quadrant IV. 

So -55° is in quadrant IV. 

(iv) 328°

Since 328° is between 270° and 360°, it is in quadrant IV.

(v) -230°

Since it is negative angle, we have to do counter clockwise rotation. 

The difference between 360° and 230° is 130°.

Since 130° is between 90° and 180°, it is in quadrant II.

So -230° is in quadrant II.

Apart from the stuff given above, if you need any other stuff in math, please use our google custom search here.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.