HOW TO DRAW HISTOGRAM FOR GROUPED DATA

A two dimensional graphical representation of a continuous frequency distribution is called a histogram.

In histogram, the bars are placed continuously side by side with no gap between adjacent bars. That is, in histogram rectangles are erected on the class intervals of the distribution. The areas of rectangle are proportional to the frequencies.

Now let us look at the steps followed in drawing histogram for grouped data.

Step 1 :

Represent the data in the continuous (exclusive) form if it is in the discontinuous (inclusive) form.

Step 2 :

Mark the class intervals along the X-axis on a uniform scale.

Step 3 :

Mark the frequencies along the Y-axis on a uniform scale.

Step 4 :

Construct rectangles with class intervals as bases and corresponding frequencies as heights.

The method of drawing a histogram is explained in the following example.

Example 1 :

Draw a histogram for the following table which represent the marks obtained by 100 students in an examination:

Solution :

The class intervals are all equal with length of 10 marks. Let us denote these class intervals along the X-axis. Denote the number of students along the Y-axis, with appropriate scale. The histogram is given below.

Scale :

X - axis  =  1 cm  =  10 marks

Y - axis  =  1 cm  =  5 students

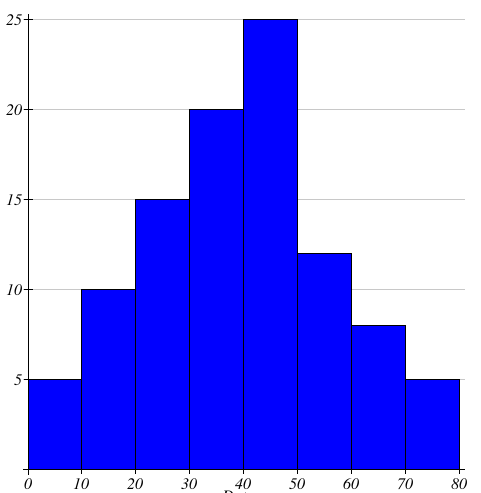
In the above diagram, the bars are drawn continuously. The rectangles are of lengths (heights) proportional to the respective frequencies. Since the class intervals are equal, the areas of the bars are proportional to the respective frequencies.

Example 2 :

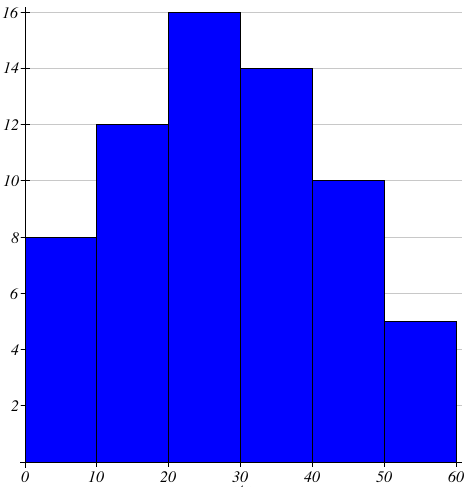
Draw a histogram to represent the following data

Scale :

X - axis  =  1 cm  =  10 units

Y - axis  =  1 cm  =  2 units

Example 3 :

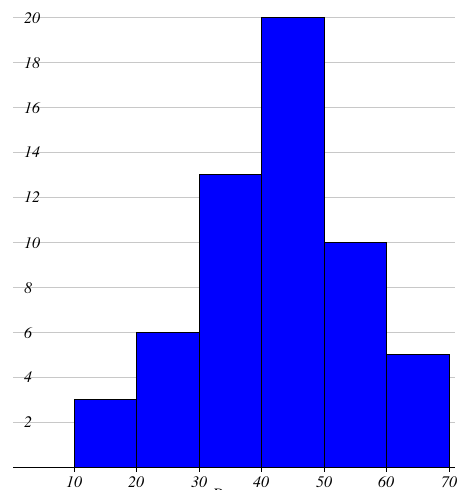
In a study of diabetic patients in a village, the following observations were noted. Represent the above data by a frequency polygon using histogram.

Scale :

X - axis  =  1 cm  =  10 age

Y - axis  =  1 cm  =  2 patients

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.