HOW TO FIND APPROXIMATE VALUE USING BINOMIAL EXPANSION

Example 1 :

Find ³√1001 approximately (two decimal places).

Solution :

³√1001  =  (1001)^1/3  =  (1001)^1/3 

  =  (1000 + 1)^1/3

  =  (1000)^1/3 (1 + (1/1000))^1/3

  =  10 (1 + (1/1000))^1/3

  =  10 [1 + (1/3) (1/1000) + ...................]

  =  10 [1 + (0.333)(0.001) ...................] 

  =  10 [1 + 0.000333 ...................] 

  =  10 [1.000333 ...................] 

  =  10.0033..........

Hence the approximate value of ³√1001 is 10.0033..........

Example 2 :

Prove that ³√(x³ + 6) − ³√(x³ + 3) is approximately equal to 1/x² when x is sufficiently large.

Solution :

³√(x³ + 6)  =  (x³ + 6)^1/3  =  (x³)^1/3 [1 + (6/x³)]^1/3

  =  x [1 + (1/3)(6/x³) + ((1/3)(-2/3))/2 (6/x³)² + ..............]

  =  x [1 + (2/x³) + (4/x⁶) + ..............]

  =  x + (2/x²) - (4/x⁵) + ..............   ------(1)

³√(x³ + 3)  =  (x³ + 3)^1/3  =  (x³)^1/3 [1 + (3/x³]^1/3

  =  x [1 + (1/3)(3/x³) + ((1/3)(-2/3)/2)(3/x³)² + ..............]

  =  x [1 + (1/x³) + (-1/x⁶) + ..............]

  =  x + (1/x²) - (1/x⁵) + ..............   ------(2)

³√(x³ + 6) − ³√(x³ + 3)

  =   (x+(2/x²)-(4/x⁵)+ ..............)-(x+(1/x²)-(1/x⁵) + ..............)

  =  x - x + (2/x²) - (1/x²)

  =  1/x²

Example 3 :

Prove that √(1−x)/(1+x) is approximately equal to 1 − x + x² when x is very small.

Solution :

√(1−x)/(1+x)  =  [(1 - x)/(1 + x)]^1/2

  =  [(1 - x)^1/2/(1 + x)]^1/2

  =  (1 - x)^1/2(1 + x)^-1/2

(1 - x)ⁿ

(1 - x)^1/2  =  1 - (1/2)x + ((1/2)(-1/2)/2!)x²+....................

(1 + x)^-1/2  =  1 - (x/2)x - (x²/8)+....................

(1 + x)^-n

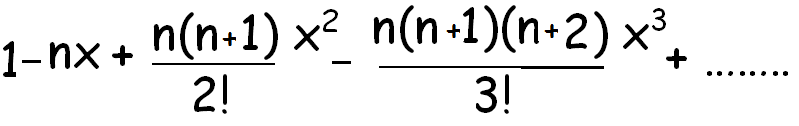
(1 + x)^-1/2  =  1 - (1/2)x + ((1/2)(3/2)/2!)x²+....................

(1 + x)^-1/2  =  1 - (x/2) + (3x²/8) +....................

(1 + x)^-1/2 (1 + x)^-1/2 

  =  (1-(x/2)-(x²/8)+..........)(1 - (x/2)+(3x²/8) +..............)

  = 1 - x/2 + 3x²/8 - x/2 + x²/4 - 3x³/16 - x²/8 + x³/16 +  ..............

  =  1 - [(x/2) + (x/2)] + [(3x²/8) + (x²/4)- (x²/8)

] + .........

  =  1 - x + [(3x²+ 2x²-x²)/8]

  =  1 - x + (4x²/8)

  =  1 - x + x²/2

Hence proved.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.