HOW TO FIND CENTER AND RADIUS FROM AN EQUATION IN COMPLEX NUMBERS

Equation of the Circle from Complex Numbers

The locus of z that satisfies the equation |z − z₀| = r where z₀ is a fixed complex number and r is a fixed positive real number consists of all points z whose distance from z₀ is r .

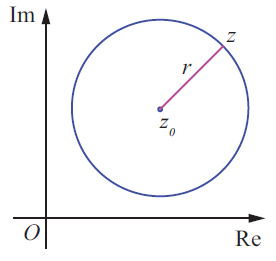
So, |z − z₀| = r is the complex form of the equation of a circle.

(i) |z − z₀| < r represents the points interior of the circle.

(ii) |z − z₀| > r represents the points exterior of the circle.

Note :

|z| = r ==> √x² + y²  =  r

x² + y²  =  r², represents a circle centre at the origin with radius r units.

Question :

Show that the following equations represent a circle, and, find its centre and radius

(i)  |z - 2 - i|  =  3

Solution :

|z - 2 - i|  =  3

|z - (2 + i)|  =  3

It is of the form |z − z₀| = r and so it represents a circle, whose centre and radius are (2, 1) and 3 respectively.

(ii) |2z + 2 − 4i| = 2

Solution :

|2z + 2 − 4i| = 2

2|z + (2 − 4i)/2| = 2

|z + (2 − 4i)/2| = 1

|z - (-(1 - 2i))| = 1

|z - (-1 + 2i)| = 1

It is of the form |z − z₀| = r and so it represents a circle, whose centre and radius are (-1, 2) and 1 respectively. 

(iii) |3z − 6 +12i|  =  8.

Solution :

|3z − 6 +12i|  =  8

3|z - (6 − 12i)/3| = 8

|z - (2 − 4i)| = 8/3

It is of the form |z − z₀| = r and so it represents a circle, whose centre and radius are (2, -4) and 8/3 respectively. 

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.