HOW TO FIND MINORS AND COFACTORS OF A MATRIX
Minor of a Matrix :
Let |A| = |[a ij]| be a determinant of order n.
The minor of an arbitrary element aij is the determinant obtained by deleting the ith row and jth column in which the element aij stands. The minor of aij by Mij.
Cofactors :
The co factor is a signed minor. The cofactor of aij is denoted by Aij and is defined as
Aij = (-1)(i+j) Mij
Example 1 :
Find the minor and cofactor of the following matrix

Solution :
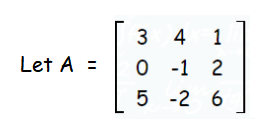
Minor of a11 (Ignore 1st row and 1st column)
Minor of a11 = -6+4 ==> -2
Minor of a12 = 0-10 ==> -10
Minor of a13 = 0+5 ==> 5
Minor of a21 = 24+2 ==> 26
Minor of a22 = 18-5 ==> 13
Minor of a23 = -6-20 ==> -26
Minor of a31 = 8+1 ==> 9
Minor of a32 = 6-0 ==> 6
Minor of a33 = -3-0 ==> -3
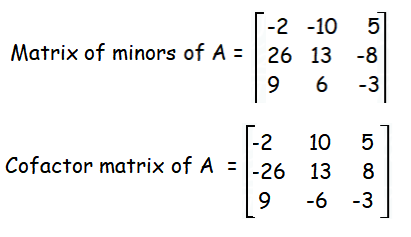
Example 2 :
Find the minor and cofactor of the following matrix
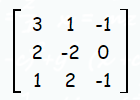
Solution :
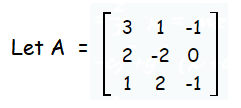
Minor of a11 = 2-0 ==> 2
Minor of a12 = -2-0 ==> -2
Minor of a13 = 4+2 ==> 6
Minor of a21 = -1+2 ==> 1
Minor of a22 = -3+1 ==> -2
Minor of a23 = 6-1 ==> 5
Minor of a31 = 0-2 ==> -2
Minor of a32 = 0+2 ==> 2
Minor of a33 = -6-2 ==> -8
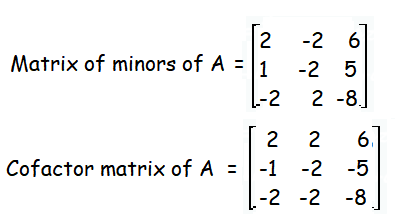
Example 3 :
Find the minors and cofactors of the elements of the second row in the determinant
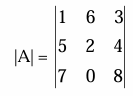
Solution :
The elements in the second row will be a21, a22 and a23
Minor of a21 :
By leaving the second row and first column, then doing the cross multiplication.
= 48 - 0
= 48
Minor of a22 :
By leaving the second row and second column, then doing the cross multiplication.
= 8 - 21
= -13
Minor of a23 :
By leaving the second row and third column, then doing the cross multiplication.
= 0 - 42
= -42
Finding cofactors of second row :
Cofactor of a21 = - minor of a21
= -48
Cofactor of a22 = + minor of a22
= + (-13)
= -13
Cofactor of a23 = - minor of a23
= -(-42)
= 42
Example 4 :
Find the minors and cofactors of the elements of the second row of the determinant
Solution :
The elements in the second row will be a21, a22 and a23
Minor of a21 :
By leaving the second row and first column, then doing the cross multiplication.
= 18 - (-21)
= 18 + 21
= 39
Minor of a22 :
By leaving the second row and second column, then doing the cross multiplication.
= 9 - 6
= 3
Minor of a23 :
By leaving the second row and third column, then doing the cross multiplication.
= -7 - 4
= -11
Finding cofactors of second row :
Cofactor of a21 = - minor of a21
= -39
Cofactor of a22 = + minor of a22
= + 3
Cofactor of a23 = - minor of a23
= -(11)
= -11
Example 5 :
Evaluate each of the following determinants using cofactors:
Solution :
By expanding the first row with cofactors, we get
= +2[0 - (-8)] - 1[3 - 6] + 0[-4 - 0]
= 2[0+8] - 1[-3] + 0
= 16 + 3
= 19
So, the values of the determinant given is 19.
Example 6 :
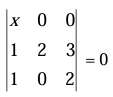
Solution :
x[4 - 0] - 0[2 - 3] + 0[0 - 2] = 0
x(4) + 0 = 0
4x = 0
x = 0/4
x = 0
So, the value of x is 0.
Example 7 :
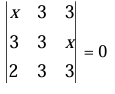
Solution :
x[9 - 3x] - 3[9 - 2x] + 3[9 - 6] = 0
x(9 - 3x) - 3(9 - 2x) + 3(3) = 0
9x - 3x2 - 27 + 6x + 9 = 0
- 3x2 + 6x + 9x + 9 - 27 = 0
- 3x2 + 15x - 18 = 0
Dividing by -3, we get
x2 - 5x + 6 = 0
(x - 3)(x - 2) = 0
x = 3 and x = 2
So, the values of x are 2 and 3.
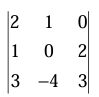
Example 8 :
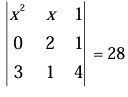
Solution :
x2[8 - 1] - x[0 - 3] + 1[0 - 6] = 28
x2[7] - x[- 3] + 1[- 6] = 28
7x2 + 3x - 6 = 28
7x2 + 3x - 6 - 28 = 0
7x2 + 3x - 34 = 0
7x2 - 14x + 17x - 34 = 0
7x(x - 2) + 17(x - 2) = 0
(7x + 17)(x - 2) = 0
x = -17/7 and x = 2
So, the values of x are 2 and -17/7.
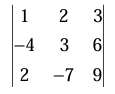
Example 9 :

Use the property to find another matrix whose determinant is equal to Δ.
Solution :
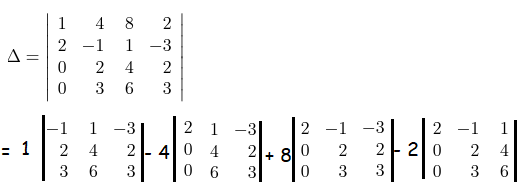
Expansion of first determinant :
-1[6 - 12] - 1[6 - 6] -3[12 - 12]
= -1(-6) - 1(0) - 3(0)
= 6
Expansion of second determinant :
2[12 - 12] - 1[0 - 0] -3[0 - 0]
= 2(0) - 1(0) - 3(0)
= 0
Expansion of third determinant :
2[6 - 6] + 1[0 - 0] -3[0 - 0]
= 2(0) - 1(0) - 3(0)
= 0
Expansion of fourth determinant :
2[12 - 12] + 1[0 - 0] + 1[0 - 0]
= 2(0) - 1(0) + 1(0)
= 0
Applying these values, we get
= 1(6) -4(0) + 8(0) -2(0)
= 6
So, the determinant is 6.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 134)
Apr 02, 25 12:40 AM
Digital SAT Math Problems and Solutions (Part - 134) -
SAT Math Resources (Videos, Concepts, Worksheets and More)
Apr 02, 25 12:35 AM
SAT Math Resources (Videos, Concepts, Worksheets and More) -
Digital SAT Math Problems and Solutions (Part 135)
Apr 02, 25 12:32 AM
Digital SAT Math Problems and Solutions (Part 135)

