SQUARE ROOT OF ALGEBRAIC EXPRESSIONS

Find the square root of the following rational expressions.

Question 1 :

a

400x⁴y¹²z¹⁶ / 100x⁸y⁴z⁴

Solution :

  =  √(400 x⁴ y¹² z¹⁶/ 100 x⁸ y⁴ z⁴)

  =  √(4 y⁸ z¹²/x⁴ )

  =  |(2 y⁴ z⁶)/x²|

Question 2 : 

(7x² + 2√(14)x + 2) / (x² - x/2 + 1/16)

Solution :

  =  √[(7x² + 2 √(14)x + 2)/(x² - x/2 + 1/16)]

(7x² + 2 √(14)x + 2)

First let us find the factors of the quadratic expression in the numerator.

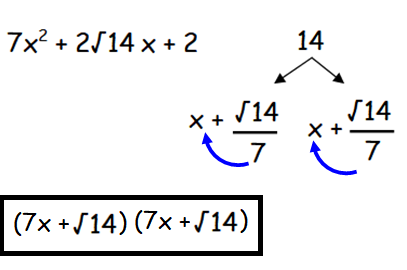
(x² - x/2 + 1/16)

  =  (4x + 1)(4x + 1)

Then, the square root of the given rational expression is 

  =  √[(7x + √14)(7x + √14) / (4x + 1) (4x + 1)]

=  |(7x + √14)/(4x + 1)|

Find the square root of the following algebraic expressions. 

Question 3 :

121(a + b)⁸(x + y)⁸(b - c)⁸

Solution :

  =  √[121 (a + b)⁸ (x + y)⁸ (b - c)⁸]

  =  √[11 ⋅ 11(a + b)⁴ (a + b)⁴ (x +y)⁴(x + y)⁴ (b - c)⁴ (b - c)⁴]

=  11|(a + b)⁴(x + y)⁴ (b - c)⁴|

Question 4 :

4x² + 20x + 25

Solution :

  =  √(4x² + 20x + 25)

Since it is in the form of quadratic equation, let us try to represent in the form of a² + 2ab + b²

  =  √((2x)² + 2(2x)(5) + 5²)

  =  √(2x + 5)²

=  |2x + 5|

Hence the square root of given quadratic polynomial is (2x + 5).

Alternative Method :

  =  √(4x² + 20x + 25)

  =  √(4x² + 10x + 10x + 25)

  =  √2x(2x + 5) + 5(2x + 5)

  =  √(2x + 5) (2x + 5)

  =  |2x + 5|

Question 5 :

9x² - 24xy + 30xz - 40yz +25z² + 16y²

Solution :

  =    √(9x² - 24xy + 30xz - 40yz + 25z² + 16y²)

  =    √(9x² + 25z² + 16y² - 24xy + 30xz - 40yz)

  =    √(3x)² + (5z)² + (-4y)²-2(3x)(-4y)+2(3x)(5z)-2(-4y)(5z))

  =    √(3x)² + (5z)² + (-4y)²-2(3x)(2y)+2(3x)(5z)-2(-4y)(5z))

   =  √(3x - 4y + 5z)²

  =  |(3x - 4y + 5z)|

Question 6 :

1 + 1/x⁶ + 2/x³

Solution :

 √(1 + 1/x⁶ + 2/x³)

  =   √(1 + 2⋅1⋅(1/x³) + (1/x³)²)

  =   √(1 + (1/x³))²

  =  |1 + (1/x³)|

Hence the square root of given polynomial is 1 + (1/x³).

Question 7 :

(4x² − 9x + 2)(7x² - 13x - 2)(28x² - 3x - 1)

Solution :

  =  √(4x² − 9x + 2) (7x² - 13x - 2) (28x² - 3x - 1)

4x² − 9x + 2  =  (4x - 1)(x - 2)

7x² - 13x - 2  =  (7x + 1)(x - 2)

28x² - 3x - 1  =  (4x - 1) (7x + 1)

  =  √(4x - 1)(x - 2)(7x + 1)(x - 2)(4x - 1) (7x + 1)

  =  |(4x - 1)(x - 2)(7x + 1)|

Question 8 :

(2x² + (17x/6) + 1)((3/2)x² + 4x + 2)((4/3)x² + (11x/3) + 2)

Solution :

  =  √(2x² + (17x/6) + 1)((3/2)x² + 4x + 2)((4/3)x² + (11x/3) + 2)

(2x² + (17x/6) + 1)  =  (12x² + 17x + 6)/6

  =  (3x + 2)(4x + 3)/6

((3/2)x² + 4x + 2)  =  (3x² + 8x + 4)/2

 =  (x + 2)(3x + 2)/2

(4/3)x² + (11x/3) + 2)  =  (4x² + 11x + 6)/3

=  (x + 2)(4x + 3)/3

=  √(3x + 2)(4x + 3)/6 ⋅ (x + 2)(3x + 2)/2 ⋅ (x + 2)(4x + 3)/3

=  |(3x + 2)(4x + 3)(x + 2)|/6

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.