HOW TO FIND THE EQUATION OF ALTITUDE OF A TRIANGLE
Consider ΔABC shown below.
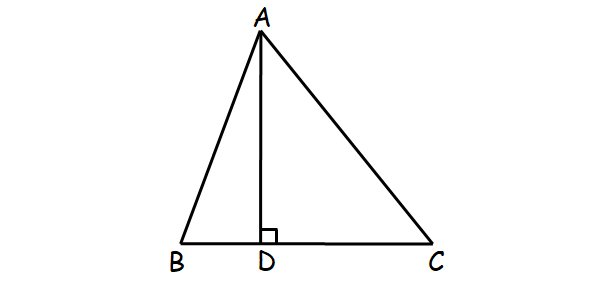
The following steps would be useful to find the equation of the altitude AD.
Step 1 :
Find the slope of BC.
Step 2 :
Since AB and BC are perpendicular,
slope of AD x slope of BC = -1
slope of AD = -1/slope of BC
Step 3 :
Altitude AD is passing through the point A. In slope intercept form equation of a line 'y = mx + b', using the slope of AD and point A, find the y-intercept 'b'.
Step 4 :
Write the equation of the altitude AD using the slope of AD, 'm' and y-intercept 'b'.
Similarly, we can find the equations of altitudes through the vertices B and C.
Example 1 :
A(-3, 0) B(10, -2) and C(12, 3) are the vertices of ΔABC. Find the equations of the altitudes through A, B and C in general form.
Solution :
Equation of the altitude through A :
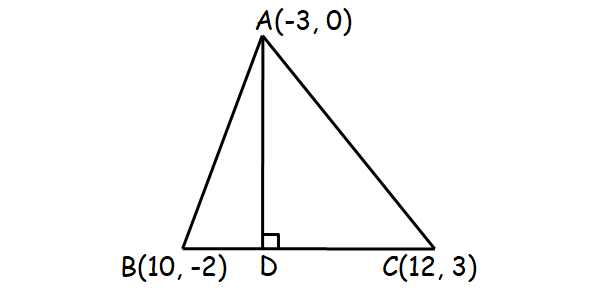
slope of BC = (y2 - y1)/(x2 - x1)
Substitute (x1, y1) = B(10, -2) and (x2, y2) = C(12, 3).
= (3 + 2)/(12 - 10)
slope of BC = 5/2
slope of AD = -1/slope of BC
= -1/(5/2)
= -2/5
Equation of the altitude AD :
y = mx + b
Substitute m = 5/2.
y = (-2/5)x + b ----(1)
Substitute (x, y) = A(-3, 0).
0 = (-2/5)(-3) + b
0 = 6/5 + b
-6/5 = b
(1)----> y = (-2/5)x - 6/5
Multiply each side by 5.
5y = -2x - 6
2x + 5y + 6 = 0
Equation of the altitude through B :
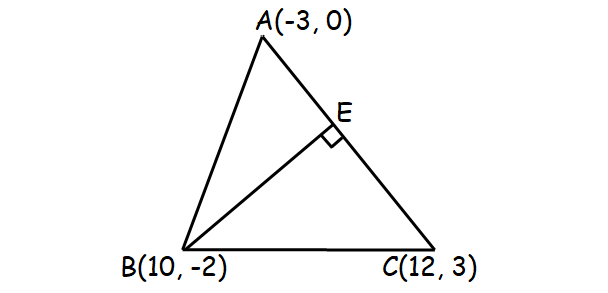
slope of AC = (y2 - y1)/(x2 - x1)
Substitute (x1, y1) = A(-3, .0) and (x2, y2) = C(12, 3).
= (3 - 0)/(12 + 3)
= 3/15
slope of AC = 1/5
slope of BE = -1/slope of AC
= -1/(1/5)
= -5
Equation of the altitude BE :
y = mx + b
Substitute m = -5.
y = -5x + b ----(2)
Substitute (x, y) = B(10, -2).
-2 = -5(10) + b
-2 = -50 + b
48 = b
(2)----> y = -5x + 48
5x + y - 48 = 0
Equation of the altitude through C :
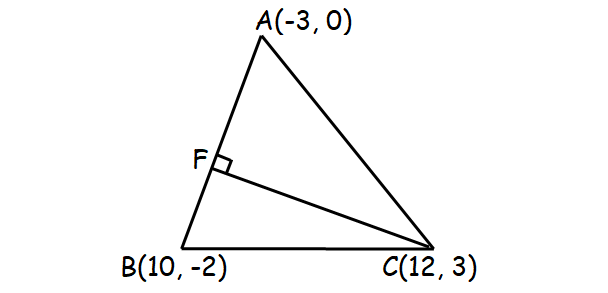
slope of AB = (y2 - y1)/(x2 - x1)
Substitute (x1, y1) = A(-3, 0) and (x2, y2) = B(10, -2).
= (-2 - 0)/(10 + 3)
= -2/13
slope of AB = -2/13
slope of CF = -1/slope of AB
= -1/(-2/13)
= 13/2
Equation of the altitude CF :
y = mx + b
Substitute m = 13/2.
y = (13/2)x + b ----(3)
Substitute (x, y) = C(12, 3).
3 = (13/2)(12) + b
3 = 78 + b
-75 = b
(3)----> y = (13/2)x - 75
Multiply each side by 2.
2y = 13x - 150
-13x + 2y + 150 = 0
13x - 2y - 150 = 0
Example 2 :
Write the equation of the perpendicular bisector that goes through the line segment with end points of (9, 5) and (-7, 13).
Solution :
Perpendicular bisector will divide the opposite side into equal parts and must create the angle measure 90 degree.
Midpoint of the line segment = (x1 + x2)/2, (y1 + y2)/2
= (9 - 7)/2, (5 + 13)/2
= 2/2, 18/2
= (1, 9)
Slope of the perpendicular bisector (or) slope of altitude
= -1/Slope of the line joining the given points
= (y2 - y1)/(x2 - x1)
= (13 - 5)/(-7 - 9)
= 8/-16
= -1/2
Slope of altitude = -1/(-1/2)
= 2
Equation of altitude :
(y - y1) = m(x - x1)
y - 9 = 2(x - 1)
y = 2x - 2 + 9
y = 2x + 7
Example 3 :
Write the equation of the perpendicular bisector that goes through the line segment with end points of (10, -10) and B (2, 2).
Solution :
Midpoint of the line segment = (x1 + x2)/2, (y1 + y2)/2
= (10 + 2)/2, (-10 + 2)/2
= 12/2, -8/2
= (6, -4)
Slope of the perpendicular bisector (or) slope of altitude
= -1/Slope of the line joining the given points
= (y2 - y1)/(x2 - x1)
= (2 - (-10))/(2 - 10)
= (2 + 10)/-8
= -12/8
= -3/2
Slope of altitude = -1/(-3/2)
= 2/3
Equation of altitude :
(y - y1) = m(x - x1)
y + 4 = (2/3)(x - 6)
3y + 12 = 2x - 12
2x - 3y - 12 + 12 = 0
2x - 3y = 0
Example 4 :
Write the equation of the perpendicular bisector that goes through the line segment with end points of (-10, -8) and B (-14 , 8).
Solution :
Midpoint of the line segment = (x1 + x2)/2, (y1 + y2)/2
= (-10 - 14)/2, (-8 + 8)/2
= -24/2, 0/2
= (-12, 0)
Slope of the perpendicular bisector (or) slope of altitude
= -1/Slope of the line joining the given points
= (y2 - y1)/(x2 - x1)
= (8 + 8)/(-14 - 10)
= 16 / (-24)
= -2/3
Slope of altitude = -1/(-2/3)
= -3/2
Equation of altitude :
(y - y1) = m(x - x1)
y - 0 = (-3/2)(x + 12)
2y = -3(x + 12)
2y = -3x + 36
y = (-3/2)x + 36/2
y = (-3/2)x + 18
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
10 Hard SAT Math Questions (Part - 32)
Oct 30, 25 08:57 AM
10 Hard SAT Math Questions (Part - 32) -
10 Hard SAT Math Questions (Part - 31)
Oct 27, 25 10:32 AM
10 Hard SAT Math Questions (Part - 31) -
Time and Work Problems
Oct 20, 25 07:13 AM
Time and Work Problems - Concept - Solved Problems
