HOW TO FIND THE PRODUCT OF TWO MATRICES

Question 1 :

If 

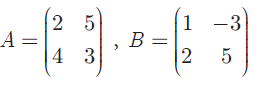
find AB, BA and check if AB = BA? 

Solution :

AB  =  

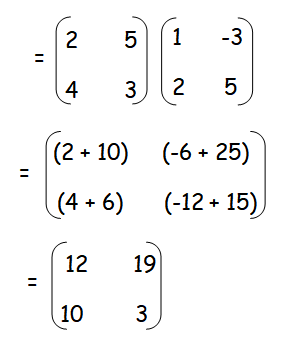
BA  =  

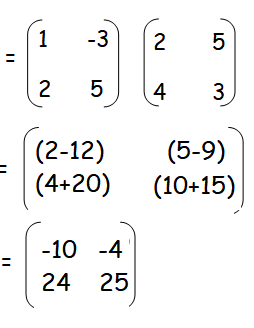
AB and BA are not equal.

Question 2 :

Given that

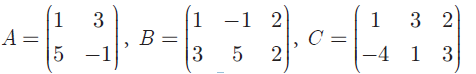
verify that A(B + C) = AB + AC .

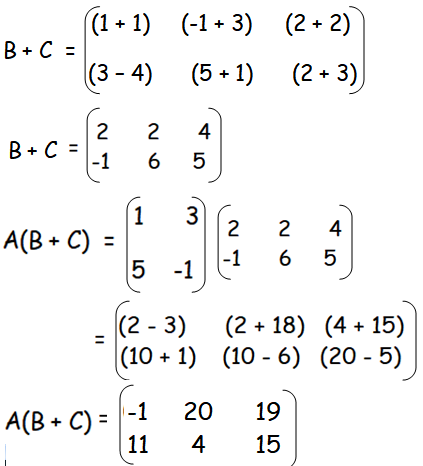
R.H.S :

AB  =

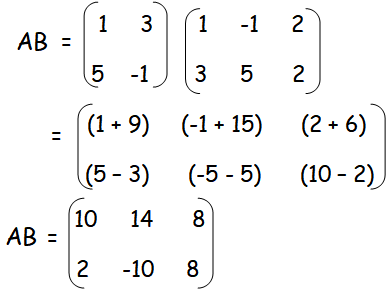
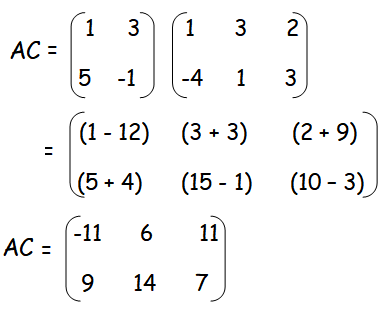
Now, let us add AB and AC

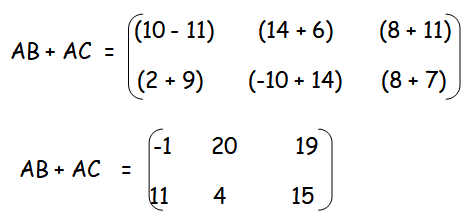
 Hence proved.

Question 3 :

Show that the matrices

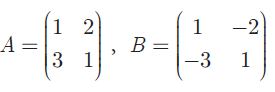
satisfy commutative property AB = BA

Solution :

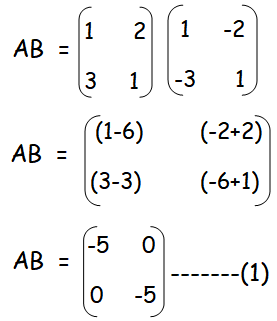
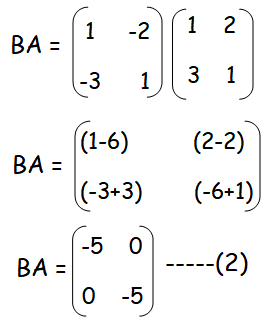
Hence the commutative property is true for the above matrices.

Question 4 :

Show that (i) A(BC) = (AB)C

(ii) (A−B)C = AC −BC (iii) (A−B)^T = A^T −B^T

Solution :

(i) A(BC) = (AB)C

First let us find the product of B and C.

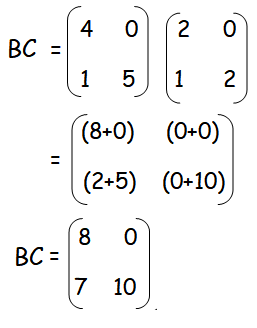
Let us multiply the matrices A and BC.

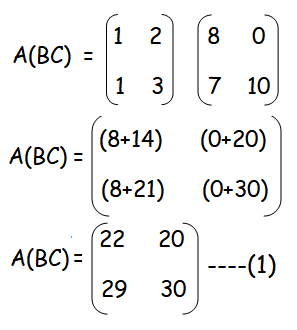
Let us find the product of AB.

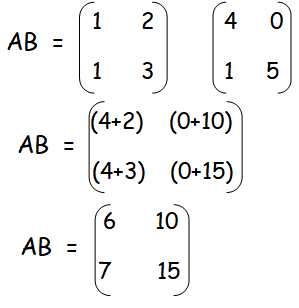
Multiplying the matrices AB and , we get

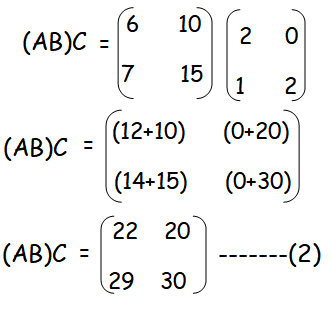
A(BC)  =  (AB)C

Hence it is proved.

(ii) (A−B)C = AC −BC 

Solution :

L.H.S

(A−B) C  =  

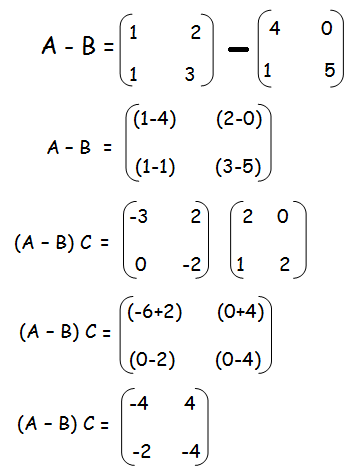
R.H.S :

AC  =  

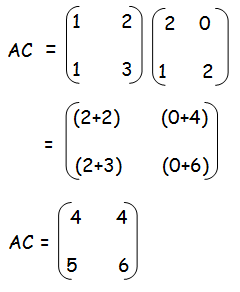
BC  =  

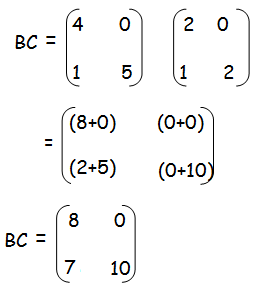
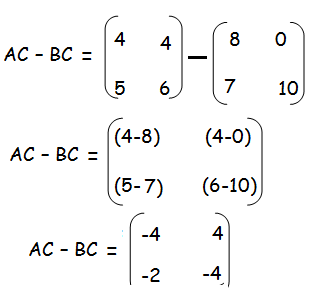
(A−B)C = AC −BC 

(iii) (A−B)^T = A^T −B^T

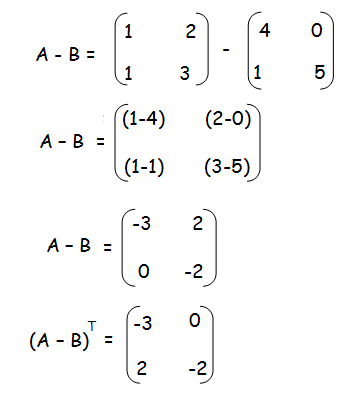
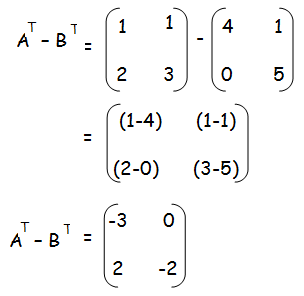
Hence proved.

Question 5 :

If

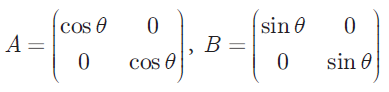
then show that A² +B² = I .

Solution :

A²   =  A x A

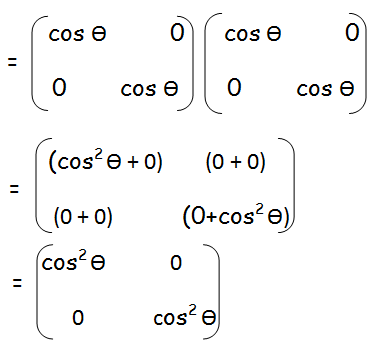
B²  =  B x B

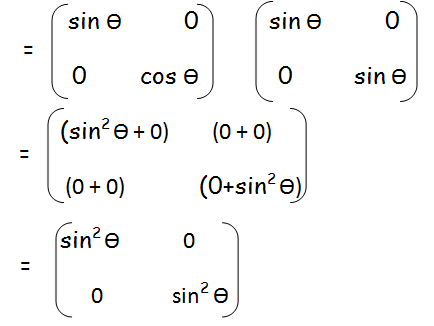
By adding the corresponding terms, we get 

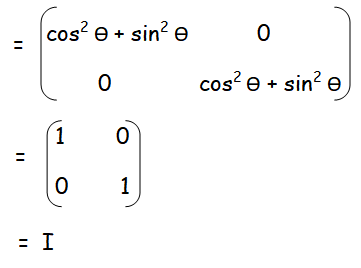
Hence proved.

Question 6 :

If A = 

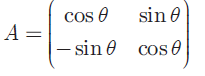
prove that AA^T = I .

Solution :

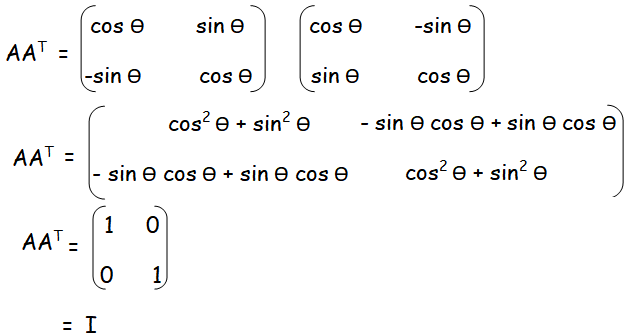
Question 7 :

Verify that A² = I when

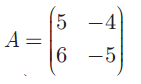
Solution :

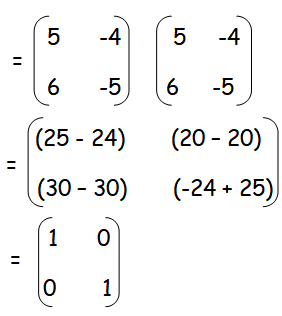
  =  I  (hence proved)

Question 8 :

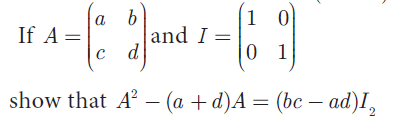
Solution :

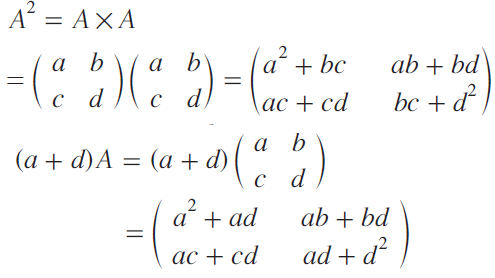
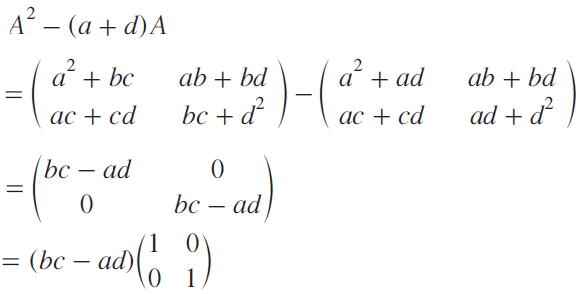
Question 9 :

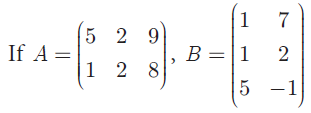
verify that (AB)^T = B^TA^T

Solution :

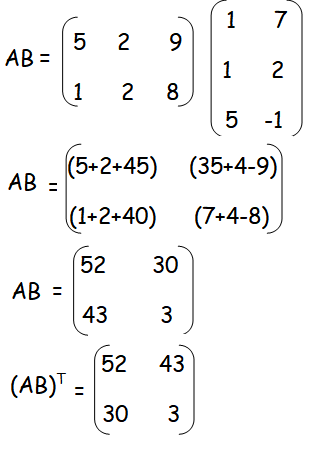
Question 10 :

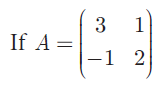
show that A² - 5A + 7I₂  =  0

Solution :

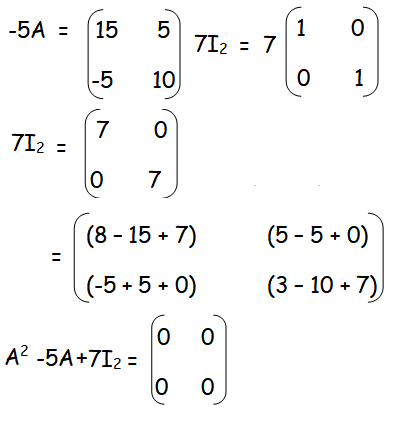
Hence proved.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.