HOW TO GRAPH A PARABOLA IN STANDARD FORM

Example 1 : 

Graph : y = 2x² - 8x + 6

Solution : 

Equation of the parabola is in vertex form : 

y = ax² + bx + c

a = 2, b = -8, and c = 6

Because a > 0, the parabola opens up.

Find and plot the vertex. The x-coordinate is :

x  =  -b/2a

Substitute. 

=  -(-8)/2(2)

=  8/4

=  2

The x-coordinate at the vertex is 2 and axis of symmetry is x = 2. 

The y-coordinate is :

y  =  2(2)² - 8(2) + 6

=  8 - 16 + 6

=  -2

So, the vertex is (2, -2). 

Draw the axis of symmetry x = 2.

Plot two points on one side of the axis of symmetry, such as (1, 0) and (0, 6). Use symmetry to plot two more points, such as (3, 0) and (4, 6).

Draw a parabola through the plotted points.

Example 2 : 

Graph : y = -x² + 6x - 7

Solution : 

Equation of the parabola is in vertex form : 

y = ax² + bx + c

a = -1, b = 6, and c = -7

Because a < 0, the parabola opens up.

Find and plot the vertex. The x-coordinate is :

x  =  -b/2a

Substitute. 

=  -6/2(-1)

=  -6/(-2)

=  3

The x-coordinate at the vertex is 3 and axis of symmetry is x = 3. 

The y-coordinate is :

y = -3² + 6(3) - 7

=  -9 + 18 - 7

=  2

So, the vertex is (3, 2). 

Draw the axis of symmetry x = 3.

Plot two points on one side of the axis of symmetry, such as (2, 1) and (1, -2). Use symmetry to plot two more points, such as (4, 1) and (5, -2).

Draw a parabola through the plotted points.

Example 3 :

Graph : y = x² - 6x + 11

Solution :

Equation of the parabola is in vertex form : 

y = ax² + bx + c

a = 1, b = -6, and c = 11

Because a > 0, the parabola opens up.

Find and plot the vertex. The x-coordinate is :

x  =  -b/2a

Substitute. 

=  -(-6)/2(1)

=  6/2

=  3

The x-coordinate at the vertex is 3 and axis of symmetry is x = 3. 

The y-coordinate is :

y = 3² - 6(3) + 11

=  9 - 18 + 11

=  2

So, the vertex is (3, 2). 

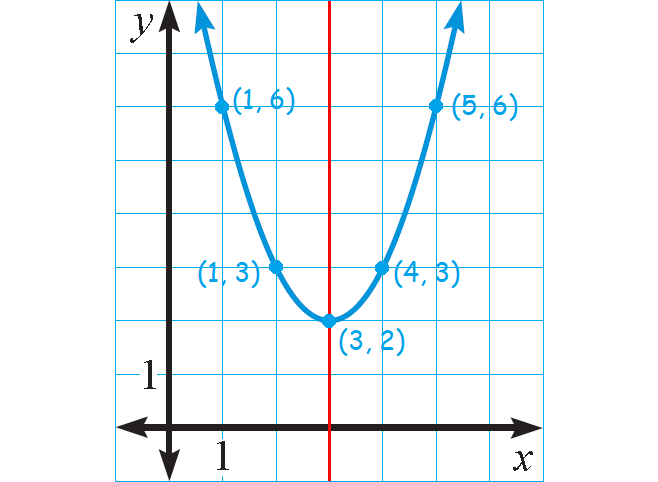
Draw the axis of symmetry x = 3.

Plot two points on one side of the axis of symmetry, such as (1, 3) and (1, 6). Use symmetry to plot two more points, such as (4, 3) and (5, 6).

Draw a parabola through the plotted points.

Example 4 :

Graph : y = x² + 6x + 3

Solution :

Equation of the parabola is in vertex form : 

y = ax² + bx + c

a = 1, b = 6, and c = 3

Because a > 0, the parabola opens up.

Find and plot the vertex. The x-coordinate is :

x  =  -b/2a

Substitute. 

=  -6/2(1)

= -6/2

= -3

The x-coordinate at the vertex is -3 and axis of symmetry is x = -3. 

The y-coordinate is :

y = 3² + 6(3) + 3

=  9 + 18 + 3

=  30

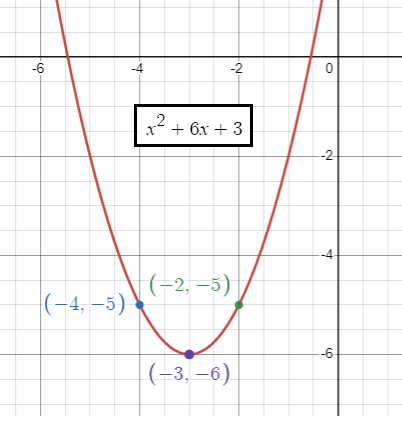
So, the vertex is (-3, 30)

Draw the axis of symmetry x = -3.

The points are (-4, -6) and (-2, -7)

Example 5 :

Graph : y = x² + 4x + 5

Solution :

Equation of the parabola is in vertex form : 

y = ax² + bx + c

a = 1, b = 4, and c = 5

Because a > 0, the parabola opens up.

Find and plot the vertex. The x-coordinate is :

x  =  -b/2a

Substitute. 

=  -4/2(1)

= -4/2

= -2

The x-coordinate at the vertex is -2 and axis of symmetry is x = -2. 

The y-coordinate is :

y = x² + 4x + 5

y =  (-2)² + 4(-2) + 5

= 4 - 8 + 5

= 9 - 8

y = 1

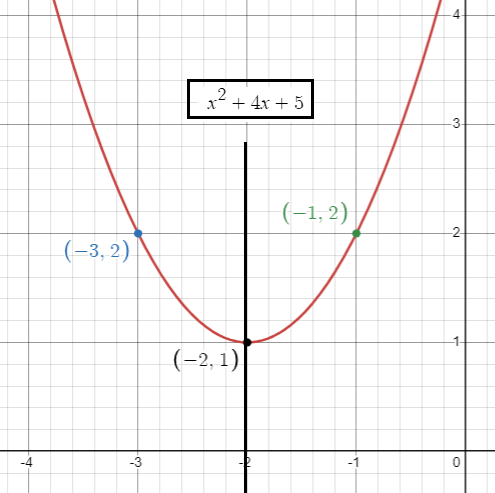
So, the vertex is (-2, 1)

Draw the axis of symmetry x = -2.

The points are (-3, 2) and (-2, 1)

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.