HOW TO SKETCH THE GRAPH AND FIND CONTINUITY OF FUNCTIONS

Question 1 :

Find the points at which f is discontinuous. At which of these points f is continuous from the right, from the left, or neither? Sketch the graph of f.

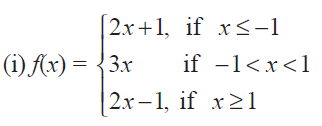
Solution :

First let us check the continuity at the point x  =  -1

lim _{x-> -1-} f(x)  =  lim _{x-> -1-} 2x + 1

By applying the limit, we get

  =  2(-1) + 1

  =  -2 + 1

  =  -1 -----(1)

lim _{x-> -1+} f(x)  =  lim _{x-> -1+} 3x

By applying the limit, we get

  =  3(-1)

  =  -3 -----(2)

lim _{x-> -1-} f(x)  ≠  lim _{x-> -1+}

So, the function is not continuous at x = -1.

Now let us check the continuity at the point x  =  1

lim _{x-> 1-} f(x)  =  lim _{x-> 1-} 3x

By applying the limit, we get

  =  3(1)

  =  3 -----(1)

lim _{x-> -1+} f(x)  =  lim _{x-> -1+} 2x - 1

By applying the limit, we get

  =  2(1) - 1

  =  1 -----(2)

lim _{x-> 1-} f(x)  ≠  lim _{x-> 1+}

So, the function is not continuous at x = 1.

To find at which of these points f is continuous from the right, from the left, or neither, we have to draw the number line.

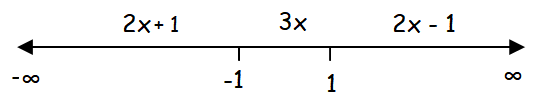
let x₀ ∈ (-∞, -1]

lim _{x-> x0} f(x)  =  lim _{x-> x0} 2x + 1

Applying the limit, we get

  =  2x₀ + 1  ------(1)

f(x₀)  =  2x₀ + 1  ------(2)

(1)  =  (2)

It is continuous in  (-∞, -1].

let x₀ ∈ (-1, -1)

lim _{x-> x0} f(x)  =  lim _{x-> x0} 3x

Applying the limit, we get

  =  3x₀  ------(1)

f(x₀)  =  3x₀  ------(2)

(1)  =  (2)

It is continuous in  (-1, 1).

let x₀ ∈ [1, ∞)

lim _{x-> x0} f(x)  =  lim _{x-> x0} 2x - 1

Applying the limit, we get

  =  2x₀ - 1  ------(1)

f(x₀)  =  2x₀ - 1    ------(2)

(1)  =  (2)

It is continuous in [1, ∞).

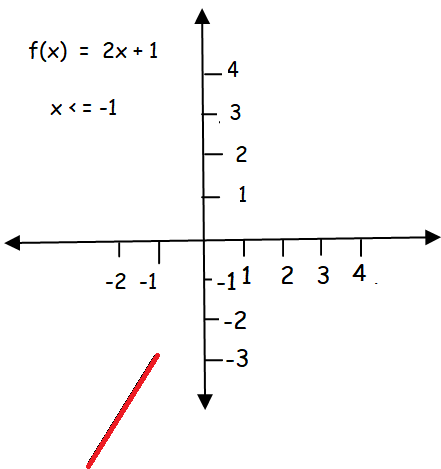
Graph of f(x) = 2x + 1 :

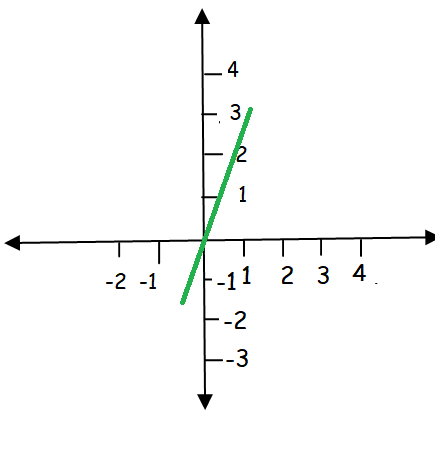
Graph of f(x) = 3x :

-1 < x < 1 

Graph of f(x) = 2x - 1:

x > = 1

Question 2 :

Find the points at which f is discontinuous. At which of these points f is continuous from the right, from the left, or neither? Sketch the graph of f.

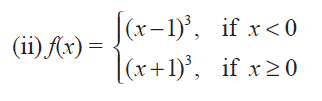
Solution :

First let us check the continuity at the point x  =  0

lim _{x-> 0-} f(x)  =  lim _{x-> 0-} (x - 1)³

By applying the limit, we get

  =  (0 - 1)³

  =  -1  -----(1)

lim _{x-> 0+} f(x)  =  lim _{x-> 0+} (x + 1)³

By applying the limit, we get

  =  ( 0 + 1)³

  =  1 -----(2)

lim _{x-> 0-} f(x)  ≠  lim _{x-> 0+}

So, the function is not continuous at x = 0.

To find at which of these points f is continuous from the right, from the left, or neither, we have to draw the number line.

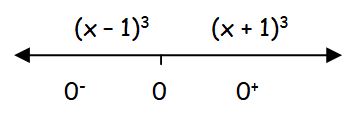
let x₀ ∈ (-∞, 0]

lim _{x-> x0} f(x)  =  lim _{x-> x0} (x - 1)³

Applying the limit, we get

  =  (x₀ - 1)³  ------(1)

f(x₀)  =  (x₀ - 1)³  ------(2)

(1)  =  (2)

It is continuous in  (-∞, 0].

let x₀ ∈ [0, ∞)

lim _{x-> x0} f(x)  =  lim _{x-> x0} (x + 1)³

Applying the limit, we get

  =  (x₀ + 1)³  ------(1)

f(x₀)  =  (x₀ + 1)³  ------(2)

(1)  =  (2)

It is continuous in  [0, ∞).

Graph of f(x) = (x - 1)³

x < 0

Graph of f(x) = (x + 1)³

x >= 0

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.