HOW TO WRITE LINEAR INEQUALITIES IN SLOPE INTERCEPT FORM

Writing linear inequalities from the graph is the reverse process of graphing linear inequalities.

Before learning, how to write linear inequalities, we must be aware of the information about two straight line shown below. 

To find linear inequalities in two variables from graphs, first we have to find two information from the graph. 

(i) Slope 

(ii) y -intercept

By using the above two information we can easily get a linear linear equation in the form y  =  mx + b.

Here m stands for slope and b stands for y-intercept.

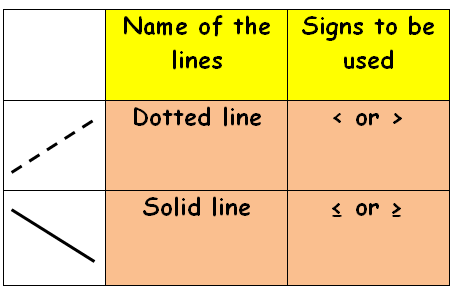
Now we have to notice, whether the given line is solid line or dotted line.

• If the graph contains the dotted line, then we have to use one of the signs < or >.
• If the graph contains the solid line, then we have to use one of the signs ≤  or ≥. 

Example 1 :

Write the inequality for the graph given below.

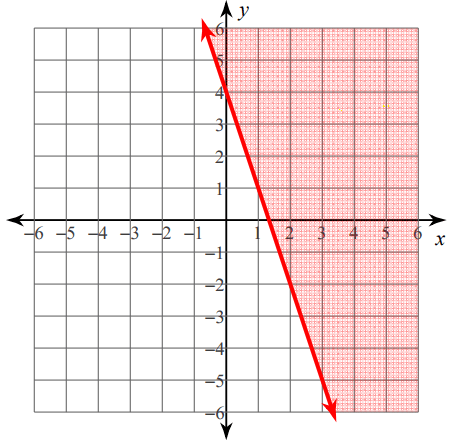
Solution :

From the above graph, first let us find the slope and y-intercept.

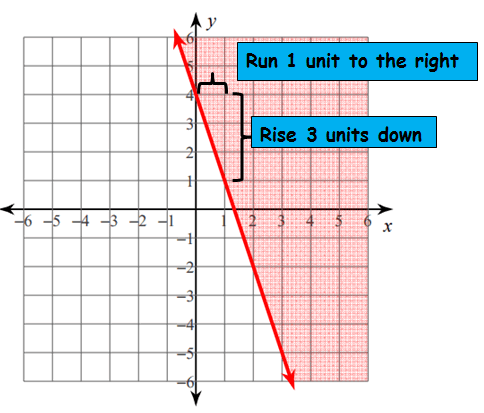
Rise  =  - 3 and Run  =  1

Slope  =  - 3 / 1  =  - 3

y-intercept  =  4

So, the equation of the given line is

y  =  - 3x + 4

But, we need to use inequality which satisfies the shaded region.

Because the graph contains solid line, we have to use one of the signs  ≤  or  ≥.

To find the correct sign, let us take a point from the shaded region.

Take the point (2, 1) and substitute into the equation of the line.

y  =  - 3x + 4

That is, 

1  =  - 3(2) + 4 ?

1  =  - 6 + 4 ?

1  =  - 2 ?

Here, 1 is greater than -2. So, we have to choose the sign ≥ instead of equal sign in the equation y  =  -3x + 4

Therefore, the required inequality is 

y  ≥  - 3x + 4

Example 2 :

Write the inequality for the graph given below.

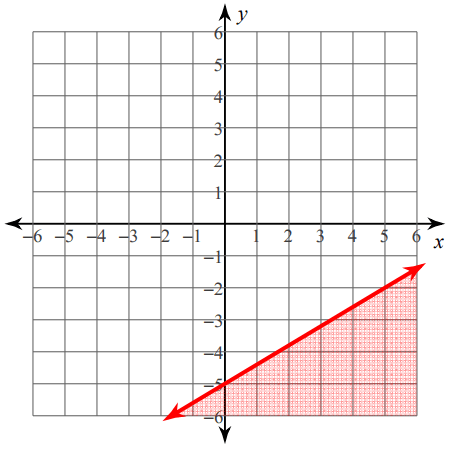
Solution :

From the above graph, first let us find the slope and y-intercept.

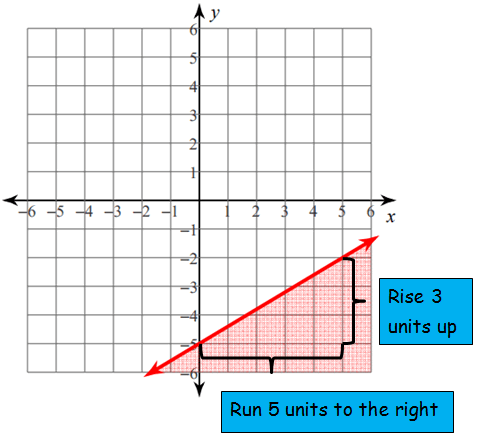
Rise  =  3 and Run  =  5

Slope  =  3 / 5 

y-intercept  =  - 5

So, the equation of the given line is

y = (3/5)x + 4

y  =  3x/5 + 4

But we need to use inequality which satisfies the shaded region.

Because the graph contains solid line, we have to use one of the signs ≤ or ≥.

Take the point (5, -3) and substitute into the equation of the line.

y  =  3x/5 + 4

-3  =  3(5)/5 + 4  ?

-3  =  3 + 4  ?

-3  =  7  ?

Here, (-3) is less than 7. So, we have to choose the sign ≤ instead of equal sign in the equation y  =  3x/5 + 4.

Therefore, the required inequality is 

y  ≤  3x/5 + 4.

Example 3 :

Write the inequality for the graph given below.

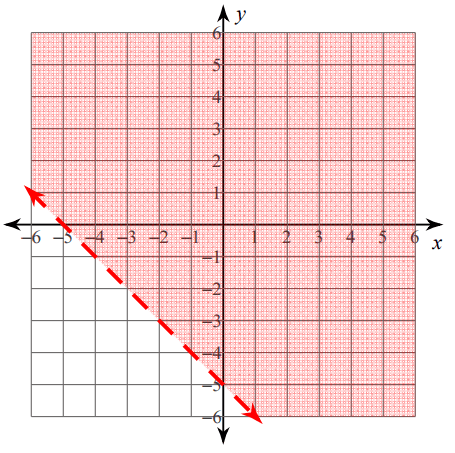
Solution :

From the above graph, first let us find the slope and y-intercept.

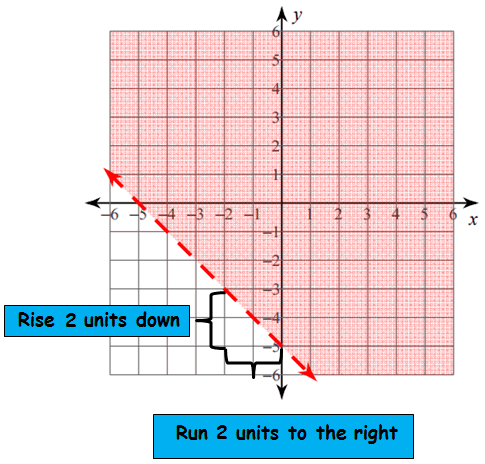
Rise  =  - 2 and Run  =  2

Slope  =  - 2/2  =  - 1

y-intercept  =  - 5

So, the equation of the given line is

y  =  - x - 5

But we need to use inequality which satisfies the shaded region.

Because the graph contains dotted line, we have to use one of the signs < or >.

Take the point (0, 0) and substitute into the equation of the line.

y  =  - x - 5

0  =  0 - 5  ?

0  =  - 5  ?

Here, 0 is greater than (-5). So, we have to choose the sign < instead of equal sign in the equation

y  =  - x - 5

Therefore, the required inequality is 

y  >  -x - 5

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.