IDENTIFY LINEAR AND NONLINEAR FUNCTIONS

Determine whether each table represents a linear or nonlinear function.

Example 1 :

Solution :

In the above table, as x increases by 2, y increases by 3. The rate of change is constant. So, the above table represents a linear function.

Example 2 :

Solution :

In the above table, as x increases by 2, y increases by a greater amount each time. The rate of change is NOT constant. So, the above table represents a nonlinear function.

Example 3 :

Solution :

In the above table, as x increases by 3, y increases by a greater amount each time. The rate of change is NOT constant. So, the above table represents a nonlinear function.

Example 4 :

Solution :

In the above table, as x increases by 4, y decreases by 2 each time. The rate of change is constant. So, the above table represents a linear function.

Determine whether each graph represents a linear or nonlinear function.

Example 5 :

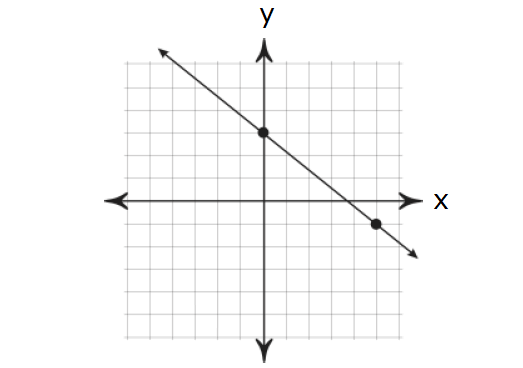
Solution :

The above graph is a straight line. So it represents a linear function.

Example 6 :

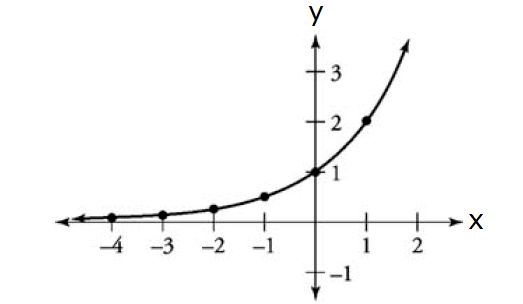
Solution :

The above graph is not a straight line. So it represents a nonlinear function.

Example 7 :

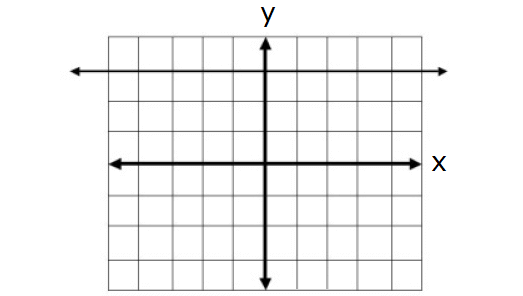
Solution :

The above graph is a straight line. So it represents a linear function.

Example 8 :

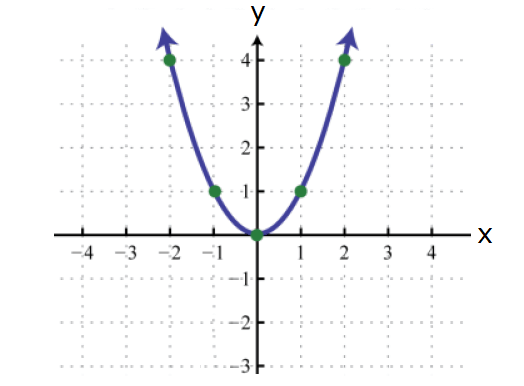
Solution :

The above graph is not a straight line. So it represents a nonlinear function.

Determine whether each equation represents a linear or nonlinear function. Remember that all linear functions can be written in the slope-intercept form, that is y = mx + b.

Example 8 :

y = 5x + 2

Solution :

The above function is in slope-intercept form.

So, it represents a linear function.

Example 9 :

y = 3(x - 4)

Solution :

The above function can be written in slope-intercept form.

y = 3(x - 4)

y = 3x - 12

So, it represents a linear function.

Example 10 :

y = x/2 + 5 

Solution :

The above function can be written in slope-intercept form.

y = x/2 + 5 

y = (1/2)x + 5

y = 0.5x + 5

So, it represents a linear function.

Example 11 :

y = 2/x - 3 

Solution :

The above function cannot be written in slope-intercept form.

So, it represents a nonlinear function.

Example 12 :

y = -5x 

Solution :

y = -5x

y = -5x + 0

The above function is in slope-intercept form.

So, it represents a linear function.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.