IIT JEE Main Mathematics Problems and Solutions

Problem 1 :

If 10^th and 11^th terms of an arithmetic progression are roots of equation 3x² – px + q = 0 and common difference of the arithmetic progression is 3/2. Also the sum of first 11 terms of the arithmetic progression is 88, then q – 2p is

A)  625

B)  474

C)  729

D)  476

Solution :

Problem 2 :

Consider an arithmetic progression of positive integers, whose sum of the first three terms is 54 and the sum of the first twenty terms lies between 1600 and 1800. Then its 11th term is :

A)  90

B)  84

C)  122

D)  108

Solution :

Problem 3 :

Find the product of all solutions of the equation shown above.

Solution :

Problem 4 :

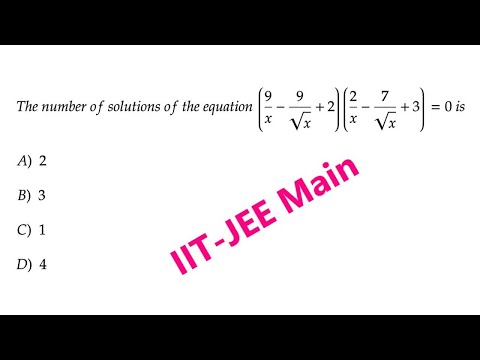
Find the number of solutions for the equation shown above.

Solution :

Problem 5 :

If the set of all a ∊ R, for which the equation 2x² + (a – 5)x + 15 = 3a    has no real root, is the interval (α, β), and X = {x ∊ Z : α < x < β}, then evaluate the following sum.

Solution :

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.