INTERIOR ANGLES OF A POLYGON

Examples 1-4 : All the polygons are regular polygons. In each case, find the measure of each interior angle.

Example 1 :

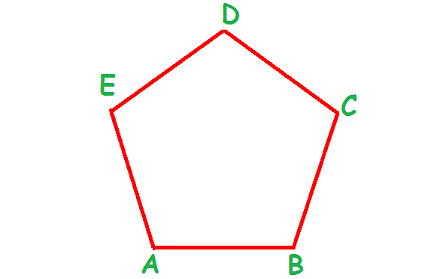
Solution :

The sides of the polygon are AB, BC, CD, DE and EA.

So, the regular polygon above has 5 sides.

Sum of interior angles of polygon = (n - 2) x 180°

= (5 - 2) x 180°

= 3 x 180°

= 540°

Measure of each interior angle :

= 540°/5

= 108°

Example 2 :

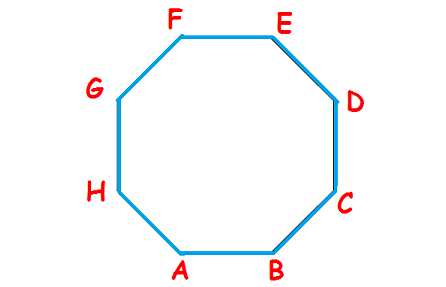
Solution :

The sides of the polygon are AB, BC, CD, DE, EF, FG, GH and HA

So, the regular polygon above has 8 sides.

Sum of interior angles of a polygon = (n - 2) x 180°

= (8 - 2) x 180°

= 6 x 180°

 = 1080°

Measure of each interior angle :

= 1080°/8

= 135°

Example 3 :

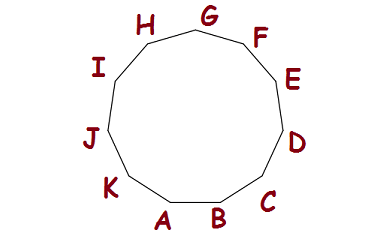
Solution :

The sides of the polygon are AB, BC, CD, DE, EF, FG, GH, HI, IJ, JK abd KA.

So, the regular polygon above has 8 sides.

Sum of interior angles of a polygon = (n - 2) x 180°

= (11 - 2) x 180°

= 9 x 180°

 = 1620°

Measure of each interior angle :

= 1620°/11

= 147° 3'

Example 4 :

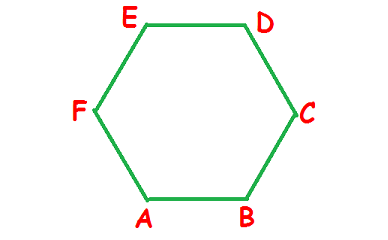
Solution :

The sides of the polygon are AB, BC, CD, DE, EF and FA.

So, the regular polygon above has 6 sides.

Sum of interior angles of a polygon = (n - 2) x 180°

= (6 - 2) x 180°

= 4 x 180°

 = 720°

Measure of each interior angle :

= 720°/6

= 120°

Example 5 :

Find the value of x shown in the polygon shown below.

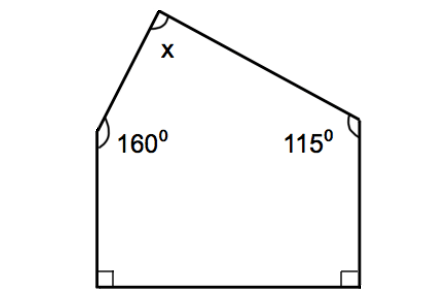
Solution :

The polygon above has 5 sides.

Sum of interior angles of the polygon = (5 - 2) x 180°

x + 115° + 90° + 90° + 160° = 3 x 180°

x + 455° = 540°

Subtract 455° from both sides.

x = 85°

Example 6 :

Find the value of x shown in the polygon shown below.

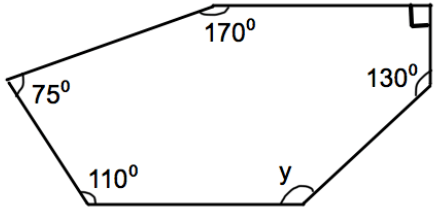
Solution :

The polygon above has 6 sides.

Sum of interior angles of the polygon = (6 - 2) x 180°

y + 130° + 90° + 170° + 75° + 110° = 4 x 180°

y + 575° = 720°

Subtract 575° from both sides.

y = 145°

Example 7 :

Find the value of x in the regular pentagon shown below.

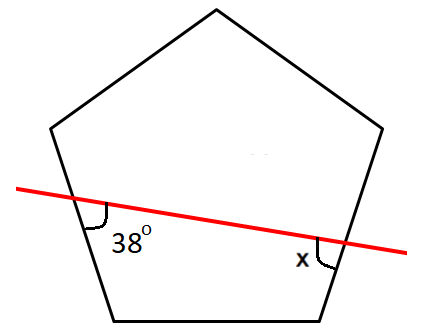
Solution :

Sum of the interior angles of a regular pentagon :

= (5 - 2) x 180°

= 3 x 180°

= 540°

Measure of each interior angle of a regular polygon :

= 540°/5

= 108°

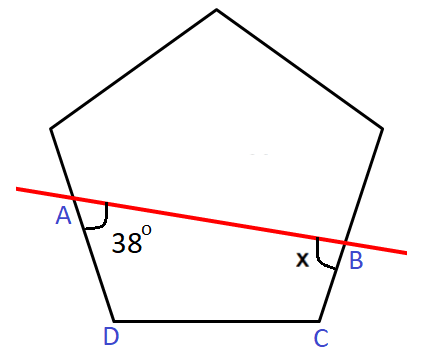
In a regular pentagon, all the interior angles are equal in measure.

m∠C = m∠D = 108°

In the figure above, ABCD is a quadrilateral.

Sum of the interior angles in a quadrilateral = 360°

m∠A + m∠B + m∠C + m∠D = 360°

38° + x + 108° + 108° = 360°

x + 254° = 308°

Subtract 254° from both sides.

x = 54°

Example 8 :

Find the measure of each interior angle of a regular hexagon.

Solution :

Sum of interior angles of an hexagon :

= (6 - 2) x 180°

= 4 x 180°

= 720°

Measure of each interior angle of a regular hexagon :

= 720°/6

= 120°

Example 9 :

If the sum of the interior angles of a polygon is 1260°, How many sides does the polygon have?

Solution :

Sum of interior angles of the polygon = 1260°

(n - 2) x 180° = 1260°

Divide both sides by 180°.

n - 2 = 7

Add 2 to both sides.

n = 9

So, the polygon has 9 sides.

Example 10 :

If the measure of each interior angle of a regular decagon is 2x°, find the value of x.

Solution :

Decagon is a 10-sided polygon.

Sum of interior angles of a decagon : (10 - 2) x 180°

= 8 x 180°

= 1440°

Since decagon is a 10-sided polygon, it will have ten interior angles

Given : The measure each interior angle of a regular decagon is 2x°.

10 x measure of each interior angle = 1440°

10(2x°) = 1440°

20x = 1440

Divide both sides by 20.

x = 72

Example 11 :

If the measure of each interior angle of a regular polygon is 150°, find the number of sides the polygon has.

Solution :

Let n be the number of sides the polygon has.

Sum of interior angles of a polygon : (n - 2) x 180°

n x 150° =  (n - 2) x 180°

n x 150° = n x 180° - 2 x 180°

150n = 180n - 360

Subtract 180n from both sides.

-30n = -360

Divide both sides by -30.

n = 12

So, the polygon has 12 sides.

Example 12 :

If the measure of each interior angle of a regular dodecagon is (2x + 50)°, find the value of x.

Solution :

Dodecagon is a 12-dided polygon

Sum of interior angles of a dodecagon : 

= (12 - 2) x 180°

= 10 x 180°

= 1800°

Measure of each interior angle of a regular dodecagon :

= 1800°/12

= 150°

Given : The measure of each interior angle of a regular dodecagon is (2x + 50)°.

Then,

(2x + 50)° = 150°

2x + 50 = 150

Subtract 50 from both sides.

2x = 100

Divide both sides by 2.

x = 50

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.