INVESTIGATING HORIZONTAL STRETCHES COMPRESSIONS AND REFLECTIONS
Doing stretches, compressions and reflections horizontally are different types of transformations of functions.
Horizontal Stretch
Let f(x) be a function.
In the above function, if we want to do horizontal expansion or compression by a factor of k, at every where of the function, x co-ordinate has to be multiplied by the factor k. Then, we get the new function.
g(x) = f[(1/k)x]
The graph of g(x) = f[(1/k)x] can be obtained by stretching the graph of f(x) horizontally by the factor k.
It can be done by using the rule given below.
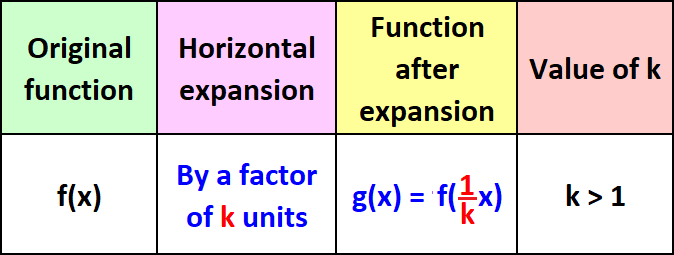
Note :
Point on the original curve : (x, y).
Point on the curve after it is horizontally expanded by the factor of k :
(kx, y)
Horizontal Compression
Let f(x) be a function.
In the above function, if we want to do horizontal compression by a factor of k, at every where of the function, x co-ordinate has to be multiplied by the factor k. Then, we get the new function.
g(x) = f(kx)
The graph of g(x) = f(kx) can be obtained by compressing the graph of f(x) horizontally by the factor k.
It can be done by using the rule given below.
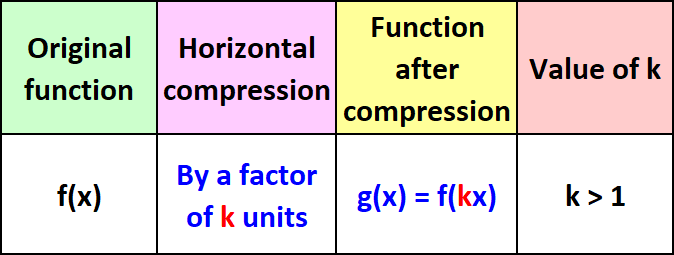
Note :
Point on the original curve : (x, y)
Point on the curve after it is horizontally compressed by the factor of k :
((1/k)x, y)
Horizontal Stretch - Example
Question :
Perform an horizontal stretch by a factor of 2 to the function
y = √x
And also write the formula that gives the requested transformation and draw the graph of both the given function and the transformed function.
Answer :
Step 1 :
Since we do horizontal stretch by the factor 2, we have to replace x by (1/2)x in the given function y = √x.
Step 2 :
So, the formula that gives the requested transformation is
y = √[(1/2)x]
Step 3 :
The graph y = √[(1/2)x] can be obtained by expanding the graph of the function y = √x horizontally by the factor 2.
(x, y) ----> (2x, y)
Step 4 :
The graph of the original function (given function).

Step 5 :
The graph of the function in which horizontal stretch made by the factor 0.5.

How to sketch the graph of the function which is horizontally stretched or compressed ?
Let y = f(x) be the given function and (x, y) by any point on the graph of the function y = f(x).
If we want to perform horizontal stretch in the graph of the function y = f(x) by the factor 0.5, we have to write the point (x, y) as (0.5x, y).
That is, x co-ordinate of each and every point to be multiplied by the factor 0.5.
Therefore, any point on the horizontally stretched graph will be in the form of (0.5x , y).
So, each and every point to be changed according to (0.5x, y) and plot them on the graph.
After having plotted the points, if we connect all the points, we will get the horizontally stretched graph.
The same procedure to be followed for horizontal compression.
Reflections
Let y = f(x) be a function.
The graph of the function f(x) can be reflected about x- axis or y-axis or the line y = x or the line y = -x or the origin using the rules given below.
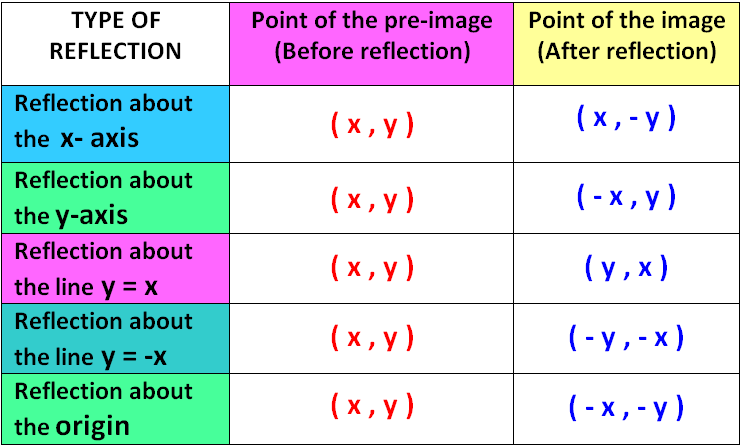
Based on the rules given above, the images and their reflections about x- axis and y-axis and the line y = x are given below.
Reflection about x - axis :
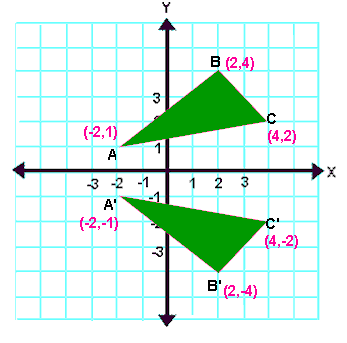
Reflection about y - axis :
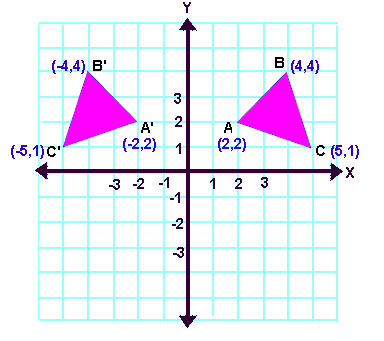
Reflection about the line y = x :
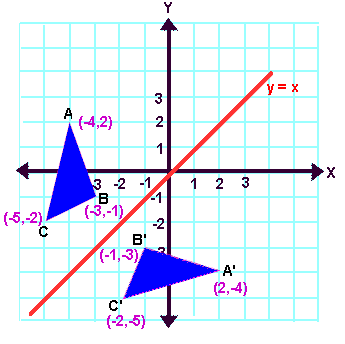
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 146)
Apr 18, 25 06:52 AM
Digital SAT Math Problems and Solutions (Part - 146) -
Logarithmic Derivative Problems and Solutions
Apr 16, 25 09:25 PM
Logarithmic Derivative Problems and Solutions -
Digital SAT Math Problems and Solutions (Part - 145)
Apr 16, 25 12:35 PM
Digital SAT Math Problems and Solutions (Part - 145)

