ISOSCELES EQUILATERAL AND SCALENE TRIANGLES

Isosceles Triangle :

A triangle is isosceles, if it has at least two congruent sides or two congruent angles.

Equilateral Triangle :

A triangle is equilateral, if all the three sides are congruent or all the three angles are congruent.

Scalene Triangle :

A triangle is scalene triangle, if it has three unequal sides.

Base Angles Theorem

If two sides of a triangle are congruent, then the angles opposite them are congruent.

If AB ≅ AC, then ∠B ≅ ∠C

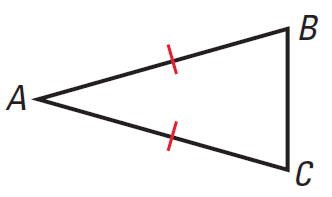Converse of the Base Angles Theorem

If two sides of a triangle are congruent, then the angles opposite them are congruent.

If ∠B ≅ ∠C, then AB ≅ AC

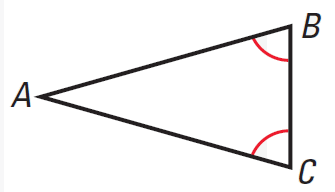Corollaries to the Above Theorems 

Corollary (i) :

If a triangle is equilateral, then it is equiangular.

Corollary (ii) :

If a triangle is equiangular, then it is equilateral.

The above two corollaries have been illustrated in the picture given below.

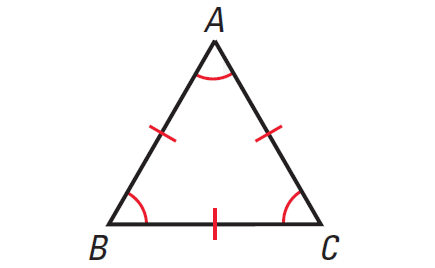
Solving Problems on Isosceles Equilateral and Scalene Triangles

Problem 1 :

Use the diagram of ΔABC shown below to prove the Base Angles Theorem.

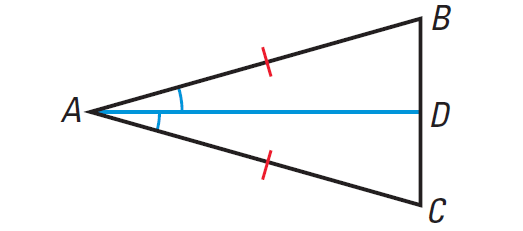
Solution :

Given : In ΔABC, AB ≅ AC.

To prove : ∠B ≅ ∠C.

Proof :

(i)  Draw the bisector of ∠CAB.

(ii)  By construction, ∠CAD ≅ ∠BAD.

(iii)  We are given that AB ≅ AC. Also DA ≅ DA, by the Reflexive Property of Congruence.

(iii) Use the SAS Congruence Postulate to conclude that ΔADB ≅ ΔADC.

(iv)  Because corresponding parts of congruent triangles are congruent, it follows that ∠B ≅ ∠C.

Problem 2 :

In the diagram shown below,

(i) find the value of x

(ii) find the value of y

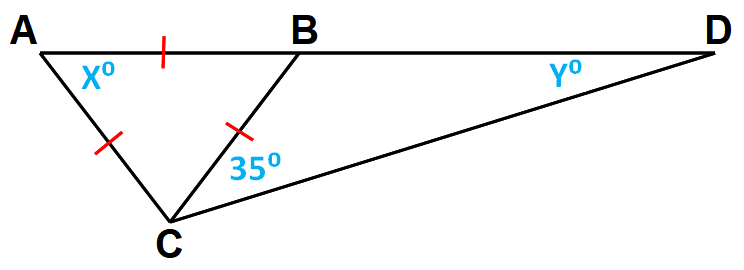
Solution (i) :

In the diagram shown above, x represents the measure of an angle of an equilateral triangle.

From the corollary given above, if a triangle is equilateral, then it is equiangular.

So, the measure of each angle in the equilateral triangle is x.

By the Triangle Sum Theorem, we have

x° + x° + x° = 180°

Simplify. 

3x = 180

Divide both sides by 3 to solve for x.

x = 60

Solution (ii) :

In the diagram shown above, the vertex angle forms a linear pair with a 60° angle, so its measure is 120°.

It has been illustrated in the diagram given below.

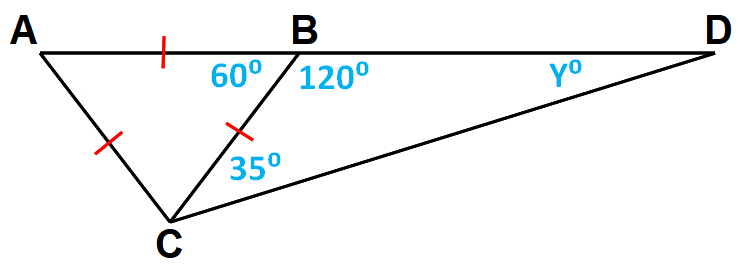
By the Triangle Sum Theorem, we have

120° + 35° + y° = 180°

Simplify.

155° + 2y° = 180°

155 + 2y = 180

Subtract 155 from both sides.

y = 25

Problem 3 :

In the diagram shown below,

(i) find the value of x

(ii) find the value of y

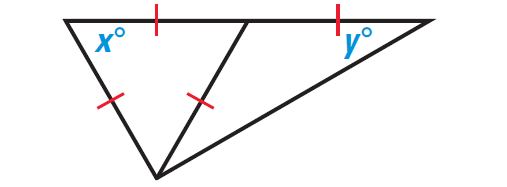
Solution (i) :

In the diagram shown above, x represents the measure of an angle of an equilateral triangle.

From the corollary given above, if a triangle is equilateral, then it is equiangular.

So, the measure of each angle in the equilateral triangle is x.

By the Triangle Sum Theorem, we have

x° + x° + x° = 180°

Simplify.

3x = 180

Divide both sides by 3 to solve for x.

x = 60

Solution (ii) :

In the diagram shown above, y represents the measure of a base angle of an isosceles triangle.

From the Base Angles Theorem, the other base angle has the same measure. The vertex angle forms a linear pair with a 60° angle, so its measure is 120°.

It has been illustrated in the diagram given below.

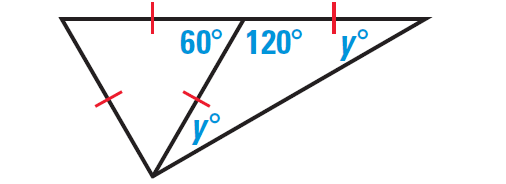
By the Triangle Sum Theorem, we have

120° + y° + y° = 180°

Simplify. 

120 + 2y = 180

Subtract 120 from both sides.

2y = 60

Divide both sides by 2.

y = 30

Problem 4 :

In an acute triangle, the measures of two angles are 50° and 60°. What is the measure of the third angle?

Solution :

In any triangle, the sum of interior angles = 180

The given two angles are 50 and 60. Let x be the third missing angle.

50 + 60 + x = 180

110 + x = 180

x = 180 - 110

x = 70

Problem 5 :

In an obtuse triangle, the measures of two angles are 120° and 10°. What is the measure of the third angle?

Solution :

The given angles are 120 and 10. Let x be the third angle.

120 + 10 + x = 180

130 + x = 180

x = 180 - 130

x = 50

Problem 6 :

One acute angle of a right triangle measures 35°. What is the measure of the other acute angle?

Solution :

Since it is a right triangle, one of the angle measure would be 90 degree. Let x be the missing angle.

90 + 60 + x = 180

150 + x = 180

x = 180 - 150

x = 30

So, the acute angle is 30 degree.

Problem 7 :

Find the angle measures of a triangle if the second angle measures 10 degrees less than twice the first, and the third angle measures 25 degrees more than the second.

Solution :

Let x be the first angle.

Second angle = 2x - 10

Third angle = 2x - 10 + 25

= 2x + 15

Sum of interior angles = 180

x + 2x - 10 + 2x + 15 = 180

5x + 5 = 180

5x = 180 - 5

5x = 175

x = 175/5

x = 35

So, the interior angles are 35, 60 and 85.

Problem 8 :

The measure of the second angle is 15 degrees more than the measure of the first angle. The measure of the third angle is 45 degrees more than the measure of the first angle. Find the measures of the interior angles in the triangle.

Solution :

Let x be the first angle.

Second angle = x + 15

Third angle = x + 45

x + x + 15 + x + 45 = 180

3x + 60 = 180

3x = 180 - 60

3x = 120

x = 120/3

x = 40

So, the interior angles are 40, 55 and 85.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.