LEAST COMMON MULTIPLE WORKSHEET
1) Find the least common multiple of 5 and 7.
2) Find the least common multiple of the following set of numbers using prime factorization method.
6, 9
3) Find the least common multiple of the following set of numbers using prime factorization method.
8, 12
4) Find the least common multiple of the following set of numbers using division method.
16, 24
5) Find the least common multiple of the following set of numbers using division method.
64, 80
6) Find the least common multiple of the following set of numbers using division method.
156, 124
7) Find the least common multiple of the following set of numbers using prime factorization method.
27, 45, 81
8) Find the least common multiple of the following set of numbers using division method.
30, 40, 60
9) Find the least common multiple of the following numbers.
0.75, 0.45
10) Find the least common multiple of the following two fractions.
3/5, 7/10

Answers
1. Answer :
There is no common divisor for 5 and 7.
So, 5 and 7 are relatively prime.
The least common multiple of two numbers that are relatively prime is product of those two numbers.
Hence, the least common multiple of 5 and 7 is
= 5 x 7
= 35
2. Answer :
Write the prime factors of 6 and 9 as shown below.
6 = 2 x 3
9 = 3 x 3
The prime factor 3 appears a maximum of 2 times in the prime factorization of 9.
The prime factor 2 appears only 1 time in the prime factorization of 6.
Hence, the required LCM is
= (3 x 3) x 2
= 9 x 2
= 18
3. Answer :
Write the prime factors of 8 and 12 as shown below.
8 = 2 x 2 x 2
12 = 2 x 2 x 3
The prime factor 2 appears a maximum of 3 times in the prime factorization of 8.
The prime factor 3 appears only 1 time in the prime factorization of 12.
Hence, the required LCM is
= (2 x 2 x 2) x 3
= 8 x 3
= 24
4. Answer :
Start with the smallest prime factor and go on dividing till all the numbers are divided as shown below.
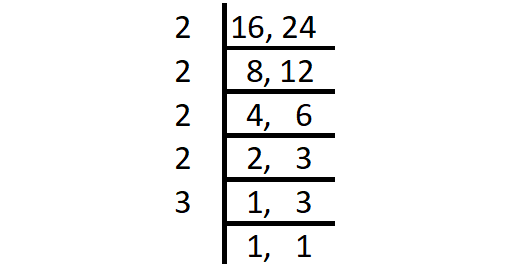
LCM = Product of all prime factors
= 2 x 2 x 2 x 2 x 3
= 48
Thus, the LCM of 16 and 24 is 48.
5. Answer :
Start with the smallest prime factor and go on dividing till all the numbers are divided as shown below.
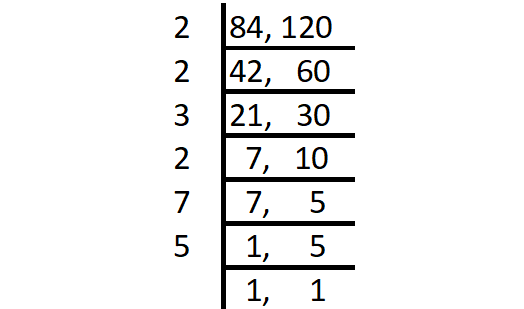
LCM = Product of all prime factors
= 2 x 2 x 3 x 2 x 7 x 5
= 840
Thus, the LCM of 64 and 80 is 840.
6. Answer :
Start with the smallest prime factor and go on dividing till all the numbers are divided as shown below.
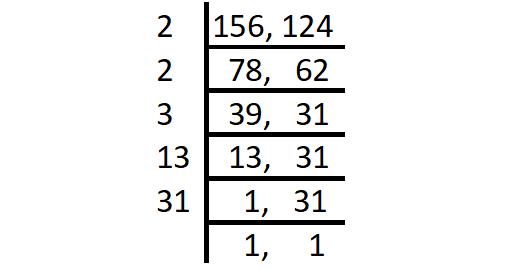
LCM = Product of all prime factors
= 2 x 2 x 3 x 13 x 31
= 4836
Thus, the least common multiple of 156 and 124 is 4836.
7. Answer :
Write the prime factors of 27, 45 and 81 as shown below.
27 = 3 x 3 x 3
45 = 3 x 3 x 5
81 = 3 x 3 x 3 x 3 x 3
The prime factor 3 appears a maximum of 5 times in the prime factorization of 81.
The prime factor 5 appears only 1 time in the prime factorization of 45.
Hence, the required LCM is
= (3 x 3 x 3 x 3 x 3) x 5
= 81 x 5
= 405
8. Answer :
Start with the smallest prime factor and go on dividing till all the numbers are divided as shown below.
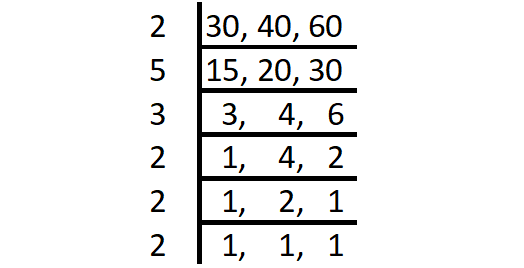
LCM = Product of all prime factors
= 2 x 5 x 3 x 2 x 2
= 120
Thus, the least common multiple of 30, 40 and 60 is 120.
9. Answer :
In the given two numbers 0.75 and 0.45, we find equal number of digits after the decimal point. That is, two digits.
To get rid of the decimal point, we have to multiply each number by 100.
0.75 ⋅ 100 = 75
0.45 ⋅ 100 = 45
Find the least common multiple of 75 and 45 using prime factorization method.
75 = 3 x 5 x 5
45 = 3 x 3 x 5
The prime factor 3 appears a maximum of 2 times in the prime factorization of 45.
The prime factor 5 appears a maximum of 2 times in the prime factorization of 75.
Least common multiple of 75 and 45 is
= (3 x 3) x (5 x 5)
= 9 x 25
= 225
Divide the lcm (225) by 100.
225 / 100 = 2.25
So, the least common multiple of 0.75 and 0.45 is
2.25
10. Answer :
Formula to find the least common multiple of fractions :
= LCM of Numerators / GCF of Denominators
*LCM -----> least common multiple
*GCF -----> greatest common factor
LCM of numerators (3, 7) = 21
GCF of denominators (5, 10) = 5
Least common multiple of 3/5 and 7/10 is
= 21/5
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Permutation Problems with Solutions
May 08, 25 12:28 PM
Permutation Problems with Solutions -
Digital SAT Math Problems and Solutions (Part - 159)
May 08, 25 01:39 AM
Digital SAT Math Problems and Solutions (Part - 159) -
Digital SAT Math Problems and Solutions (Part - 158)
May 06, 25 11:00 AM
Digital SAT Math Problems and Solutions (Part - 158)

