LENGTH OF ARC

Arc is a portion of edge of the circle defined by two end points.

There are two types of arcs. They are minor arc and major arc. 

Minor arc is an arc which has the central angle measure less than 180°. 

Major arc is an arc which has the central angle measure greater than 180°. 

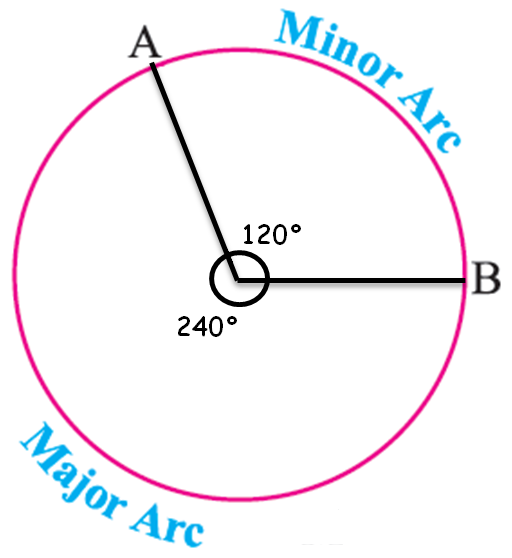
Arc length of a sector is the length of the portion on the circumference of the circle intercepted between the bounding radii and is denoted by l.

Formula to find the length of the arc is 

 l  =  (θ/360°) x 2πr

where

l  = Length of the arc

θ  =  Arc measure or central angle

Formula for area of sector is

=  lr/2 square units

Formula for perimeter of sector is

=  l + 2r units

Solved Examples

Example 1 :

Find the length of the arc whose radius is 42 cm and central angle is 60° (Take π  ≈  3.14 and round your answer to the nearest hundredth, if necessary).

Answer :

Arc length is

=  (θ/360°) ⋅ 2πr

Substitute r  =  42, θ  =  60° and π  ≈  3.14. 

≈  (60°/360°) ⋅ 2 ⋅ (3.14) ⋅ 42

=  (1/6) ⋅ 263.76

=  43.96

So, the length of the arc is about 43.96 cm. 

Example 2 :

Find the length of the arc whose radius is 10.5 cm and central angle is 36° (Take π  ≈  3.14 and round your answer to the nearest hundredth, if necessary).

Answer :

Arc length is

=  (θ/360°) ⋅ 2πr

Substitute r  =  10.5 and θ  =  36° and π  ≈  3.14.

≈  (36°/360°) ⋅ 2 ⋅ (3.14) ⋅ 10.5

 =  (1/10) ⋅ 65.94

=  6.59

So, the length of the arc is about 6.59 cm. 

Example 3 :

Find the length of the arc whose radius is 21 cm and central angle is 120° (Take π  ≈  3.14 and round your answer to the nearest hundredth, if necessary).

Answer :

Arc length is

=  (θ/360°) ⋅ 2πr

Substitute r  =  21 and θ  =  120° and π  ≈  3.14. 

≈  (120°/360°) ⋅ 2 ⋅ (3.14) ⋅ 21

 =  (1/3) ⋅ 131.8

=  43.96

So, the length of the arc is about 43.96 cm.

Example 4 :

Find the arc length whose central angle is 180 degree and circumference of circle is 64 cm.

Solution :

Circumference of circle  =  64 cm

2πr  =  64

Arc length is

l  =  (θ/360°) ⋅ 2πr

Substitute θ  =  180° and 2πr  =  64.

  l  =  (180°/360°) ⋅ 64

l  =  (1/2) ⋅ 64

l  =  32 cm

Example 5 :

Find the area of the sector whose arc length is 20 cm and radius is 7 cm.

Solution :

Area of sector  =  lr/2

Substitute l  =  20 and r  =  7.

Area of sector  =  (20 x 7) / 2

Area of sector  =  70 square units.

Apart from the stuff given above, if you need any other stuff in math, please use our google custom search here. 

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.