LINEAR EQUATIONS WITH UNKNOWN COEFFICIENTS

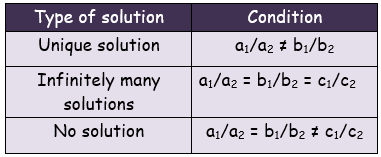
When we solve problems of linear equations with unknown coefficients, we should be aware of number of solutions the system of equations have.

The general form of a pair of linear equations is

a₁x + b₁y + c₁  =  0

a₂x + b₂y + c₂  =  0

(where a₁, a₂, b₁, b₂, c₁, and c₂ are real numbers)

Example 1 :

For what value of c will system of equations below have no solution ?

cx – 2y  =  6

3x + 4y  =  4

Solution :

By writing the given equations a₁x + b₁y + c₁  =  0 and a₂x + b₂y + c₂  =  0 in the form, we get

cx – 2y – 6  =  0

3x + 4y – 4  =  0

Since the given equations have no solution,

a₁/a₂  =  b₁/b₂ ≠ c₁/c₂

Here a₁  =  c, a₂  =  3, b₁  =  -2, and b₂  =  4

c/3  =  -2/4

c  =  3(-1/2)

c  =  -3/2

So, the value of c is -3/2

Example 2 :

For what value of b will system of equations below have infinitely many solutions ?

-2x + y  =  4

5x - by  =  -10

Solution :

-2x + y – 4  =  0

5x – by + 10  =  0

Since the given equations have infinitely many solutions,

a₁/a₂  =  b₁/b₂  =  c₁/c₂

Here a₁  =  -2, a₂  =  5, and b₁  =  1, b₂  =  -b

-2/5  =  1/-b

2b  =  5

b  =  5/2

So, the value of b is 5/2

Example 3 :

ax - y  =  0

x - by  =  1

In the system of equations above, a and b are constants and x and y are variables. If the system of equation above has no solution, what is the value of a . b ?

Solution :

ax - y + 0  =  0

x – by - 1  =  0

Since the given equations have no solution,

a₁/a₂  =  b₁/b₂ ≠ c₁/c₂

Here a₁  =  a, a₂  =  1, b₁  =  -1, and b₂  =  -b

a/1  =  -1/-b

-ab  =  -1

a.b  =  1

So, the value of a.b is 1

Example 4 :

2x - ky  =  14

5x - 2y  =  5

In the system of equations above, k is a constants and x and y are variables. For what value of k will the system of equations have no solution ?

Solution :

2x - ky - 14  =  0

5x – 2y - 5  =  0

Since the given equations have no solution,

a₁/a₂  =  b₁/b₂ ≠ c₁/c₂

Here a₁  =  2, a₂  =  5, b₁  =  -k, and b₂  =  -2

2/5  =  -k/-2

-4  =  -5k

k  =  4/5

So, the value of k is 4/5

Example 5 :

2x – 1/2y  =  15

ax – 1/3y  =  10

In the system of equations above, a is a constants and x and y are variables. For what value of a will the system of equations infinitely many solutions ?

Solution :

2x – 1/2y - 15  =  0

ax – 1/3y - 10  =  0

Since the given equations have infinitely many solutions,

a₁/a₂  =  b₁/b₂  =  c₁/c₂

Here a₁  =  2, a₂  =  a, b₁  =  -1/2, and b₂  =  -1/3

2/a  =  (-1/2)/(-1/3)

-2/3  =  -a/2

4  =  3a

a  =  4/3

So, the value of a is 4/3

Example 6 :

ax + 4y  =  14

5x + 7y  =  8

In the system of equations above, a is a constants and x and y are variables. If the system no solution, what is the value of a ?

Solution :

ax + 4y - 14  =  0

5x + 7y - 8  =  0

Since the given equations have no solution,

a₁/a₂  =  b₁/b₂ ≠ c₁/c₂

Here a₁  =  a, a₂  =  5, b₁  =  4, and b₂  =  7

a/5  =  4/7

7a  =  20

a  =  20/7

So, the value of a is 20/7

Example 7 :

ax + 1/2y  =  16

4x + 3y  =  8

In the system of equations above, a is a constants and x and y are variables. If the system no solution, what is the value of a ?

Solution :

ax + 1/2y - 16  =  0

4x + 3y - 8  =  0

Since the given equations have no solution,

a₁/a₂  =  b₁/b₂ ≠ c₁/c₂

Here a₁  =  a, a₂  =  4, b₁  =  1/2, and b₂  =  3

a/4  =  (1/2)/3

3a  =  2

a  =  2/3

So, the value of a is 2/3

Example 8 :

3x + ky  =  8

x + 4y  =  -1

If(x, y) is a solution to the system of equations above and k is a constant, what is y in terms of k ?

Solution :

3x + ky  =  8 -----(1)

x + 4y  =  -1 -----(2)

Since the given equations (x, y) is a solution,

We are using the elimination method.

Subtract (1) – 3(2), we get

3x + ky – 3x - 12y  =  8 – 3

ky - 12y  =  5

(k - 12)y  =  5

y  =  5/(k – 12)

So, the answer is 5/(k – 12)

Example 9 :

5x + 16y  =  36

cx + dy  =  9

The system of equations above, where c and d are constants, has infinitely many solutions. what is the value of cd ?

Solution :

5x + 16y – 36  =  0

cx + dy – 9  =  0

Since the given equations have infinitely many solutions,

a₁/a₂  =  b₁/b₂  =  c₁/c₂

Here a₁  =  5, a₂  =  c, b₁  =  16, b₂  =  d, c₁  =  -36 and c₂  =  -9

5/c  =  16/d  =  -36/-9

5d  =  16c  =  4

5d  =  4 and 16c  =  4

d  =  4/5 and c  =  1/4

So, the value of c and d is 1/4 and 4/5

Example 10 :

0.3x – 0.7y  =  1

kx – 2.8y  =  3

In the system of equations above, k is a constant. If the system no solution, what is the value of k ?

Solution :

0.3x – 0.7y – 1  =  0

kx – 2.8y – 3  =  0

Since the given equations have no solution,

a₁/a₂  =  b₁/b₂ ≠ c₁/c₂

Here a₁  =  0.3, a₂  =  k, b₁  =  -0.7, and b₂  =  -2.8

0.3/k  =  -0.7/-2.8

(0.3 × -2.8)  =  -0.7k

-0.84  =  -0.7k

k  =  1.2

So, the value of k is 1.2.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.