LINEAR INEQUALITIES WORKSHEET

Problem 1 :

(7, 3) ; y < x - 1

Problem 2 :

(4, 5) ; y > 3x + 2

Problem 3 :

y < 3x + 4

Problem 4 :

3x + 2y ≥ 6

Problem 5 :

Problem 6 :

Problem 7 :

Problem 8 :

5x - 2 ≥ 4 + 3x

Problem 9 :

3x - 2 < 4 + 4x

Problem 10 :

5 - x > -7

Problem 11 :

2 - 3x ≤ 14

Problem 12 :

(2x - 3)/4 + 9 ≥ 3 + 4x/3

Problem 13 :

Lily can spend at most $7.50 on vegetables for a party. Broccoli costs $1.25 per bunch and carrots cost $0.75 per package.

A. Write a linear inequality to describe the situation.

B. Graph the solutions.

C. Give two combinations of vegetables that Sarah can buy.

1. Answer :

Substitute 7 for x and 3 for y in the given inequality.

3 < 7 - 1

3 < 4 ✓

(7, 3) is a solution.

2. Answer :

Substitute 4 for x and 5 for y in the given inequality.

5 > 3(4) + 2

5 > 12 + 2

 5 > 14 ✗

(4, 5) is not a solution.

3. Answer :

Step 1 : 

The inequality is already solved for y.

Step 2 : 

Graph the boundary line y = 3x + 4. Use a dashed line for <.

Step 3 : 

The inequality is <, so shade below the line.

4. Answer :

Step 1 :

Solve the inequality for y.

3x + 2y  ≥  6

2y  ≥  6 - 3x

y  ≥  3 - (3/2)x

y  ≥  -(3/2)x + 3

Step 2 :

Graph the boundary line y = -(3/2)x + 3. Use a solid line for ≥.

Step 3 :

The inequality is ≥, so shade above the line.

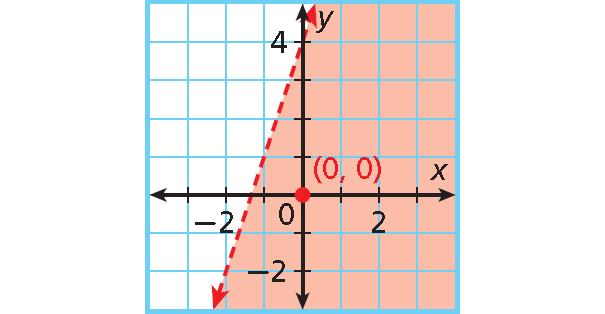
5. Answer :

y-intercept : 2; slope : - 1/3.

Write an equation in slope-intercept form.

y = mx + b ---> y = -(1/3)x + 2

The graph is shaded below a dashed boundary line.

Replace = with < to write the inequality y < -(1/3)x + 2.

6. Answer :

y-intercept : -2; slope : 5.

Write an equation in slope-intercept form.

y = mx + b ---> y = 5x + (-2)

The graph is shaded above a solid boundary line.

Replace = with ≥ to write the inequality y ≥ 5x - 2.

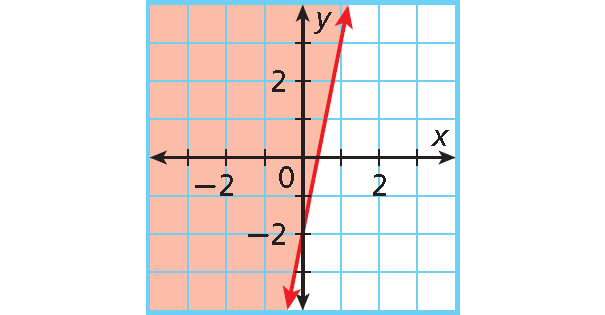
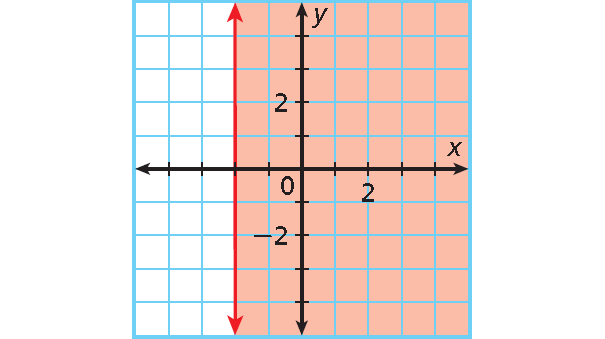
7. Answer :

y-intercept : none; slope : undefined.

The graph is a vertical line at x = -2.

The graph is shaded on the right side of a solid boundary line.

Replace = with ≥ to write the inequality x ≥ - 2.

8. Answer :

5x - 2 ≥ 4 + 3x

Subtract 3x from both sides.

2x - 2 ≥ 4

Add 2 to both sides.

2x ≥ 6

Divide both sides by 2.

x ≥ 3

9. Answer :

3x - 2 < 4 + 4x

Subtract 4x from both sides.

-x - 2 < 4

Add 2 to both sides.

-x < 6

Multiply both sides by -1.

x > -6

10. Answer :

5 - x > -7

Subtract 5 from both sides.

-x > -12

Multiply both sides by -1.

x < 12

11. Answer :

2 - 3x ≤ 14

Subtract 2 from both sides.

-3x ≤ 12

Divide both sides by -3.

x ≥ -4

12. Answer :

(2x - 3)/4 + 9 ≥ 3 + 4x/3

Least common multiple of the denominators 3 and 4 is 12. Multiply both sides of the equation by 12 to get rid of the denominators. 

12[(2x - 3)/4 + 9 ≥ 12[3 + 4x/3]

12(2x - 3)/4 + 12(9) ≥ 12(3) + 12(4x/3)

3(2x - 3) + 108 ≥ 36 + 4(4x)

6x - 9 + 108 ≥ 36 + 16x

6x + 99 ≥ 36 + 16x

Subtract 16x from both sides.

-10x + 99 ≥ 36

Subtract 99 from both sides.

-10x ≥ -63

Divide both sides by 10.

x ≤ 6.3

13. Answer :

A. Write a linear inequality to describe the situation.

Let x represent the number of bunches of broccoli and let y represent the number of packages of carrots.

Write an inequality. Use ≤ for “at most.”

Cost of broccoli plus cost of carrots is at most $7.50

1.25x + 0.75y ≤ $7.50

Solve the inequality for y.

1.25x + 0.75y ≤ 7.50

100(1.25x + 0.75y) ≤ 100(7.50)

125x + 75y ≤ 750

75y ≤ 750 - 125x

75y/75 ≤ 750/75 - 125x/75

y ≤ 10 - (5/3)x

y ≤ -(5/3)x + 10

B. Write a linear inequality to describe the situation.

Step 1 :

Because Lily cannot buy a negative amount of vegetables, the system is graphed only in Quadrant I. Graph the boundary line y = -(5/3)x + 10. Use a solid line for ≤.

Step 2 :

Shade below the line. Lily must buy whole numbers of bunches or packages. All points on or below the line with whole-number coordinates represent combinations of broccoli and carrots that Lily can buy.

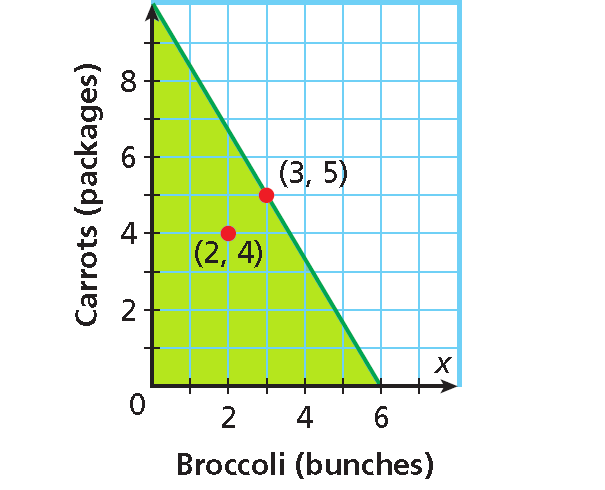
C. Give two combinations of vegetables that Lily can buy.

Two different combinations that Lily could buy for $7.50 or less are 2 bunches of broccoli and 4 packages of carrots, or 3 bunches of broccoli and 5 packages of carrots.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.