LOGARITHM QUESTIONS AND ANSWERS CLASS 11

(1)  Let b > 0 and b ≠ 1. Express y = b^x in logarithmic form. Also state the domain and range of the logarithmic function.            Solution

(2)  Compute log₉ 27 − log₂₇ 9              Solution

(3)  Solve log₈ x + log₄ x + log₂ x = 11         Solution

(4)  Solve log₄ 2^8x = 2^log₂ ⁸         Solution

(5)  If a² + b² = 7ab, show that

log (a + b) / 3  =  1/2(log a + log b)         Solution

(6)  Prove that log (a²/bc) + log (b²/ac) + log (c²/ab)  =  0

Solution

(7)   Prove that

log 2 + 16 log (16/15) + 12 log (25/24) + 7 log(81/80)  =  1

Solution

(8)  Prove that 

Solution

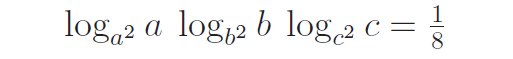
(9)  Prove log a + log a² + log a³ + · · · + log aⁿ = (n(n+1)/2) log a.      Solution

(10)  If log x/(y - z)  =  log y/(z - x)  =  log z/(x - y), then prove that xyz  =  1.            Solution

(11)  Solve for x : log₂ x − 3log _1/2 x  =  6   Solution

(12)  Solve for x : log_5-x (x² − 6x + 65)  =  2      Solution

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.