MATRIX AND DETERMINANTS EXAMPLE PROBLEMS

Question 1 :

Prove that

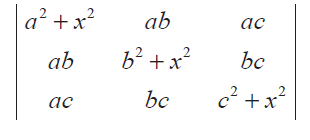
Solution :

First let us factor "a" from the 1^st row, "b" from the 2^nd row and c from the 3^rd row.

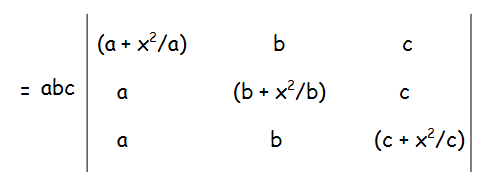
Now we have to multiply column 1, 2 and  by a, b and c respectively.

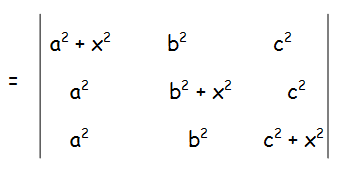
Let us subtract 2^nd row from 1^st row and subtract 3^rd row from the 2^nd row.

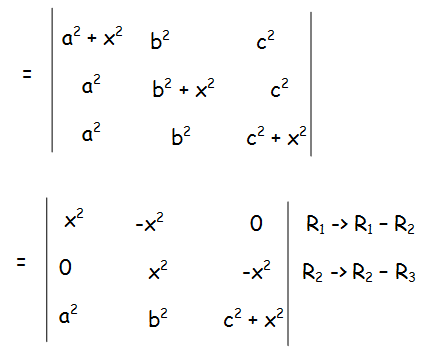
  =  x²(c²x² + x⁴ + b²x²) + x²(0 + a² x²) 

  =  x²(c²x² + x⁴ + b²x²) + x²(0 + a² x²) 

  =  x⁴ c² + x⁶ + b²x⁴ + a²x⁴

  =  x⁴(c² + x² + b²+ a²)

Hence it is divisible by x⁴.

Question 2 :

If a, b, c are all positive, and are p^th, q^th and r^th terms of a G.P., show that

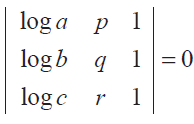
Solution :

n^th term of G.P

a_n  =  ar^n-1

a = p^th term of G.P  =  ar^p-1   --(1)

b = q^th term of G.P  =  ar^q-1   --(2)

c = r^th term of G.P  =  ar^r-1   --(3)

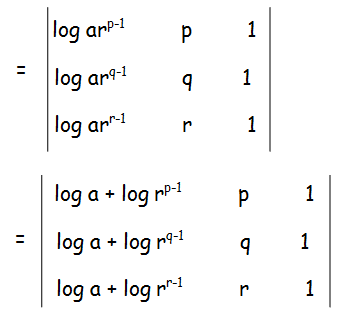
By using properties of determinants, let us write them as sum of two determinants.

In the second determinant, let us add 1^st and 3^rd column.

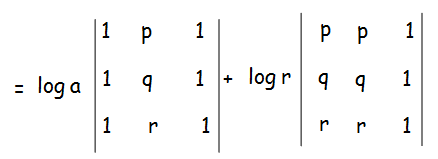
In the first determinant column 1 and  are identical. In the second determinant column 1 and 2 are identical.

  =   log a (0) + log r (0)

  =  0

Hence it is proved.

Question 3 :

Find the value of 

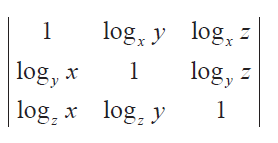
if x, y and z ≠ 1

Solution :

By expanding the above determinant, we get

  =  1[1 - log_zy log_yz] - log_xy[log_yx - log_zx log_yz] + log_xz[log_yxlog_zy - log_zx]

By using the properties of logarithms

  =  [1 - log_yy] - log_xylog_yx + log_xylog_zx log_yz + log_xzlog_yxlog_zy - log_xzlog_zx

  =  [1 - log_yy] - log_yy + log_zylog_yz + log_yzlog_zy - log_zz

  =  [1 - 1] - 1 + log_yy + log_zz - 1

  =  - 1 + 1 + 1 - 1

  =  0

Hence the answer is 0.

Question 4 :

If A =

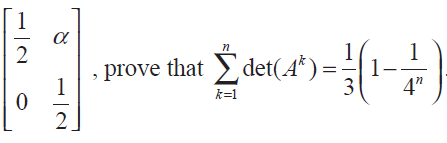
Solution :

|A|  =  1/4

det (A^k)  =  (1/4)^k

By finding the sum, we get

  =  (1/4) + (1/4)² + (1/4)³ + ..................n terms

Sum of geometric series

S_n  =   a(rⁿ - 1) / (r - 1)

a  =  1/4,  r  =  1/4

S_n  =   (1/4)((1/4)ⁿ - 1) / ((1/4) - 1)

  =   (1/4)((1/4)ⁿ - 1) / (-3/4)

  =  (-1/3) ((1/4)ⁿ - 1)

  =  (1/3)(1 - (1/4)ⁿ)

Hence it is proved.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.