MEAN ABSOLUTE DEVIATION

A measure of variability is a single number used to describe the spread of a data set. It can also be called a measure of spread. One measure of variability is the mean absolute deviation (MAD), which is the mean of the distances between the data values and the mean of the data set.

Example : 

The data represent the height, in feet, of various buildings. Find the mean absolute deviation for each data set.

A : 60, 58, 54, 56, 63, 65, 62, 59, 56, 58

B : 46, 47, 56, 48, 46, 52, 57, 52, 45)

Solution : 

Step 1 :

60, 58, 54, 56, 63, 65, 62, 59, 56, 58

Calculate the mean. Round to the nearest whole number.

Mean is 

=  (60 + 58 + 54 + 56 + 63 + 65 + 62 + 59 + 56 + 58) / 10

=  591 / 10

≈  59

Complete the table.

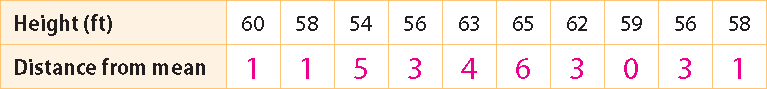
Calculate the MAD by finding the mean of the values in the second row of the table. Round to the nearest whole number.

=  (1 + 1 + 5 + 3 + 4 + 6 + 3 + 0 + 3 + 1) / 10

=  27 / 10

=  2.7

≈  3

Step 2 :

46, 47, 56, 48, 46, 52, 57, 52, 45

Calculate the mean. Round to the nearest whole number.

Mean is 

=  (46 + 47 + 56 + 48 + 46 + 52 + 57 + 52 + 45) / 10

=  449 / 9

≈  50

Complete the table.

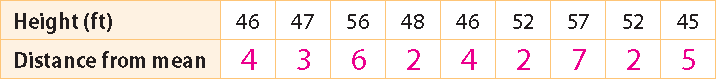
Calculate the MAD by finding the mean of the values in the second row of the table. Round to the nearest whole number.

=  (4 + 3 + 6 + 2 + 4 + 2 + 7 + 2 + 5) / 9

=  35 / 10

=  3.5

≈  4

Reflect

1. Compare the MADs. How do the MADs describe the distribution of the heights in each group ?

The MAD in part B is greater; the heights in part B are spread out more from the mean than the heights in part A.

2. What is the difference between a measure of center and a measure of variability ?

A measure of center is a number that indicates where the “middle” or center of a data set is, while a measure of variability is a number that indicates how much the data are spread out from the center of the data.

Coefficient of Mean Absolute Deviation

A relative measure of dispersion applying MAD is given by coefficient of mean deviation. 

Formula to find coefficient of mean deviation is given by 

=  (Mean deviation about A / A) x 100

Properties of Mean Absolute Deviation

1)  MAD takes its minimum value when the deviations are taken from the median.

2)  MAD remains unchanged due to a change of origin but changes in the same ratio due to a change in scale

That is,  if y = a + bx, a and b being constants, then

MAD of y  =  |b| × MAD of x

3)  It is rigidly defined

4) It is based on all the observations and not much affected by sampling fluctuations.

5)  It is difficult to comprehend and its computation

6) Furthermore, unlike SD, MAD does not possess mathematical properties.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.