MODELING THE VOLUME OF A CONE

A cone is a three-dimensional figure that has one vertex and one circular base as shown in the figure given below.

Let us see how the volume of a cone can be modeled. 

To explore the volume of a cone, Alexa does an experiment with a cone and a cylinder that have congruent bases and heights. She fills the cone with popcorn kernels and then pours the kernels into the cylinder as shown in the figure.

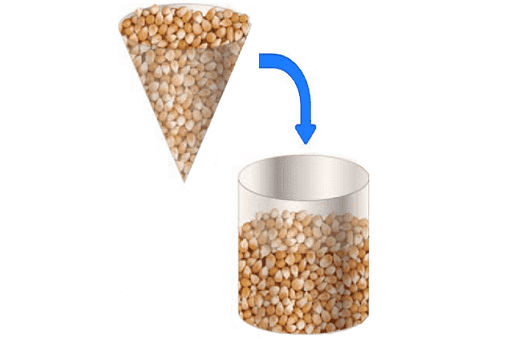
She repeats this until the cylinder is full.

Alexa finds that it takes 3 cones to fill the volume of the cylinder.

Step 1 :

Write the formula for volume V of a cylinder with base area B and height h.

V = B · h

Step 2 :

Find the base area B of the cone. 

We know that the base of the cone is a circle (Look at the figure given below).  

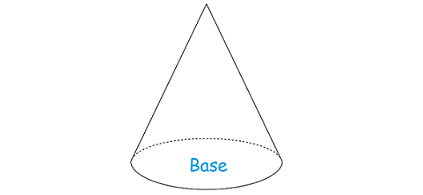
So, the area of the base of a cone is 

B = πr²

Step 3 :

Alexa found that, when the bases and height are the same,

3 x Volume of cone = Volume of cylinder

Step 4 :

From step 3, solve for volume of cone. 

3 x Volume of cone = Volume of cylinder

Divide both sides by 3. 

(3 x Volume of cone) ÷ 3 = Volume of cylinder ÷ 3

Volume of cone = 1/3 · Volume of cylinder

(Here, base radius and height of the cone and base radius and height of the cylinder are equal)

The formula to find the volume of a cone is given by

V = 1/3 · πr²h cubic units

Reflect

1. Use the conclusion from this experiment to write a formula for the volume of a cone in terms of the height and the radius. Explain.

Volume of the cone = 1/3 · Volume of cylinder

Substitute the formula for volume of cylinder.

Volume of the cone = 1/3 · πr²h

2. How do you think the formula for the volume of a cone is similar to the formula for the volume of a pyramid ?

Both are one third the area of the base times the height.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.