MULTIPLICATION OF TWO LOGARITHMS

Before learning multiplication of two logarithms, we have to be aware of the parts of a logarithm.

Consider the logarithm given below.

log_ba

In the logarithm above, 'a' is called argument and 'b' is called base.

You can multiply two logarithms, if one of the following two conditions is met.

Condition (i) :

The argument of the first logarithm and the base of the second logarithm must be same.

Condition (ii) :

The base of the first logarithm and the argument of the second logarithm must be same.

Let us see how the multiplication of two logarithms can be simplified in the following examples.

Example 1 :

Simplify : log_ab ⋅ log_bc

In the above two logarithms, the argument of the first logarithm and the base of the second logarithm are same. 

So, we can simplify the multiplication of above two logarithms by removing the part circled in red color. 

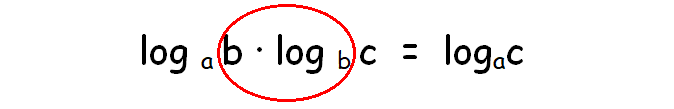
Example 2 :

Simplify : log_xy ⋅ log_zx

In the above two logarithms, the base of the first logarithm and the argument of the second logarithm are same.

So, we can simplify the multiplication of above two logarithms by removing the part marked in red color.

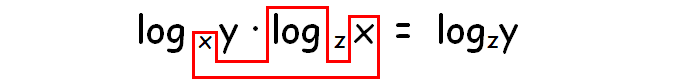
Still don't understand what is explained above, please watch the video below for step by step live explanation.

Solved Problems

Problem 1 :

Solve for x :

log_yx = 2/log₁₀y 

Solution :

log_yx = 2/log₁₀y

Multiply both sides by log₁₀y.

log_yx ⋅ log₁₀y = 2

Here, the base of the first logarithm and argument of the second logarithm are same.

Then,

log₁₀x = 2

The above equation is in logarithmic form. Convert it to exponential form to solve for x.

x = 10²

x = 100

Problem 2 :

If a = log₂₄12, b = log₃₆24 and c = log₄₈36, then find the value of (1 + abc) in terms of b and c.

Solution :

1 + abc = 1 + log₂₄12 ⋅ log₃₆24 ⋅ log₄₈36

= 1 + log₃₆12 ⋅ log₄₈36

= 1 + log₄₈12

= log₄₈48 + log₄₈12

= log₄₈(48 ⋅ 12)

= log₄₈(2 ⋅ 12)²

= 2log₄₈24

= 2log₃₆24 ⋅ log₄₈36

= 2bc

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.