MULTIPLYING INTEGERS

The product of two integers with opposite signs is negative. The product of two integers with the same sign is positive. 

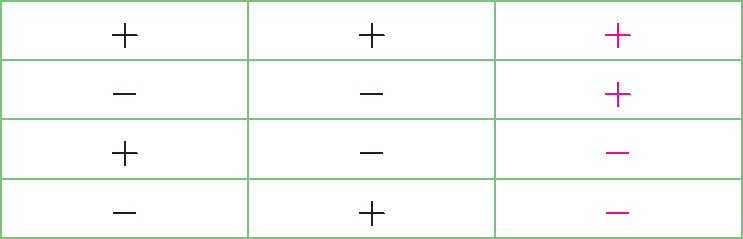
The product of 0 and any other integer is 0.

We can use the Multiplication property of 0 and the Distributive Property to show that a negative number times a negative number is always a positive number.

Show that (-1)(-1)  =  1.

0  =  -1(0) ----> Multiplication property of 0

0  =  -1(-1 + 1) ----> Addition property of opposites

0  =  (-1)(-1) + (-1)(1) ----> Distributive Property

0  =  (-1)(-1) + (-1) ----> Multiplication property of 1

So,

(-1)(-1)  =  1 ----> Definition of opposites

Practice Questions

Question 1 : 

Multiply :

(13)(-3)

Answer : 

Step 1 : 

Determine the sign of the product.

13 is positive and -3 is negative. Since the numbers have opposite signs, the product will be negative.

Step 2 :

Find the absolute values of the numbers and multiply them.

|13|  =  13 and |-3|  =  3

13 x 3  =  39

Step 3 : 

Assign the correct sign to the product.

13(-3)  =  -39

So, the product is -39.

Question 2 : 

Multiply :

(-5)(-8)

Answer : 

Step 1 : 

Determine the sign of the product.

-5 is negative and -8 is negative. Since the numbers have the same sign, the product will be positive.

Step 2 :

Find the absolute values of the numbers and multiply them.

|-5|  =  5 and |-8|  =  8

5 x 8  =  40

Step 3 : 

Assign the correct sign to the product.

(-5)(-8)  =  40

So, the product is 40.

Question 3 : 

Multiply :

(-3)(8)

Answer : 

Step 1 : 

Determine the sign of the product.

-3 is negative and 8 is positive. Since the numbers have opposite signs, the product will be negative.

Step 2 :

Find the absolute values of the numbers and multiply them.

|-3|  =  3 and |8|  =  8

3 x 8  =  24

Step 3 : 

Assign the correct sign to the product.

(-3)(8)  =  -24

So, the product is -24.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.